Solve x2−x−2>0.
A
x∈(−∞,−1)∪(2,∞)
B
x∈(∞,2)
C
x∉(−∞,−1)∪(2,∞)
D
x∉(∞,2)
Video Solution
Text Solution
The correct Answer is:A
x2−x−2>0.
or (x−2)(x+1)>0
Now x2−x−2=0⇒x=−1,2
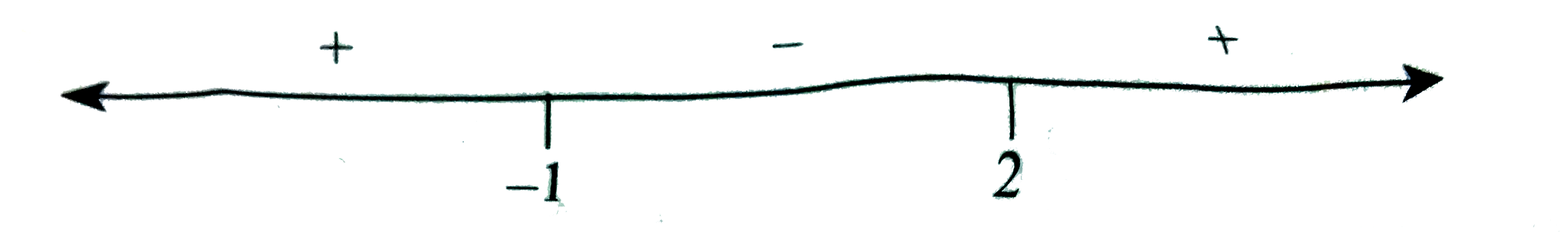
On the number lilne (x- axis) mark x= -1 and x =2
Now when x>2,x+1>0 and x−2>0
⇒(x+1)(x−2)<0
When −1<x<2,x+1>0 but x−2<0
⇒(x+1)(x−2)<0
When x>−1,x+1<0andx−2<0
⇒(x+1)(x−2)>0
Hence sing scheme of x2−x−2is
From the figure x2−x−2>0,x∈(−∞,−1)∪(2.∞)
|
Topper's Solved these Questions
- SET THEORY AND REAL NUMBER SYSTEM BOOK - CENGAGECHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Exercise 1.1
12 Videos
- SET THEORY AND REAL NUMBER SYSTEM BOOK - CENGAGECHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Exercise 1.2
8 Videos
- SEQUENCE AND SERIESBOOK - CENGAGECHAPTER - SEQUENCE AND SERIESEXERCISE - Question Bank
36 Videos
- SETS AND RELATIONSBOOK - CENGAGECHAPTER - SETS AND RELATIONSEXERCISE - Question Bank
15 Videos
Similar Questions
Knowledge Check
Solve for x(x>0)
log3x(3x)+log23x=1Ax = 1 and there are infinitely more solutions .Bx = 1 , x2=13 only two solutionsCx = 1 , x2=3 only two solutionsDx = 1 , x2=3,x3=13 only three solutions
