State true or False: ∑p1q1p0q1×100 is Paasche’s Price Index Number.
The correct Answer is:False
To determine whether the statement "sum(p_1q_1)/(p_0q_1)xx100 is Paasche’s Price Index Number" is true or false, we can analyze the formula and compare it with the correct definition of Paasche's Price Index Number.
1. Understand the Components:
- Let P0 be the price in the base year.
- Let P1 be the price in the current year.
- Let Q1 be the quantity in the current year.
2. Define Paasche’s Price Index:
- Paasche’s Price Index is defined as:
P=∑(P1Q1)∑(P0Q1)×100
- Here, the numerator is the total value of current year prices multiplied by current year quantities, and the denominator is the total value of base year prices multiplied by current year quantities.
3. Analyze the Given Expression:
- The expression given in the question is:
sum(p1q1)(p0q1)×100
- In this expression, the denominator is not summing over the quantities, which is a crucial part of the Paasche's Price Index formula.
4. Conclusion:
- Since the expression does not match the definition of Paasche’s Price Index (specifically, it lacks the summation in the denominator), we can conclude that the statement is False.
Final Answer:
False: The expression given is not Paasche’s Price Index Number.
- Let P0 be the price in the base year.
- Let P1 be the price in the current year.
- Let Q1 be the quantity in the current year.
- Paasche’s Price Index is defined as:
P=∑(P1Q1)∑(P0Q1)×100
- Here, the numerator is the total value of current year prices multiplied by current year quantities, and the denominator is the total value of base year prices multiplied by current year quantities.
- The expression given in the question is:
sum(p1q1)(p0q1)×100
- In this expression, the denominator is not summing over the quantities, which is a crucial part of the Paasche's Price Index formula.
- Since the expression does not match the definition of Paasche’s Price Index (specifically, it lacks the summation in the denominator), we can conclude that the statement is False.
False: The expression given is not Paasche’s Price Index Number.
Topper's Solved these Questions
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 5. INDEX NUMBERS (IV) Solve the following problems.)
21 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 5. INDEX NUMBERS (V Activity)
1 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 5. INDEX NUMBERS (II Fill in the blanks.)
8 Videos
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
Similar Questions
Knowledge Check
∑p1q1w∑p0q0w×100 gives
AValue Index Number by Simple Aggregate methodBValue Index Number by Weighted Aggregate methodCCost of Living Index NumberDLaspeyre’s Index NumberThe formula p01=∑p1q0∑p0q0×100 is for
ALaspeyre’s Price Index NumberBPaasche’s Price Index NumberCFisher’s Price Index NumberDWalsh’s Price Index NumberP01=∑p1q0∑p0q0×100 is the formula of:
ALaspeyre's MethodBPaasche's MethodCFisher's MethodDnone of these
State True or False: ∑p0√q0+q1∑p1√q0+q1 is Marshall-Edgeworth Price Index Number.
View SolutionState True or False: Walsh’s Price Index Number is given by
∑p1√q0q1∑p0√q0q1×100
View SolutionThe average of Laspeyre’s and Paasche’s Price Index Numbers is called……………..Price Index Number.
View SolutionFind the missing price if Laspeyre’s and Paasche’s Price Index Numbers are equal for following data.
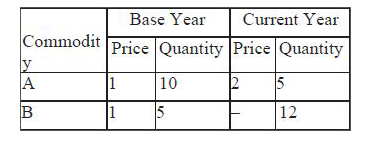
View SolutionState whether the statements given are True or False.
If pq is a rational number and m is a non-zero integer, then p×mq×m is a rational number not equivalent to pq.
View Solution
