State whether the given statement is True or False: The order of rotational symmetry of a figure is 4 and the angle of rotation is only.
To determine whether the statement "The order of rotational symmetry of a figure is 4 and the angle of rotation is 180 degrees only" is true or false, we can follow these steps:
1. Understand Rotational Symmetry:
- Rotational symmetry of a figure means that the figure can be rotated around a central point and still look the same at certain angles.
- The order of rotational symmetry is the number of times a figure looks the same during a full rotation of 360 degrees.
2. Identify the Given Information:
- The statement claims that the order of rotational symmetry is 4, and the angle of rotation is 180 degrees.
3. Calculate the Angle of Rotation for Order 4:
- If the order of rotational symmetry is 4, then the angle of rotation can be calculated as:
Angle of rotation=360∘Order of rotational symmetry=360∘4=90∘
- This means that for a figure with an order of rotational symmetry of 4, it will look the same every 90 degrees.
4. Evaluate the Given Statement:
- The statement claims that the angle of rotation is 180 degrees. However, we have calculated that for an order of 4, the angle of rotation should be 90 degrees.
- Therefore, the angle of rotation being 180 degrees contradicts the order of rotational symmetry being 4.
5. Conclusion:
- Since the angle of rotation does not match the order of rotational symmetry stated in the question, the statement is False.
Final Answer:
The statement is False.
- Rotational symmetry of a figure means that the figure can be rotated around a central point and still look the same at certain angles.
- The order of rotational symmetry is the number of times a figure looks the same during a full rotation of 360 degrees.
- The statement claims that the order of rotational symmetry is 4, and the angle of rotation is 180 degrees.
- If the order of rotational symmetry is 4, then the angle of rotation can be calculated as:
Angle of rotation=360∘Order of rotational symmetry=360∘4=90∘
- This means that for a figure with an order of rotational symmetry of 4, it will look the same every 90 degrees.
- The statement claims that the angle of rotation is 180 degrees. However, we have calculated that for an order of 4, the angle of rotation should be 90 degrees.
- Therefore, the angle of rotation being 180 degrees contradicts the order of rotational symmetry being 4.
- Since the angle of rotation does not match the order of rotational symmetry stated in the question, the statement is False.
The statement is False.
Topper's Solved these Questions
- PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESBOOK - NCERT EXEMPLARCHAPTER - PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESEXERCISE - THINK AND DISCUSS
6 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Think and Discuss
7 Videos
- RATIONAL NUMBERSBOOK - NCERT EXEMPLARCHAPTER - RATIONAL NUMBERSEXERCISE - Application
2 Videos
Similar Questions
Knowledge Check
The order of rotational symmetry of a circle is
A0B1C2DinfiniteThe order of rotational symmetry of a rectangle is.
A1B4C2D0The order of rotational symmetry of regular octagon is
A7B8C10D6
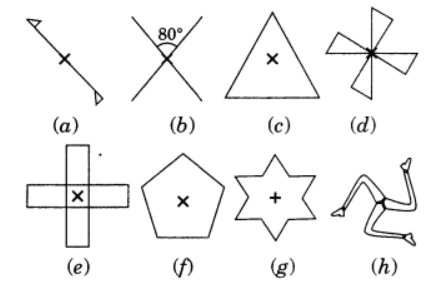
Give the order of rotational symmetry for each figure:
View SolutionThe order of rotational symmetry in the figure given below is

View SolutionOrder of rotational symmetry of a semi circle is two.
View SolutionThe order of rotational symmetry in the fig given below is

View SolutionThe order of rotational symmetry in the given figure is:

View Solution