State whether the statement given is true (T)
or false (F):
A four-digit number abcd is divisible by 4 if ab is divisible by 4
To determine whether the statement "A four-digit number abcd is divisible by 4 if ab is divisible by 4" is true or false, we can follow these steps:
Step 1: Understand the Rule of Divisibility by 4
The rule for a number to be divisible by 4 is that the last two digits of the number must be divisible by 4. For a four-digit number represented as abcd, the last two digits are cd.
Hint: Remember that for divisibility by 4, we only need to check the last two digits of the number.
Step 2: Analyze the Given Statement
The statement claims that if the first two digits (ab) of the four-digit number are divisible by 4, then the entire number abcd is also divisible by 4. However, this is not necessarily true.
Hint: Think about examples where ab is divisible by 4, but cd might not be.
Step 3: Provide a Counterexample
Let's consider an example:
- Take the number 1204. Here, ab = 12, which is divisible by 4.
- Now check cd = 04, which is also divisible by 4. In this case, the statement holds true.
Now consider another example:
- Take the number 1205. Here, ab = 12, which is divisible by 4.
- Now check cd = 05, which is not divisible by 4. In this case, the statement does not hold true.
Hint: Use specific numbers to test the validity of the statement.
Step 4: Conclusion
Since we found a case where ab is divisible by 4 but cd is not, we conclude that the statement is false. Therefore, the statement "A four-digit number abcd is divisible by 4 if ab is divisible by 4" is false.
Final Answer: The statement is False (F).
The rule for a number to be divisible by 4 is that the last two digits of the number must be divisible by 4. For a four-digit number represented as abcd, the last two digits are cd.
The statement claims that if the first two digits (ab) of the four-digit number are divisible by 4, then the entire number abcd is also divisible by 4. However, this is not necessarily true.
Let's consider an example:
- Take the number 1204. Here, ab = 12, which is divisible by 4.
- Now check cd = 04, which is also divisible by 4. In this case, the statement holds true.
- Take the number 1205. Here, ab = 12, which is divisible by 4.
- Now check cd = 05, which is not divisible by 4. In this case, the statement does not hold true.
Since we found a case where ab is divisible by 4 but cd is not, we conclude that the statement is false. Therefore, the statement "A four-digit number abcd is divisible by 4 if ab is divisible by 4" is false.
Topper's Solved these Questions
- PLAYING WITH NUMBERS BOOK - NCERT EXEMPLARCHAPTER - PLAYING WITH NUMBERS EXERCISE - Exercise
28 Videos
- PLAYING WITH NUMBERS BOOK - NCERT EXEMPLARCHAPTER - PLAYING WITH NUMBERS EXERCISE - Application, Games and Puzzles
1 Videos
- PLAYING WITH NUMBERS BOOK - NCERT EXEMPLARCHAPTER - PLAYING WITH NUMBERS EXERCISE - Exercise (Fill in the blanks)
16 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - THINK AND DISCUSS
2 Videos
- RATIONAL NUMBERS BOOK - NCERT EXEMPLARCHAPTER - RATIONAL NUMBERS EXERCISE - Think and Discuss
1 Videos
Similar Questions
State whether the statement given is true (T) or false (F):
A three-digit number abc is divisible by 5 if c is an even number.
View SolutionState whether the statement given is true (T) or false (F):
A three-digit number abc is divisible by 6 if c is an even number and a + b + c is a multiple of 3.
View SolutionState whether the statement given is true (T) or false (F):
A two-digit number ab is always divisible by 2 if b is an even number.
View SolutionState whether the statement given is true (T) or false (F):
If a number a is divisible by b, then it must be divisible by each factor of b.
View Solutionstate whether the statement are true (T) or false (F)
If a number is divisible by 2 and 4, then it will be divisible by 8.
View Solutionstate whether the statement are true (T) or false (F)
A three-digit number 42x is divisible by 9. Find the value of x.
View SolutionState whether the statement given is true (T) or false (F):
If 213x 27 is divisible by 9, then the value of x is 0.
View Solutionstate whether the statement are true (T) or false (F)
Let ab be a two-digit number, then ab + ba is divisible by 9.
View SolutionState whether the statements are true (T) or (F) false.
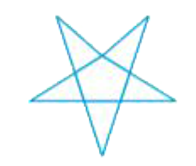 is a polygon.
is a polygon.
View Solutionstate whether the statement are true (T) or false (F).
 is a concave polygon.
is a concave polygon.
View Solution
