State which of the following are not the probability distributions of a random variable. Give reasons for your answer.
The correct Answer is:N/a
We know that the sum of all probabilities of a probality distribution is 1.
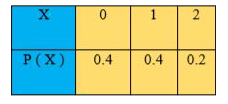
(i) Sum of probability =0.4+0.4+0.2=1
Therefore given table is the probability distribution of a random variable.
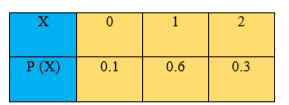
(ii) It can be seend that for X=3,P(X)=−0.1
The probability given table is not the probability distribution of a random variable.
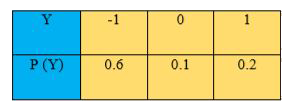
(iii) Here all probabilities are positive but their sum is less than 1. Therefore given distribution is not a probability distribution.
(0.6+0.1+0.2=0.9<1)
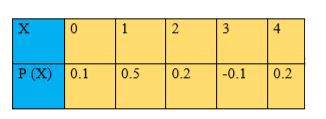
(iv) Here all probabilities are positive but their sum is more than 1.
(0.3+0.2+0.4+0.1+0.05=1.05>1) ltrbgt Therefore given distribution is ot a probability distribution.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - PROBABIILITYEXERCISE - Exercise 13.515videos
PROBABIILITY
- BOOK - NAGEEN PRAKASHANCHAPTER - PROBABIILITYEXERCISE - Miscellaneous Exercise19videos
PROBABIILITY
- BOOK - NAGEEN PRAKASHANCHAPTER - PROBABIILITYEXERCISE - Exercise 13.314videos
PROBABIILITY
- BOOK - NAGEEN PRAKASHANCHAPTER - MATRICESEXERCISE - Miscellaneous Exerice15videos
MATRICES
- BOOK - NAGEEN PRAKASHANCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Miscellaneous Exercise19videos
RELATIONS AND FUNCTIONS
Similar Questions
State which of the following are not the probability distributions of a random variable. Given reasons for your answer.
 View Solution
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
 View Solution
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
 View Solution
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
 View Solution
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
 View Solution
View SolutionState whether the following is not the probability mass function of random variable. Give reasons for your answer.
 View Solution
View SolutionWhich of the following probability distribution does not exist for randam variable? Give reason?
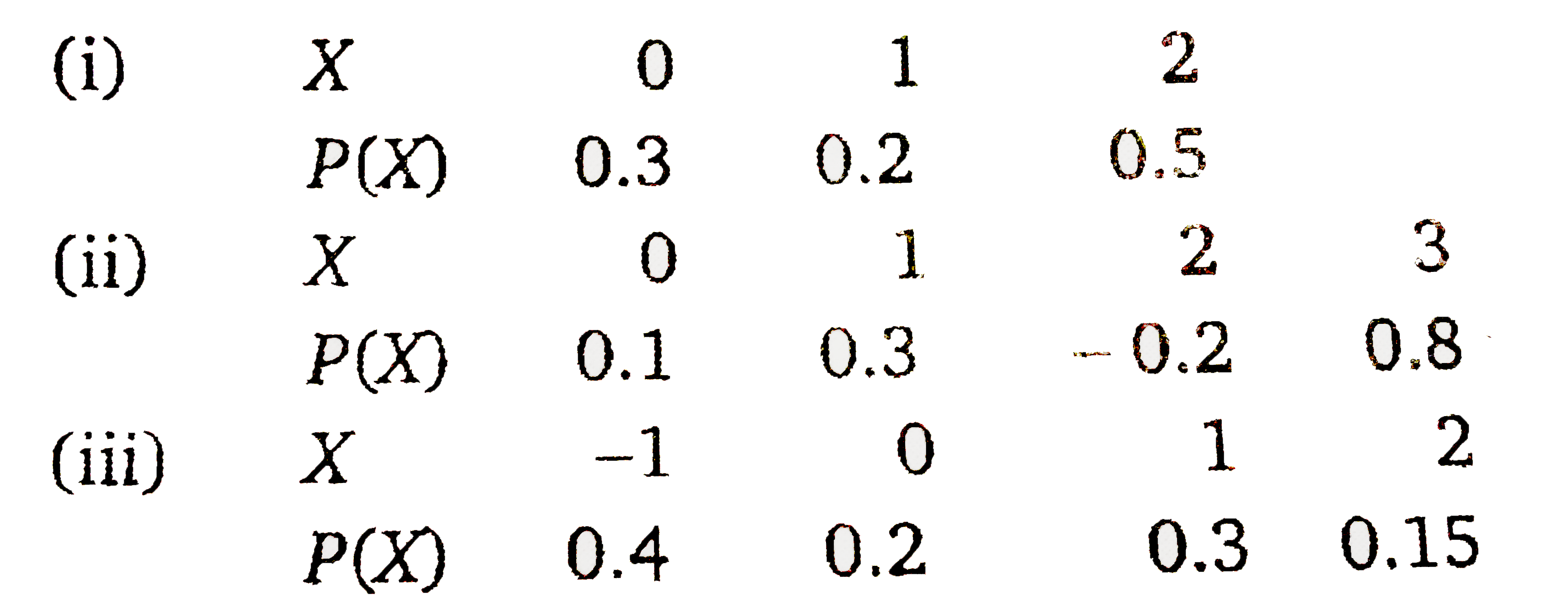 View Solution
View SolutionThe following is the probability distribution of a random vaiable X.
The value of k is
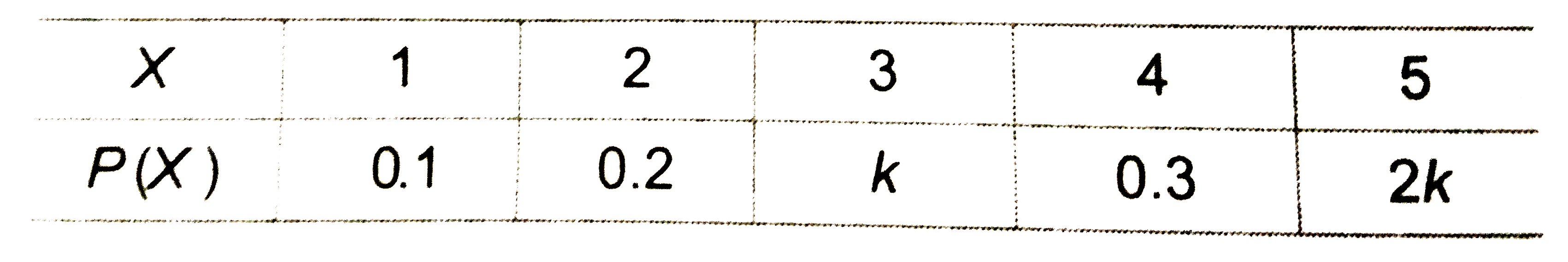 View Solution
View SolutionConsider the probability distribution of a random variable X.

Variance of XView SolutionThe probability distribution of a random variable is as follows: The variance of x is
View Solution

