State, with reason which of the are surds and which are not :
√180
The correct Answer is:√1806√5
To determine whether √180 is a surd or not, we will simplify it and analyze the result.
1. Factorization of 180:
We start by factorizing 180 into its prime factors.
180=18×10
Now, we can further factor 18 and 10:
18=2×9=2×32
10=2×5
So, combining these, we have:
180=2×32×2×5=22×32×5
2. Simplifying the Square Root:
Now we can simplify √180 using the prime factorization:
√180=√22×32×5
We can take the square root of the perfect squares out of the radical:
√180=√22×√32×√5=2×3×√5
This simplifies to:
√180=6√5
3. Conclusion:
Since √5 is not a perfect square and cannot be simplified further, √180 remains in the form 6√5. Therefore, √180 is a surd.
Final Answer:
√180 is a surd because it cannot be simplified to a rational number.
We start by factorizing 180 into its prime factors.
180=18×10
Now, we can further factor 18 and 10:
18=2×9=2×32
10=2×5
So, combining these, we have:
180=2×32×2×5=22×32×5
Now we can simplify √180 using the prime factorization:
√180=√22×32×5
We can take the square root of the perfect squares out of the radical:
√180=√22×√32×√5=2×3×√5
This simplifies to:
√180=6√5
Since √5 is not a perfect square and cannot be simplified further, √180 remains in the form 6√5. Therefore, √180 is a surd.
√180 is a surd because it cannot be simplified to a rational number.
Topper's Solved these Questions
- RATIONAL AND IRRATIONAL NUMBERS BOOK - ICSECHAPTER - RATIONAL AND IRRATIONAL NUMBERS EXERCISE - EXERCISE 1 (D)
21 Videos
- RATIONAL AND IRRATIONAL NUMBERS BOOK - ICSECHAPTER - RATIONAL AND IRRATIONAL NUMBERS EXERCISE - EXERCISE 1 (B)
43 Videos
- PYTHAGORAS THEOREMBOOK - ICSECHAPTER - PYTHAGORAS THEOREMEXERCISE - 4 MARKS QUESTIONS
9 Videos
- RECTILINEAR FIGURESBOOK - ICSECHAPTER - RECTILINEAR FIGURESEXERCISE - QUADRILATERALS AND ITS PROPERTIES - 4 MARKS QUESTIONS
7 Videos
Similar Questions
State, with reason which of the are surds and which are not :
√π
View SolutionState, with reason which of the are surds and which are not :
4√27
View SolutionState, with reason which of the are surds and which are not :
√3+√2
View SolutionState, with reason which of the are surds and which are not :
3√−125
View SolutionState, with reason, which of the following are surds and which are not :
(i) √180 (ii) 3√64 (iii) 3√25×3√40
View SolutionState with reasons which of the following is a surd which is not :
(i) √27 (ii) √225×√4
View SolutionState giving reason whether AB||CD
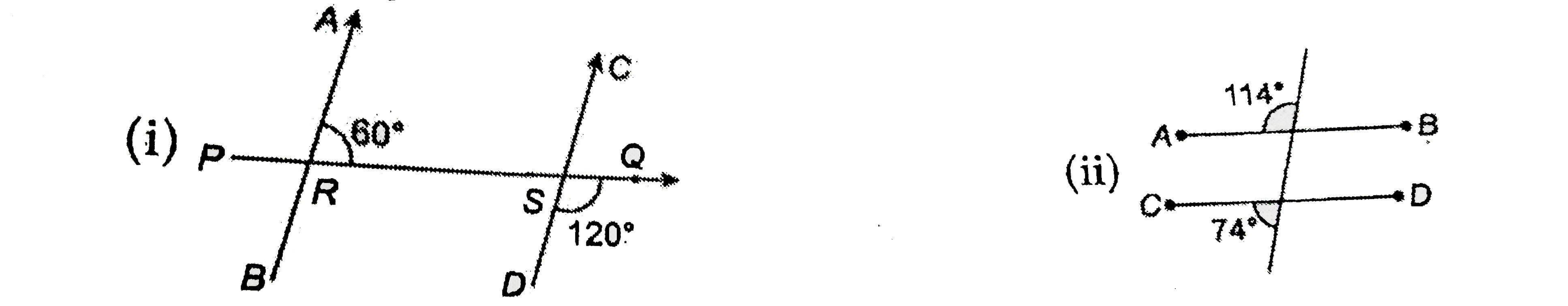
View SolutionState with reasons, which of the following pairs of terms are of like terms and which are of unlike terms : (i) 7x,12y (ii) 15x−21x (iii) 4ab,7ba
View SolutionState, which of the following variable are continuous and which are discrete:
marks scored in a test.
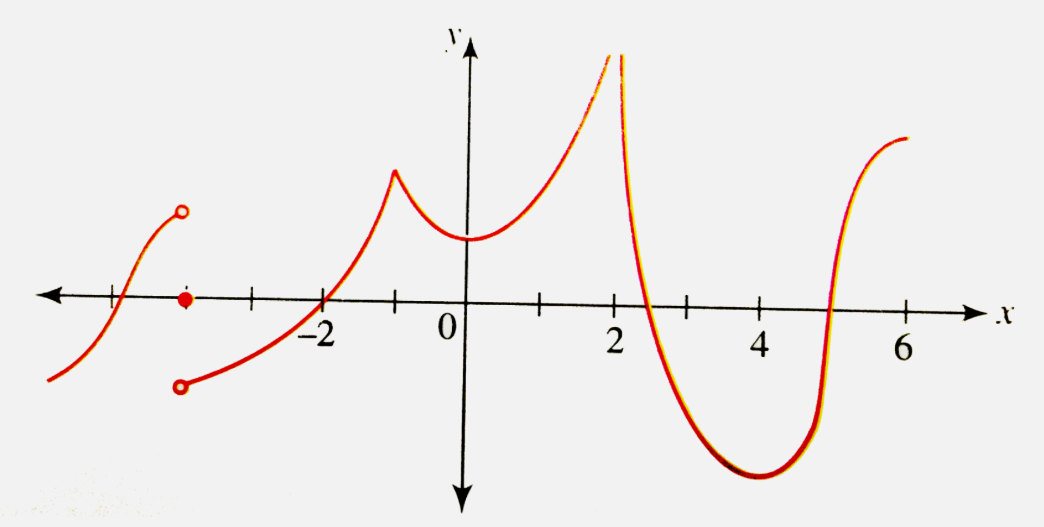
View SolutionThe graph of f is shown. State, with reason, the numbers at which f is not differentiable.
View Solution
