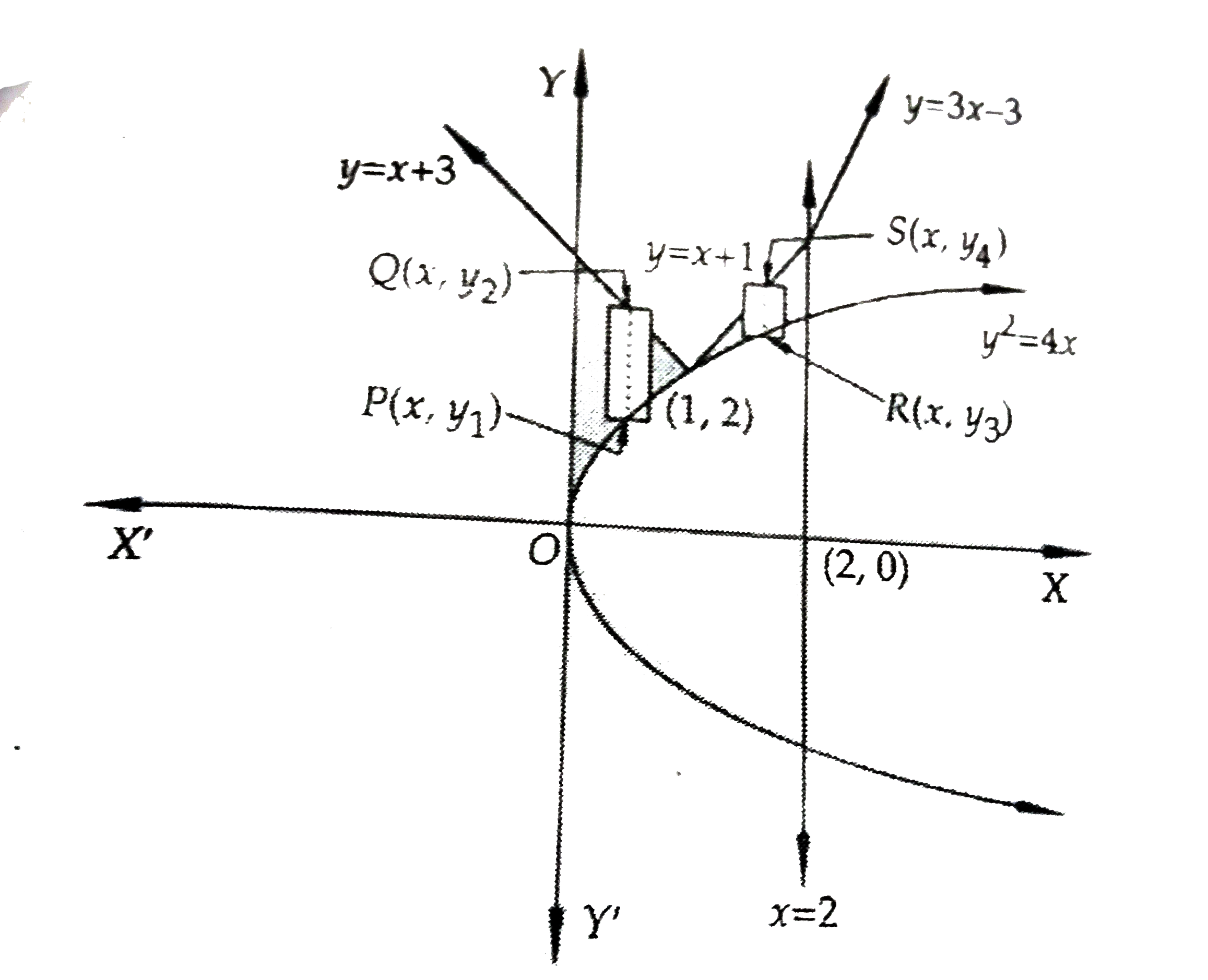
Suppose that denotes the area of the region bounded by and , where . Then the value of when is (A) 4 (B) 5 (C) 6 (D) 9
A
5
B
6
C
7
D
9
Video Solution
Text Solution
The correct Answer is:a
When F(1) is the area of the shaded region bounded by
or,
as shown in Fig. 31
|
Topper's Solved these Questions
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
62 Videos
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- APPLICATION OF DERIVATIVESBOOK - OBJECTIVE RD SHARMACHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Illustration
6 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos
Similar Questions
Knowledge Check
Let f(x)=αxx+1,x≠−1. Then, for what values of α is f[f(x)]=x?
A√2B−√2C1D-1The equation of the circle which touches the axes at a distances 5 from the origin is y2+x2−2αx−2αy+α2=0
What is the value of α ?A4B5C6D7If α,β are the roots of the equation x2+αx+β=0 such that α≠β and ||x−β|−α|∣≤α, then
Ainequality is satisfied by exactly two integral values of xBinequality is satisfied by all values of x∈(−4,−2)CRoots of the equation are opposite in signDx2+αx+β<0∀x∈[−1,0]
If y=(1−x)−αe−αx,x≠1 then
View Solutionif two curves y=2sin(5π6)x and y=αx2−3ax+2α+1 touch each other at some point then the value of √3α5π is (0≤x≤185)
View SolutionIf f(x) is a non - negative function such that the area bounded by y=f(x), x - axis and the lines x = 0 and x=α is 4αsinα+2 sq. Units
(∀α∈[0,π]), then the value of f(π2) is equal to
View SolutionGiven that α1,α2,α3 are the roots of 3x3−x2−10x+8=0 then the value of α21+α22+α23
View SolutionLet f(x)=αx2−a+1x"where"alphaisrealconstant.Thesmal≤stalphaforf(x)ge0"for all"xgt0 is-
View Solution
