Tangents are drawn to the ellipse x2a2+y2b2=1,(a>b), and the circle x2+y2=a2 at the points where a common ordinate cuts them (on the same side of the x-axis). Then the greatest acute angle between these tangents is given by tan−1(a−b2√ab) (b) tan−1(a+b2√ab) tan−1(2ab√a−b) (d) tan−1(2ab√a+b)
tan−1(a−b2√ab)
tan−1(a+b2√ab)
tan−1(2ab2√a−b)
tan−1(2ab2√a+b)
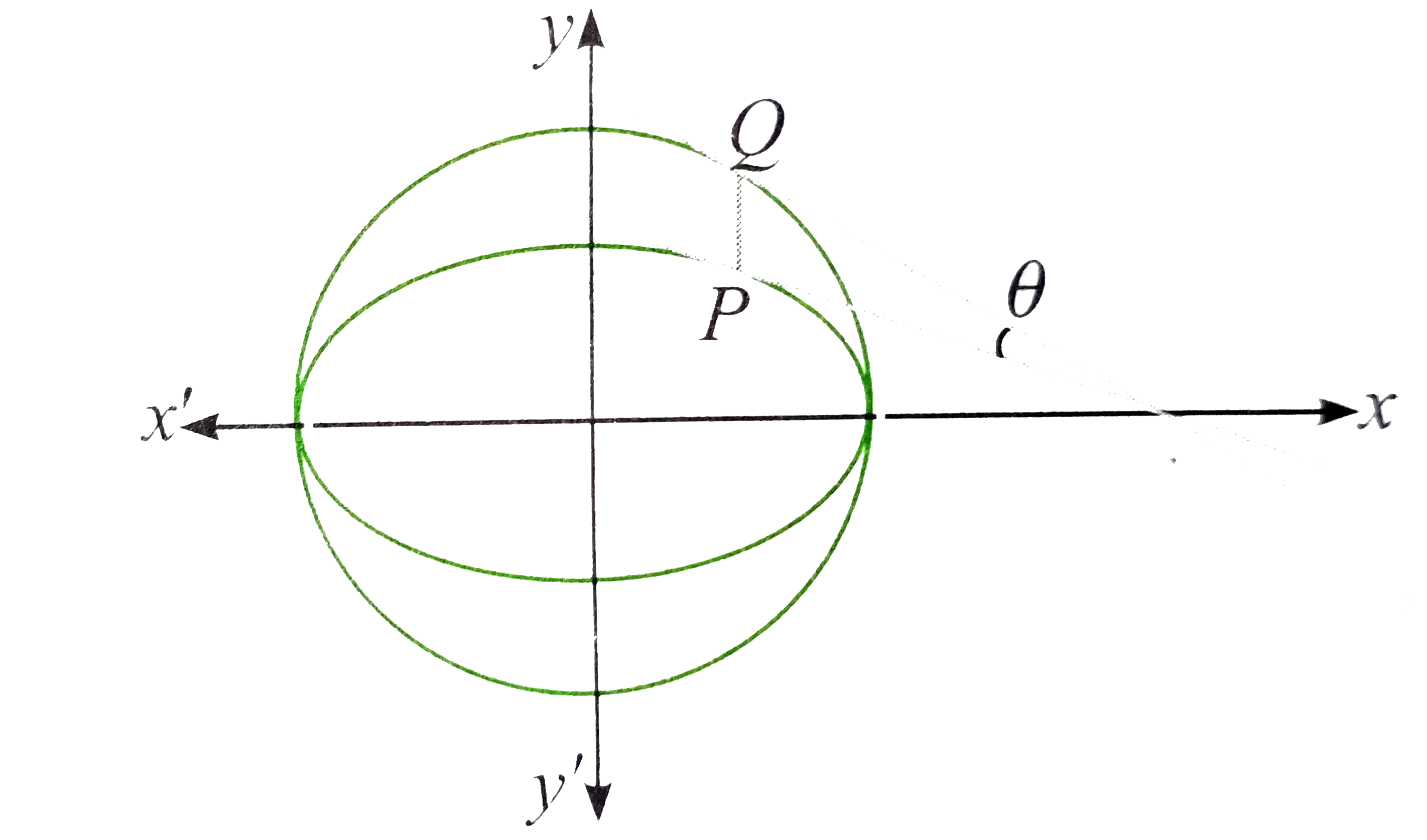
Tanent to the ellipse at P(acosα,bsinα) is xacosα+ybisnα=1 (1)
Tangent to the circle at Q(acos,α,asinα) is cosax+sinαy=a (2)
Now, the angle between the tangents is θ. Then, tanθ=∣∣
∣
∣∣−bacotα−(−cotα)1+(−bacotα)(−cotα)∣∣
∣
∣∣
=∣∣
∣
∣∣cosα(1−ba)1+bacot2α∣∣
∣
∣∣=∣∣∣a−batanα+bcotα∣∣∣
=∣∣
∣
∣∣a−b(√atanα−√bcotα)2+2√ab∣∣
∣
∣∣
Now, the greatest value of the above expression is ∣∣∣a−b2√ab∣∣∣ when √tanα=√btanα. Therefore,
θmaximum=tan−1(a−b2√ab)
Similar Questions
Tangents are drawn to the ellipse x2a2+y2b2=1,(a>b), and the circle x2+y2=a2 at the points where a common ordinate cuts them (on the same side of the x-axis). Then the greatest acute angle between these tangents is given by (A) tan−1(a−b2√ab) (B) tan−1(a+b2√ab) (C) tan−1(2ab√a−b) (D) tan−1(2ab√a+b)
View Solutiona(√a+√b2b√a)−1+ b(√a+√b2a√b)−1
View Solution
Knowledge Check
If xa+yb=√2 touches the ellipse x2a2+y2b2=1 at a point P, then eccentric angle of P is
A0B45∘C60∘D90∘
∫1a2−b2cos2xdx(a>b)=1a√a2−b2tan−1[atanx√a2−b2]+c
View Solutiony=tan−1[√a−ba+btanx2]
View SolutionIf 3tan−1(12+√3)−tan−11x=tan−113, then x is equal to 1 (b) 2 (c) 3 (d) √2
View SolutionProve that 2tan−1√ba=cos−1(a−ba+b)
View SolutionProve that 2tan−1(√a−ba+btan(θ2))=cos−1(acosθ+ba+bcosθ)
View SolutionProve that: tan[π4+12tan−1(ab)]+tan[π4−12tan−1(ab)]=2√a2+b2b
View SolutionIf y=√(a−x)(x−b)−(a−b)tan−1(√a−x√x−b) then dydx=
View Solution
