The angle of elevation of the bottom of a window 10 m above the ground level from a point on the ground is 30∘ A pole projecting outwards from the bottom of the window makes an angle of 30∘ with the wall. If the angle of elevation of the top of the pole observed from the same point on the ground is 60∘, find the length of the pole to the nearest whole number.
To solve the problem step by step, we will first visualize the scenario and then apply trigonometric principles to find the length of the pole.
Step 1: Draw the Diagram
- Let's denote the point on the ground as A.
- The bottom of the window, which is 10 m above the ground, is point B.
- The top of the pole is point D, and the point where the pole meets the wall is point C.
- The angle of elevation from point A to point B is 30∘.
- The pole CD makes an angle of 30∘ with the wall.
Step 2: Identify the Lengths
- Let AD be the length of the pole, which we need to find, and denote it as x.
- Let AC be the distance from point A to point C (the base of the pole), and denote it as y.
Step 3: Use Triangle ABC
In triangle ABC:
- The angle ACB is 30∘.
- The angle CAB can be calculated using the property that the sum of angles in a triangle is 180∘:
∠CAB+30∘+90∘=180∘⟹∠CAB=60∘
Step 4: Calculate AC (y)
Using the sine function in triangle ABC:
sin(30∘)=ABAC⟹12=10y
Cross-multiplying gives:
y=20 m
Step 5: Use Triangle ACD
In triangle ACD:
- The angle DAE is 30∘.
- The angle DAC is 90∘ (since AC is vertical).
- The angle DCA is 30∘ (since DCA+ACB=DCB and DCB=60∘).
Using the tangent function:
tan(30∘)=ADAC⟹1√3=x20
Cross-multiplying gives:
x=20√3≈11.55 m
Step 6: Round to the Nearest Whole Number
The length of the pole AD is approximately 11.55 m, which rounds to 12 m.
Final Answer
The length of the pole is approximately 12 meters.
---
- Let's denote the point on the ground as A.
- The bottom of the window, which is 10 m above the ground, is point B.
- The top of the pole is point D, and the point where the pole meets the wall is point C.
- The angle of elevation from point A to point B is 30∘.
- The pole CD makes an angle of 30∘ with the wall.
- Let AD be the length of the pole, which we need to find, and denote it as x.
- Let AC be the distance from point A to point C (the base of the pole), and denote it as y.
In triangle ABC:
- The angle ACB is 30∘.
- The angle CAB can be calculated using the property that the sum of angles in a triangle is 180∘:
∠CAB+30∘+90∘=180∘⟹∠CAB=60∘
Using the sine function in triangle ABC:
sin(30∘)=ABAC⟹12=10y
Cross-multiplying gives:
y=20 m
In triangle ACD:
- The angle DAE is 30∘.
- The angle DAC is 90∘ (since AC is vertical).
- The angle DCA is 30∘ (since DCA+ACB=DCB and DCB=60∘).
tan(30∘)=ADAC⟹1√3=x20
Cross-multiplying gives:
x=20√3≈11.55 m
The length of the pole AD is approximately 11.55 m, which rounds to 12 m.
The length of the pole is approximately 12 meters.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Exercise32videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (short Answer Questions)5videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions5videos
REAL NUMBERS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
Similar Questions
The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground is 30∘ . What will be the angle of elevation, if the height of the tower is tripled?
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.
 View Solution
View Solution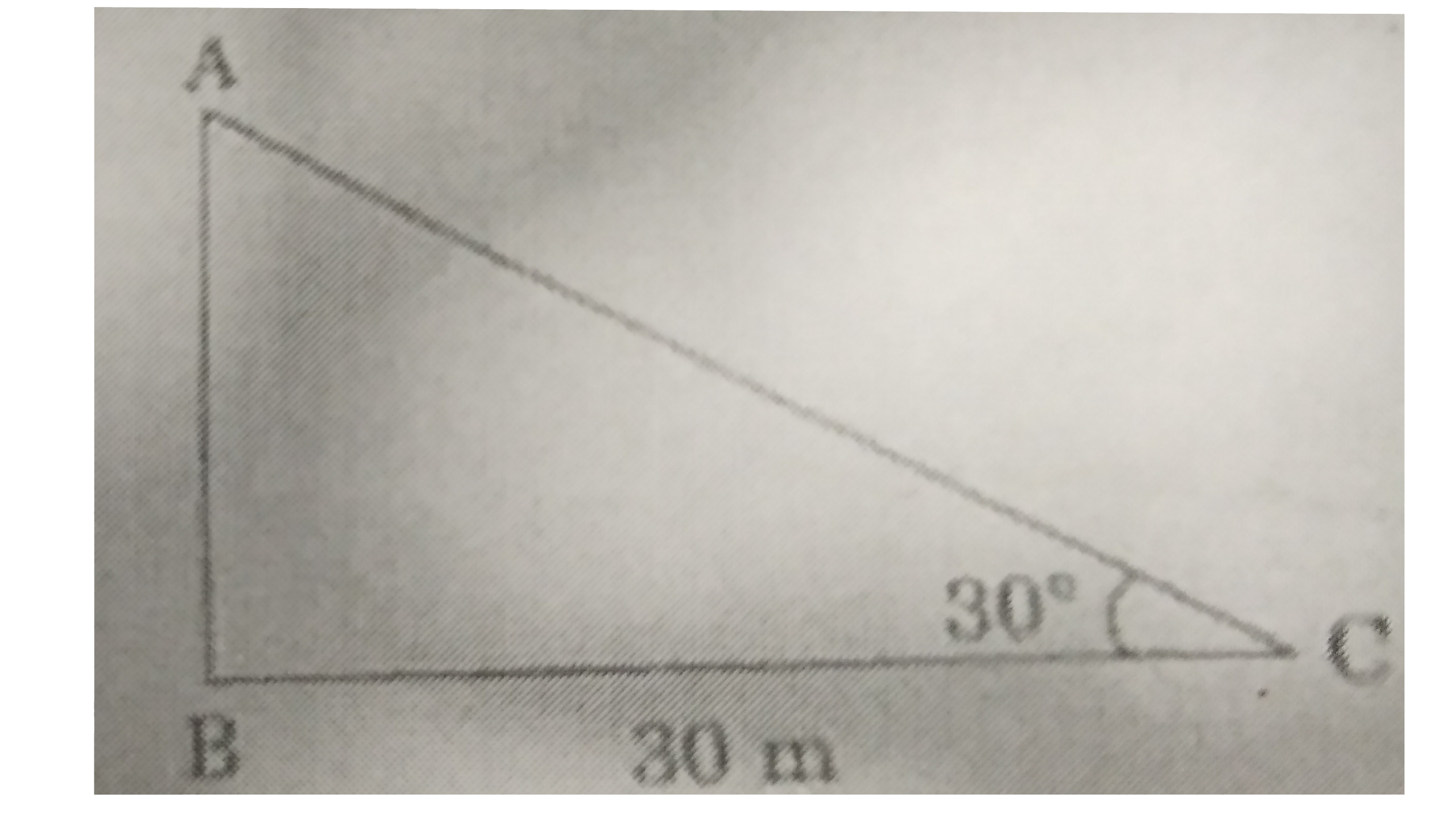
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
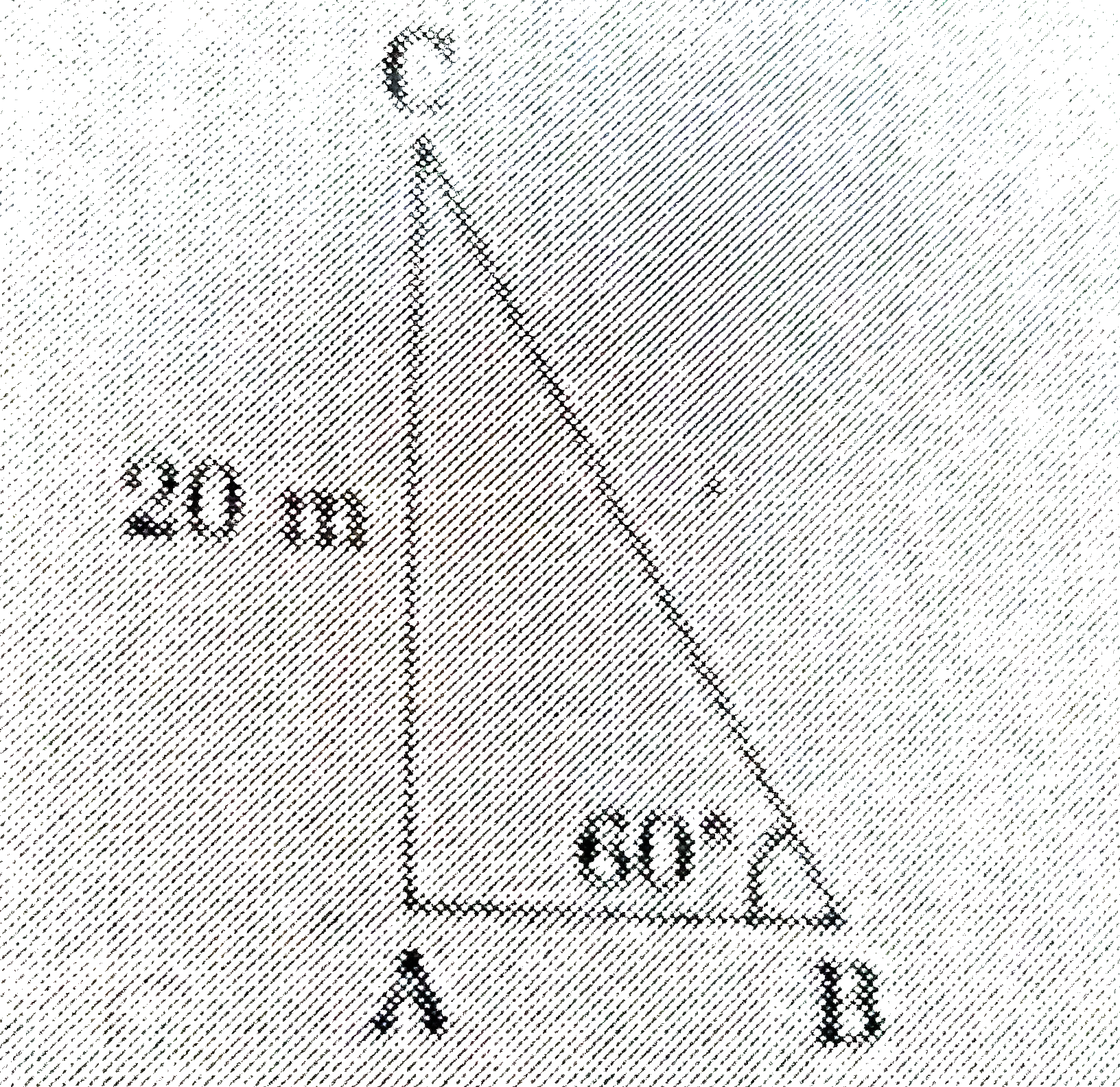 View Solution
View Solution
