The area bounded by and isSolution in Bengali
sq. units
sq. units
sq. units
None of these
The correct Answer is:C
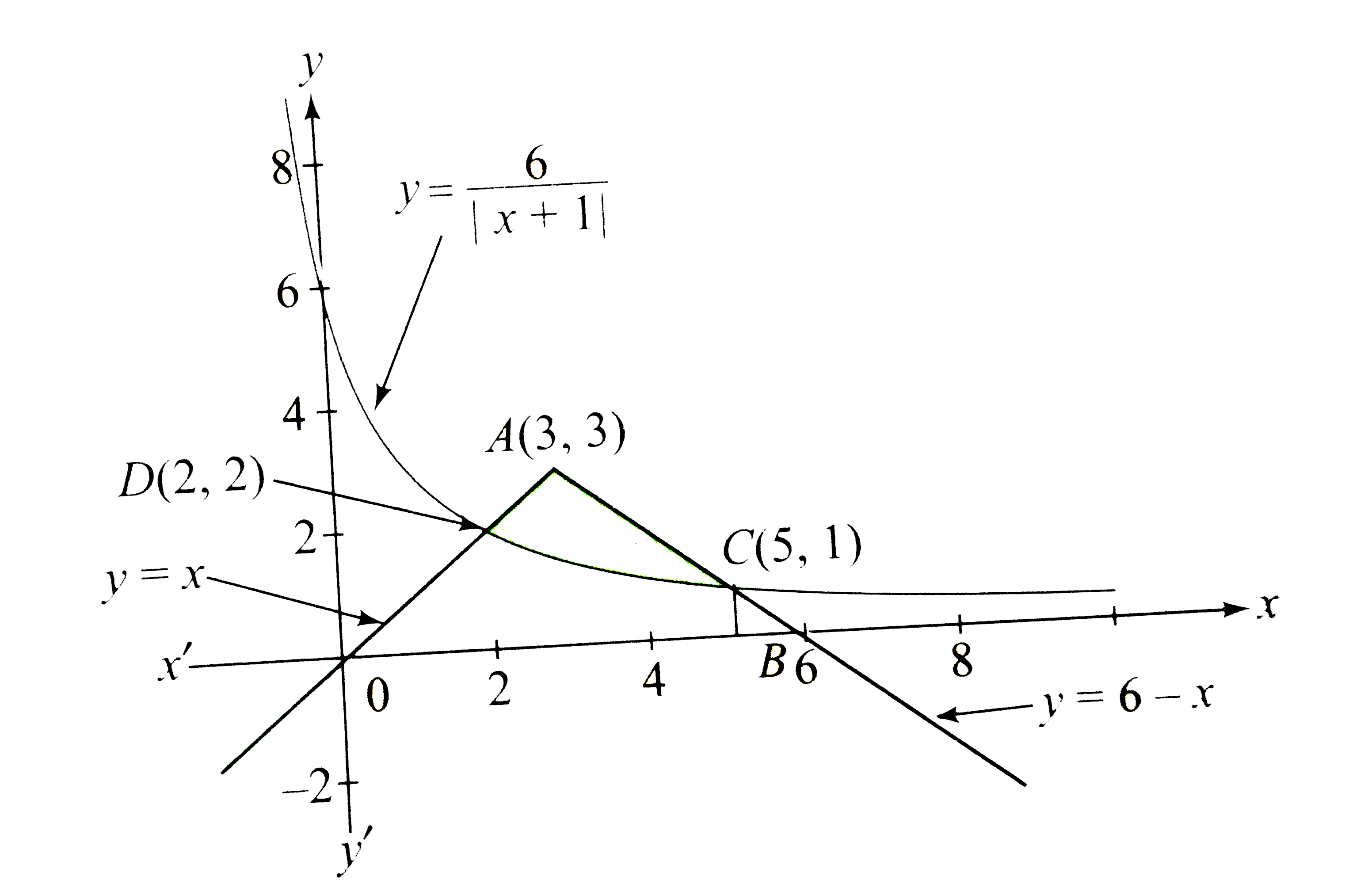
First consider
sq. units.
Topper's Solved these Questions
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Multiple Correct Answers Type
13 Videos
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Linkded Comprehension Type
21 Videos
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Concept Application Exercise 9.3
7 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGE PUBLICATIONCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
Similar Questions
Let A(k) be the area bounded by the curves y=x2−3 and y=kx+2 The range of A(k) is (10√53,∞) The range of A(k) is (20√53,∞) If function k→A(k) is defined for k∈[−2,∞ ), then A(k) is many-one function. The value of k for which area is minimum is 1.
View SolutionLet Ar be the area of the region bounded between the curves y2=(e−kr)x(where k>0,r∈N) and the line y=mx(where m≠0), k and m are some constants
A1,A2,A3,… are in G.P. with common ratio
View SolutionFind the continuous function f where (x4−4x2)≤f(x)≤(2x2−x3) such that the area bounded by y=f(x),y=x4−4x2. then y-axis, and the line x=t, where (0≤t≤2) is k times the area bounded by y=f(x),y=2x2−x3,y-axis , and line x=t(where0≤t≤2).
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionThe area bounded by y=3-|3-x| and y=(6)/(|x+1|) is
View SolutionArea bounded by the curves y=x^(2)-1,x+y=3 is
View SolutionArea bounded by y=x^(3)-x and y=x^(2)+x is
View SolutionThe area of the region bounded by y=1+|x+1|,x=-3,x=3,y=0 is
View SolutionThe area bounded by the curve y=|x-1| and y=3-|x|
View SolutionThe area of the region bounded by y=|x−1|andy=3−|x|, is
View SolutionThe area of the figures bounded by the curves y=|x−1| and y=3 -|x| is-
View SolutionThe area of the region bounded by the curves y=|x−1|andy=3−|x| is
View SolutionThe area of the region bounded by the curves : y=|x-1| and y=3-|x| is :
View SolutionThe area of the region bounded by y = x - 1 and x=3−y2 is
View SolutionThe area bounded by y=3−|3−x| and y=6|x+1| is
View Solution
