The area cut off a parabola $4 y = 3 x^{2}$ by the straight line $2 y = 3 x + 12$ in square units, is

A

$27$ sq units

B

$12$ sq units

C

$33$ sq units

D

$21$ sq units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Clearly $4 y = 3 x^{2}$ is an upward parabola with its vertex at (0,0) And 3x- 2y+12=0 is aline The given equations are
$4 y = 3 x^{2}$
and 3x-2y+12=0
The points of intesection of the given parabola and the given line will be obtined by solving (i) and
(ii) simultaneously
Putting $y = \frac{3}{4} x^{2}$ from (i) in, (ii) ltbegt We get
$3 x - \frac{3}{2} x^{2} + 12 = 0 \Rightarrow x^{2} + 12 = 0$
The points of intersection of the given parabola and the given line will be obtioned by solving (i) and (simulataneously
Putting $y = \frac{3}{4} x^{2}$ from (i) in (ii)
We get
$3 x - \frac{3}{2} x^{3} + 12 = 0 \Rightarrow x^{2} - 2 x - 8 = 0$
$\Rightarrow x^{2} - 4 x + 2 x - 8 = 0$
$\Rightarrow x (x - 4) + 2 (x - 4) = 0$
$\Rightarrow (x - 4) (x + 2) = 0$
$\Rightarrow x = (- 2 or x = 4$
Now , $(x = - 2 \Rightarrow y = 3) and (x = 4 \Rightarrow y = 12) .$
So , the points of intersection of ( i) and (ii) are A(-2,3) and B( 4,12)
Draw $A L ⊥ O X and B M ⊥ O X .$
Required aera= area ALMBA )-(area ALOMBOA)
$= 4 \int - 2 (y of the line ) d x - 4 \int - 2 (y of the parabola) d x$
$= 4 \int - 2 \frac{(3 x + 12)}{2} d x - 4 \int - 2 \frac{3 x^{2}}{4} d x = {[\frac{3 x^{2}}{4} + 6 x]}_{- 2}^{4} - {[\frac{x^{3}}{4}]}_{- 2}^{4}$
$= (45 - 18) = 27$ sq units
Hence the required area is 27 sq units

|

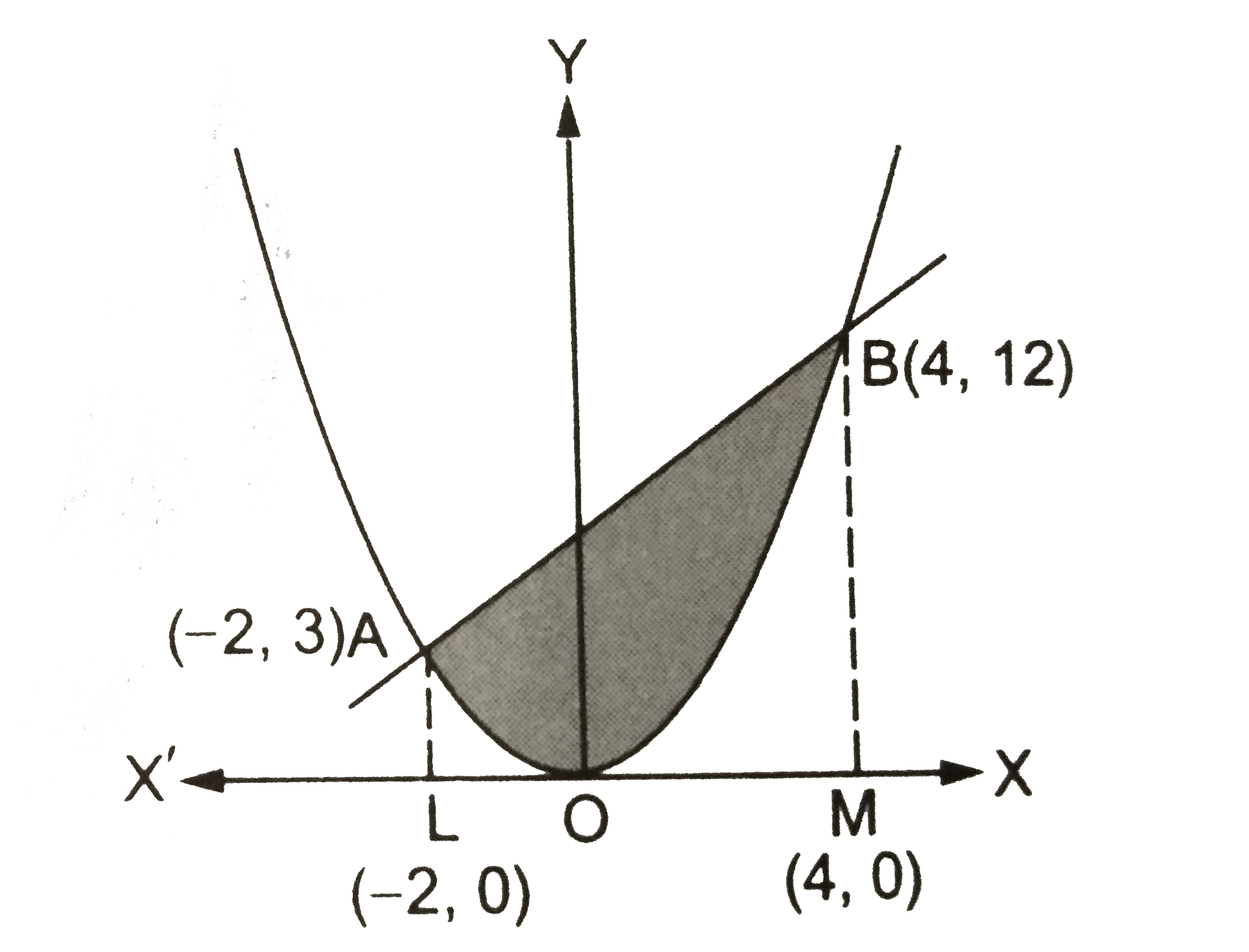
Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area cut off a parabola $4 y = 3 x^{2}$ by the straight line $2 y = 3 x + 12$ in square units, is
A16
B21
C27
D36
Question 2 - Select One
The area of the region bounded by parabola $y^{2} = x$ and the straight line 2y = x is
A $\frac{4}{3} sq units$
B $1 sq unit$
C $\frac{2}{3} sq unit$
D $\frac{1}{3} sq units$
Question 3 - Select One
The area of the region bounded by parabola $y^{2} = x$ and the straight line $2 y = x$ is
A $\frac{4}{3} sq units$
B $1 sq unit$
C $\frac{2}{3} sq unit$
D $\frac{1}{3} sq units$

RS AGGARWAL-AREA OF BOUNDED REGIONS -Exercise 17

Home
Ask Doubt
Profile