The area (in sq. units) of the quadrilateral formed by the tangents at the end points of the latus rectum to the ellipse x29+y25=1 is (a) 274 (b) 18 (c) 272 (d) 27 Solution in Bengali
27/4
18
27/2
27
The correct Answer is:D
The given ellipe is
x29+y25=1
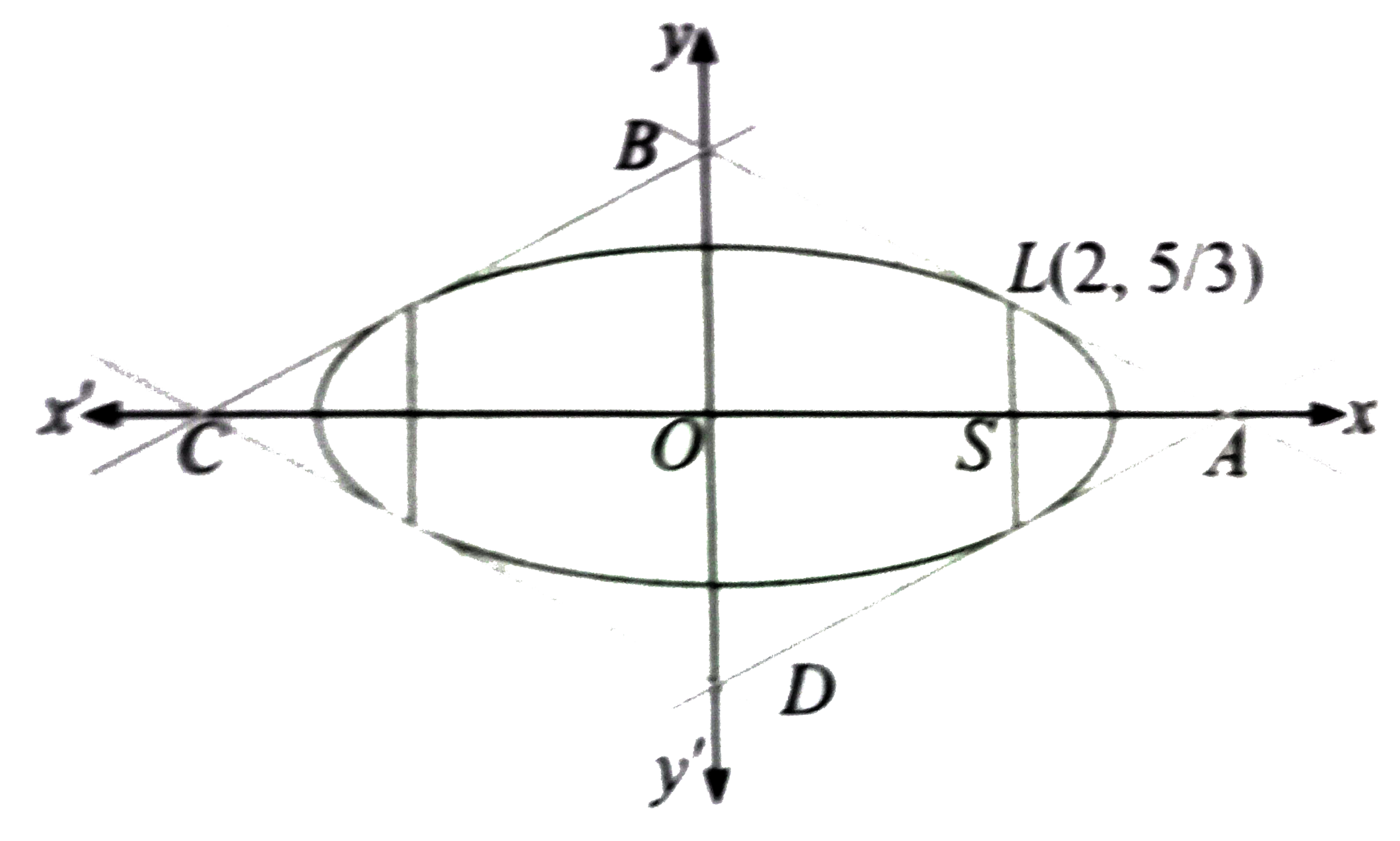
Then a2=9,b2=5. Therefore,
e=√1−59=23 ∴ae=2
Hence, the endpoint of latus rectum in the first quadrant is L(ae,b2/a)≡L(2,5/3)
The equation of tangent at L is 2x9+y3=1
the tangents meets the x-axis at A(9/2,0) and the y-axis at B(0,3). Therefore,
Area of ΔOAPB=12×92×3=274
∴ Area of quadrilateral =4×274=27
Similar Questions
The area (in sq. units) of the quadrilateral formed by the tangents at the end points of the latus rectum to the ellipse x29+y25=1 is (a) 274 (b) 18 (c) 272 (d) 27
View SolutionThe area of the quadrilateral formed by the tangents drawn at extreme points of latus reactum of an ellipse x29+y25=1 is …Sq. units .
View SolutionTangents are drawn to the ellipse x29+y25=1 at the end of latus rectum. Find the area of quadrilateral so formed
View SolutionArea of the quadrilateral formed by the tangents at the ends of latus rectum of x225+y216=1 is (in sq. units)
View SolutionThe area of the quadrilateral formed by the tangents at the end points of latus rectum to the ellipse x29+y25=1 is
View SolutionThe area (in sq. units) of the triangle formed by the latus rectum and the tangents at the end points of the latus rectum of x216−y29=1 is equal to
View SolutionThe area of the quadrilateral formed by the tangents at the endpoint of the latus rectum to the ellipse (x^2)/9+(y^2)/5=1 is (A) 27/4 sq. unit (B) 9 sq. units (C) 27/2 sq. unit (D) 27 sq. unit
View SolutionThe area (in sq. units) of the quadrilateral formed by the tangents at the end points of the latus rectum to the ellipse x29+y25=1 is (a) 274 (b) 18 (c) 272 (d) 27
View Solution
