The average marks scored by Ajay in certain number of test is 84. He scored 100 marks in his last test. His average score of all these tests is 86, then the total number of tests he appeared is.
8
7
9
10
The correct Answer is:A
To solve the problem step by step, we can follow these calculations:
Step 1: Define the variables
Let n be the total number of tests Ajay appeared for before his last test. According to the problem, his average score for these tests is 84.
Step 2: Calculate the total marks before the last test
The total marks scored by Ajay in these n tests can be calculated as:
Total marks=Average×Number of tests=84n
Step 3: Include the last test score
Ajay scored 100 marks in his last test. Therefore, the total marks after including the last test becomes:
Total marks after last test=84n+100
Step 4: Set up the equation for the new average
After including the last test, the total number of tests becomes n+1. The new average score is given as 86. We can set up the equation:
84n+100n+1=86
Step 5: Cross-multiply to eliminate the fraction
Cross-multiplying gives us:
84n+100=86(n+1)
Step 6: Expand and simplify the equation
Expanding the right side:
84n+100=86n+86
Now, rearranging the equation to isolate n:
100−86=86n−84n
14=2n
Step 7: Solve for n
Dividing both sides by 2:
n=7
Step 8: Calculate the total number of tests
Since n is the number of tests before the last one, the total number of tests Ajay appeared for is:
Total tests=n+1=7+1=8
Final Answer
Ajay appeared for a total of 8 tests.
---
Let n be the total number of tests Ajay appeared for before his last test. According to the problem, his average score for these tests is 84.
The total marks scored by Ajay in these n tests can be calculated as:
Total marks=Average×Number of tests=84n
Ajay scored 100 marks in his last test. Therefore, the total marks after including the last test becomes:
Total marks after last test=84n+100
After including the last test, the total number of tests becomes n+1. The new average score is given as 86. We can set up the equation:
84n+100n+1=86
Cross-multiplying gives us:
84n+100=86(n+1)
Expanding the right side:
84n+100=86n+86
Now, rearranging the equation to isolate n:
100−86=86n−84n
14=2n
Dividing both sides by 2:
n=7
Since n is the number of tests before the last one, the total number of tests Ajay appeared for is:
Total tests=n+1=7+1=8
Ajay appeared for a total of 8 tests.
Topper's Solved these Questions
- STATISTICS BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - STATISTICS EXERCISE - Achievers section (Hots)
3 Videos
- STATISTICS BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - STATISTICS EXERCISE - Achievers section (Hots)
3 Videos
- SOME APPLICATIONS OF TRIGONOMETRY BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - SOME APPLICATIONS OF TRIGONOMETRY EXERCISE - ACHIEVERS SECTION (HOTS)
5 Videos
- SURFACE AREAS AND VOLUMES BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - Achievers section (Hots)
3 Videos
Similar Questions
Knowledge Check
In a Mathematics test, the average marks of boys is x% and the average marks of girls is y% with x≠y. If the average marks of all students is z% the ration of the number of girls to the total number of students is
Az−xy−xBz−yy−xCz+yy−xDz+xy−xThe adjoining diagram is frequency polygon for the scores of students in a test. What is the total number of students appeared in the test ?
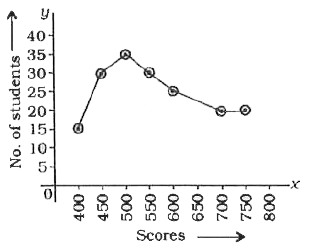 A180B200C175D150
A180B200C175D150The average score of 42 students in a test is 69. The ratio of the number of boysto that of girls is 10: 11. The average score of the boys is 20% more than that of the girls. The average score of the boys is :
A75.2B73.5C75.6D82.8
The average scored by the students of a class in English is 64. The average of marks scored by boys and the girls are respectively 68 and 58. Then find the ratio of the number of boys to the number of girls.
View SolutionThe given bar graph shows the marks obtained by students in a Science test.
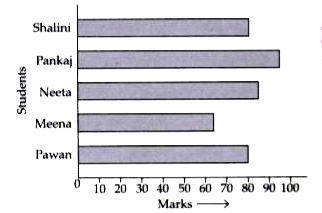
Who scored the highest marks in the test ? Whats in the score ?
View SolutionThe list of marks which students have scored in a class test will fall in the category of
View SolutionThe average score of a cricketer for 13 matches is 42 runs . If his average score for the first 5 matches is 54, then what is his average score (in runs) for last 8 matches ?
View SolutionThree maths classes : X, Y and Z, take an algebra test. The average score of class X is 83. The average score of class Y is 76. The average score of class Z is 85. The average score of class X and Y is 79 and average score of class Y and Z is 81. What is the average score of classes X , Y, Z
View Solution
