The circle with equation x2+y2=1 intersects the line y=7x+5 at two distinct points A and B. Let C be the point at which the positive x-axis intersects the circle. The angle ACB isSolution in Bengali
tan−1(43)
tan−1(34)
π/4
tan−1(32)
The correct Answer is:C
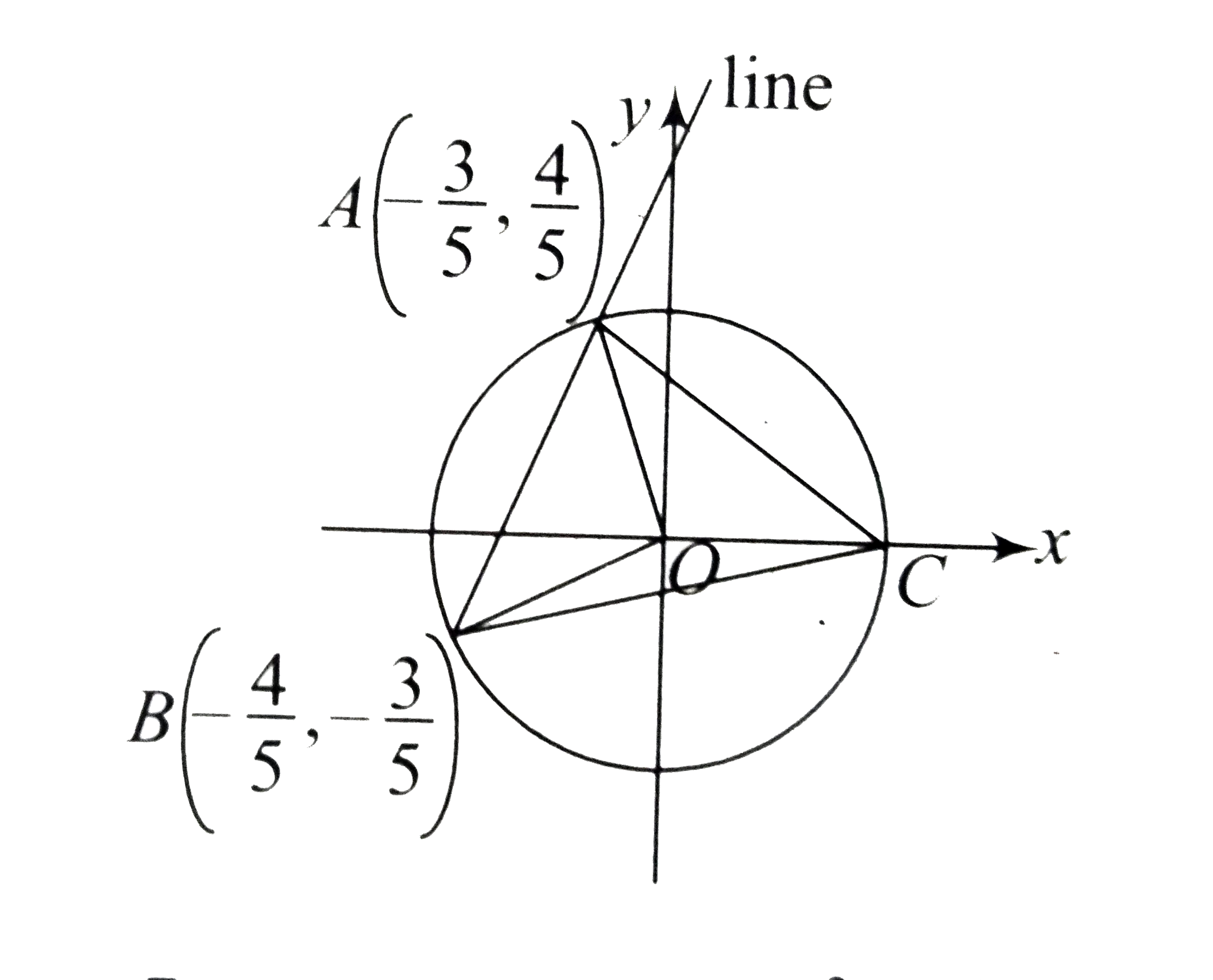
Solving y=7x+5 and the circle x2+y2=1, we get
A(−35,45) and B(−45,−35)
mOA=−43 and mOB=34
Hence, ∠AOD=90∘⇒∠ACB=π4
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Multiple Correct Answers Type
9 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - For problems 3 and 4
2 Videos
- COMPLEX NUMBERSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
Similar Questions
The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is
View SolutionPARAGRAPH X Let S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let E1E2 and F1F2 be the chords of S passing through the point P0(1, 1) and parallel to the x-axis and the y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope −1 . Let the tangents to S at E1 and E2 meet at E3 , the tangents to S at F1 and F2 meet at F3 , and the tangents to S at G1 and G2 meet at G3 . Then, the points E3, F3 and G3 lie on the curve x+y=4 (b) (x−4)2+(y−4)2=16 (c) (x−4)(y−4)=4 (d) xy=4
View SolutionLet z1 and z2 be two distinct complex numbers and z=(1−t)z1+tz2, for some real number t with 0<t<1 and i=√−1. If arg(w) denotes the principal argument of a non-zero compolex number w, then
View SolutionThe circle with equation x^(2)+y^(2)=1 intersects the line y=7x+5 at two distinct points A and B. Let C be the point at which the points A and B.Let C be the point at which the positive x-axis intersects the circle.The angle ACB is
View SolutionThe line x+2y=a intersects the circle x2+y2=4 at two distinct points AandB Another line 12x−6y−41=0 intersects the circle x2+y2−4x−2y+1=0 at two CandD. The value of 'a' for which the points A,B,CandD are concyclic -
View SolutionThe circles x^(2)+y^(2)=4x+8y+5 intersects the line 3x-4y = m at two distinct points of
View SolutionThe line x+2y+a=0 intersects the circle x2+y2−4=0 at two distinct points A and B. Another line 12x-6y-41=0 intersects the circle x2+y2−4x−2y+1=0 at two distincts points C and D.
The value of 'a' so that the line x+2y+a=0 intersects the circle x2+y2−4=0 at two distinct points A and B is
View SolutionStatement-1:If the line y=x+c intersects the circle x^2+y^2=r^2 in two real distinct points then -rsqrt2 lt c lt r sqrt2 Statement -2: If two circles intersects at two distinct points then C_1C_2 lt r_1+r_2
View SolutionThe circle x^2+y^2 =4x+8y+5 intersects the line 3x-4y=m at two distinct points if :
View SolutionThe line y=mx+c intersects the circle x2+y2=r2 in two distinct points if
View SolutionThe circle with equation x2+y2=1 intersects the line y=7x+5 at two distinct points A and B. Let C be the point at which the positive x-axis intersects the circle. The angle ACB is
View SolutionThe line x+2y=a intersects the circle x2+y2=4 at two distinct points AandB Another line 12x−6y−41=0 intersects the circle x2+y2−4x−2y+1=0 at two CandD. The value of 'a' for which the points A,B,CandD are concyclic -
View SolutionThe circle x^2 +y^2=4x+8y+ 5 intersects the line 3x-4y= m at two distinct points, if
View SolutionThe circle with equation x2+y2=1 intersects the line y=7x+5 at two distinct points A and B. Let C be the point at which the positive x-axis intersects the circle. The angle ACB is
View Solution
