Doubtnut Promotions Sticky

The diagonals of a cyclic quadrilateral are at right angles. Prove tha the perpendicular from the point of their intersection on any side when produced backwards , bisects the opposite side.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

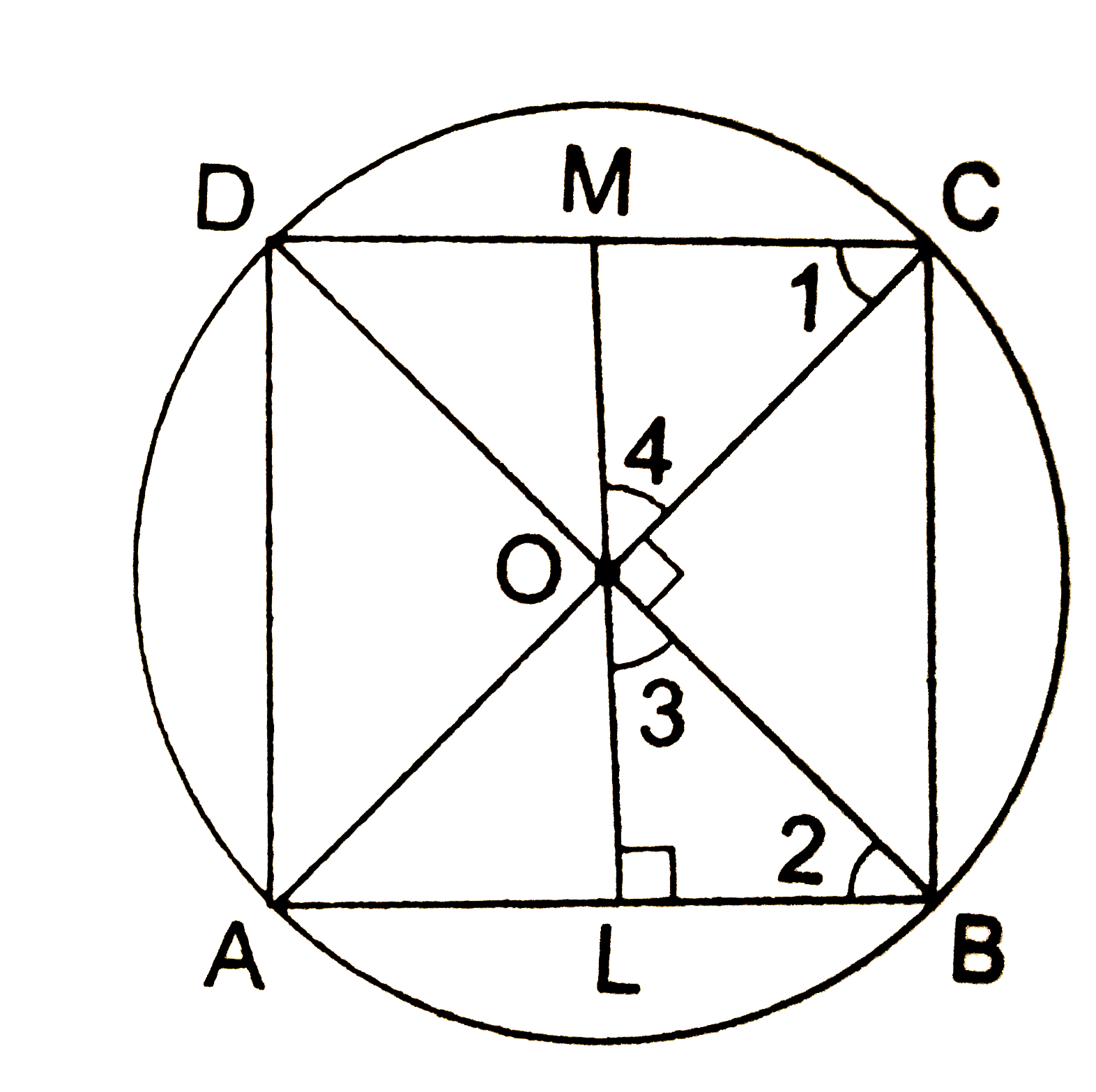
Let ABCD be a cyclic quadrilateral whose diagonals AC and BD intersect at O at right angles . Let OL⊥AB such that LO produced meets CD at M.
Then, we have to prove that CM = MD
Clearly, ∠1=∠2[∠s in the same segment]
∠2+∠3=90∘[∵∠OLB=90∘]
∠3+∠4=90∘[∵LOM is a straight line and ∠BOC=90∘]
Thus, ∠1=∠2 and ∠2=∠4⇒∠1=∠4
∴OM = CM and similarly, OM=MD.
Hence CM=MD
ocr_image

|
Updated on:21/07/2023
Class 9MATHSCIRCLES

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Prove that; If the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.

    Arhombus
    Btrapezium
    Crectangle
    Dkite
  • Question 2 - Select One

    If the diagonals of a quadrilateral bisect each other at right angles, then this quadrilateral is

    Aa rectangle
    Ba rhombus
    Ca kite
    Dnone of these
  • Question 3 - Select One

    Which of the following statements is/are correct?
    The intersections of the angle bisectors of the quadrilateral are the vertices of the cyclic quadrilateral
    If diagonals of a cyclic quadrilateral perpendicularly intersect at point P, then the line through P, perpendicular to any side bisects the opposite side.
    The product of the lengths of the two diagonals of a cyclic quadrilateral is equal to the sum of the products of opposite sides.

    A(i) and (ii)
    B(ii) and (iii)
    C(i) and (iii)
    Dall of these