The dimensions of a plot are 200 m x 150 m. A builder builds 3 roads which are 3 m wide along the length on either side and one in the middle. On either side of the middle road he builds houses to sell. How much area did he get for building the houses?
To solve the problem step by step, we will calculate the area of the plot and then subtract the area occupied by the roads to find the area available for building houses.
Step 1: Calculate the area of the plot
The area of a rectangle is calculated using the formula:
Area=Length×Breadth
Given the dimensions of the plot:
- Length = 200 m
- Breadth = 150 m
So, the area of the plot is:
Area of plot=200m×150m=30000m2
Step 2: Calculate the area of one road
The builder constructs three roads, each 3 m wide. Since the roads run along the length of the plot, we can calculate the area of one road using the formula:
Area of one road=Length×Width
Given:
- Length of the plot = 200 m
- Width of one road = 3 m
So, the area of one road is:
Area of one road=200m×3m=600m2
Step 3: Calculate the total area of the three roads
Since there are three roads, the total area occupied by the roads is:
Total area of roads=3×Area of one road=3×600m2=1800m2
Step 4: Calculate the area available for building houses
To find the area available for building houses, we subtract the total area of the roads from the area of the plot:
Area for houses=Area of plot−Total area of roads
Area for houses=30000m2−1800m2=28200m2
Final Answer
The area available for building houses is:
Area for houses=28200m2
---
The area of a rectangle is calculated using the formula:
Area=Length×Breadth
- Length = 200 m
- Breadth = 150 m
Area of plot=200m×150m=30000m2
The builder constructs three roads, each 3 m wide. Since the roads run along the length of the plot, we can calculate the area of one road using the formula:
Area of one road=Length×Width
- Length of the plot = 200 m
- Width of one road = 3 m
Area of one road=200m×3m=600m2
Since there are three roads, the total area occupied by the roads is:
Total area of roads=3×Area of one road=3×600m2=1800m2
To find the area available for building houses, we subtract the total area of the roads from the area of the plot:
Area for houses=Area of plot−Total area of roads
Area for houses=30000m2−1800m2=28200m2
The area available for building houses is:
Area for houses=28200m2
Similar Questions
A farmer wants to plough his square field of side 150m. How much area will he have to plough?
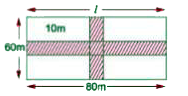
View SolutionA rectangular grass plot 80m×60m has two roads, each 10 m wide, running in the middle of it, one parallel to length and the other parallel to breadth.Find the area of the roads.
 View Solution
View SolutionA rectangular lawn is 30 m by 20 m. It has two roads each 2 m wide running in the middle of it one parallel to the length and the other parallel to the breadth. Find the area of the roads.
View SolutionIn a rectangular park, there is an elliptical flower bed in the middle and the border. The side lengths of the park are 30m and 20m. Major axis & minor axis are of length 14m and 10m respectively. Around the flower bed in the middle there is a walking path 1.4 m wide and in the middle of each side there is entrance of 2m width to the park upto the walking path. At the border the width of flower bed is Im. Rest of the park is grass area. If the entire walking path is covered by bricks of dimension 10 x 20 cm2, find the approximate number of bricks required. If cost of reading the grass and plating the flowers is Rs.10 and Rs:.50 per square metre and cost of a brick is Rs.2. Find total cost of renovation
View SolutionA girl is sitting in the middle of a park of dimension 12m×12m. On the left side of it there is a building adjoining the park and on right side of the park, there is a road adjoining the park. A sound is produced on the road by a cracker. Is it possible for the girl to hear the echo of this sound ? Explain your answer.
View SolutionA building with the base in the form of a regular hexagon of side 7 m stands in a huge field. In one corner of the building, a cow is tethered with a rope of length 14 m. Find the area grazed by the cow.
View SolutionTwo poles of equal heights are standing opposite to each other on either side of the road which is 80 m wide. From a point between them on the road the angles of elevation of the top of the poles are 60∘ and 30∘ respectively. Find the height of the poles and the distances of the point from the poles.
View SolutionThrough a rectangular field of sides 90m×60m, two roads are constructed which are parallel to the sides and cut each other at right angles through the centre of the field. If the width of the roads is 3 m, find the total area covered by the two roads.
View SolutionThere is a rectangular lawn 20m long and 6m wide in front of a house (Fig. 6.12). It is fenced along the two smaller sides and one longer side leaving a gap of 4m for the entrance. Find the length of fencing.
View SolutionTwo poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60∘and 30∘, respectively. Find the height of the poles and the distances of the point from the poles.
View Solution

