The feasible solution of LPP ………..Solution in Gujrati
satisfy all the constraints
satisfy some of the constraints
always corner points of feasible solution
always optimal value of objective function
The correct Answer is:A
Step by step video, text & image solution for The feasible solution of LPP ……….. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - PRACTICE PAPER - 1214videos
LINEAR PROGRAMMING
- BOOK - KUMAR PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - PRACTICE WORK25videos
LINEAR PROGRAMMING
- BOOK - KUMAR PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - SOLUTIONS OF NCERT EXEMPLAR PROBLEMS44videos
LINEAR PROGRAMMING
- BOOK - KUMAR PRAKASHANCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - PRACTICE PAPER - 2 (SECTION - D)2videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - KUMAR PRAKASHANCHAPTER - MATRICES EXERCISE - Practice Paper - 3 (Section - D)1videos
MATRICES
Similar Questions
The correct points of the feasible region determined by the following system of linear inequalities, 2x+y≤10,x+3y≤15,x,y≥0, are (0, 0), (5, 0), (3, 4) and (0, 5). Let Z = px + qy, where p,q>0. Condition on p and q so that the maximum of Z occurs at both (3, 4) and (0, 5) is ..........
01:47View SolutionSolve the following linear programming problems graphically :
Minimise : Z = 3x + 2y subject to the constraints x+y≤8,3x+5y≥15,x≥0,y≥000:35View SolutionDetermine the maximum value of z = 11x + 7y subject to the constraints :
2x+y≤6,x≤2,x≥0,y≥000:49View SolutionProve that :
cot−1(√1+sinx+√1−sinx√1+sinx−√1−sinx)=x2,x∈(0,π4)01:24View SolutionFor matrix A=⎡⎢⎣11112−32−13⎤⎥⎦ . Prove that , A3−6A2+5A+11I=0. Hence find A−1 using it .
02:06View SolutionThe feasible solution for a LPP is shown in following figure. Let Z=3x−4y be the objective function, Minimum of Z occurs at
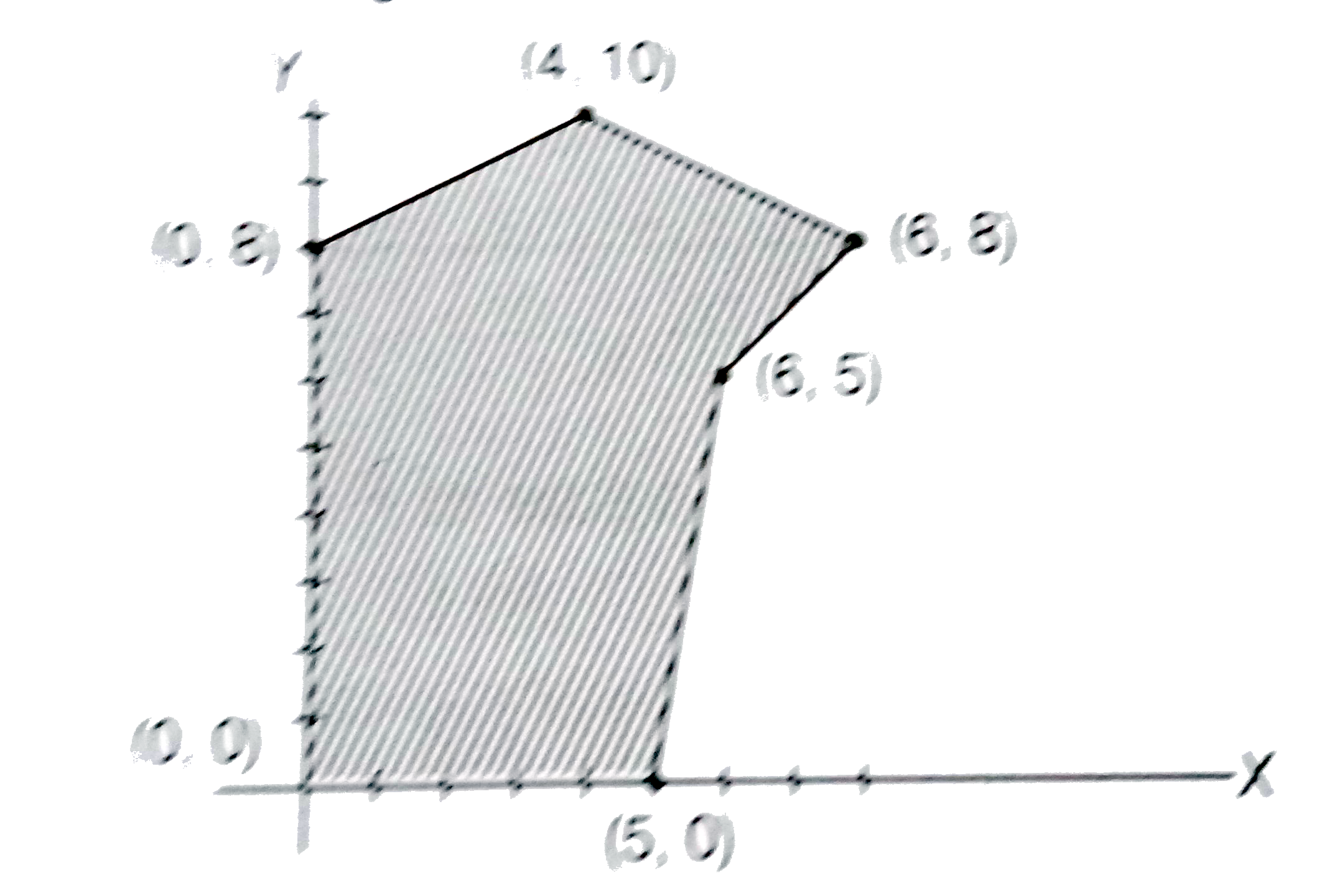 02:01View Solution
02:01View SolutionThe feasible region for an LPP is always a..polygon
00:45View SolutionThe feasible solution of a LPP belongs to
00:58View SolutionDefine the term Feasible Solution in a LPP.
03:50View SolutionDefine the term corner point of a feasible region in an LPP.
02:01View SolutionStatement I : The common region determined by all the constraints of a LPP is called the feasible region
Statement II : A solution that also satisfies the non negativtiy restrications of a LPP is called the feasible soltion01:59View SolutionIf an LPP admits optimal solution at two consecutive vertices of a feasible region, then
View SolutionThe feasible region for an LPP is always a..polygon
03:11View SolutionDefine Feasible region in LPP.
03:00View SolutionThe feasible solution for a LPP is shown in Figure
Let z = -3x - 4y
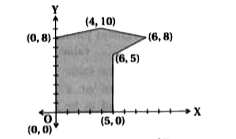
objective function. Minimum of Z occurs at03:54View Solution

