The following cumulative frequency distribution table shows the daily electricity consumption (in kW) of 40 factories in an industrial state: Consumption (in kW) No. of Factories Below 240 Below 270 Below 300 Below 330 Below 360 Below 390 Below 420 1 4 8 24 33 38 40 Represent this as a frequency distribution table
To convert the given cumulative frequency distribution table into a frequency distribution table, we will follow these steps:
Step 1: Identify the Class Intervals
The cumulative frequency table provides ranges of electricity consumption. We will define the class intervals based on the cumulative frequencies provided.
- Below 240 kW: 0 to 240
- Below 270 kW: 240 to 270
- Below 300 kW: 270 to 300
- Below 330 kW: 300 to 330
- Below 360 kW: 330 to 360
- Below 390 kW: 360 to 390
- Below 420 kW: 390 to 420
Step 2: Write the Cumulative Frequencies
The cumulative frequencies given in the table are:
- Below 240: 1
- Below 270: 4
- Below 300: 8
- Below 330: 24
- Below 360: 33
- Below 390: 38
- Below 420: 40
Step 3: Calculate the Frequencies
To find the frequency for each class interval, we will subtract the cumulative frequency of the previous class from the cumulative frequency of the current class.
1. For 0 to 240: Frequency = 1 (remains the same)
2. For 240 to 270: Frequency = 4 - 1 = 3
3. For 270 to 300: Frequency = 8 - 4 = 4
4. For 300 to 330: Frequency = 24 - 8 = 16
5. For 330 to 360: Frequency = 33 - 24 = 9
6. For 360 to 390: Frequency = 38 - 33 = 5
7. For 390 to 420: Frequency = 40 - 38 = 2
Step 4: Create the Frequency Distribution Table
Now, we can compile the class intervals and their corresponding frequencies into a frequency distribution table.
| Consumption (in kW) | No. of Factories |
|----------------------|------------------|
| 0 - 240 | 1 |
| 240 - 270 | 3 |
| 270 - 300 | 4 |
| 300 - 330 | 16 |
| 330 - 360 | 9 |
| 360 - 390 | 5 |
| 390 - 420 | 2 |
Step 5: Verify the Total Frequency
To ensure our frequency distribution table is correct, we will sum the frequencies:
1 + 3 + 4 + 16 + 9 + 5 + 2 = 40
The total is 40, which matches the total number of factories given in the problem.
Final Frequency Distribution Table
| Consumption (in kW) | No. of Factories |
|----------------------|------------------|
| 0 - 240 | 1 |
| 240 - 270 | 3 |
| 270 - 300 | 4 |
| 300 - 330 | 16 |
| 330 - 360 | 9 |
| 360 - 390 | 5 |
| 390 - 420 | 2 |
---
The cumulative frequency table provides ranges of electricity consumption. We will define the class intervals based on the cumulative frequencies provided.
- Below 270 kW: 240 to 270
- Below 300 kW: 270 to 300
- Below 330 kW: 300 to 330
- Below 360 kW: 330 to 360
- Below 390 kW: 360 to 390
- Below 420 kW: 390 to 420
The cumulative frequencies given in the table are:
- Below 240: 1
- Below 270: 4
- Below 300: 8
- Below 330: 24
- Below 360: 33
- Below 390: 38
- Below 420: 40
To find the frequency for each class interval, we will subtract the cumulative frequency of the previous class from the cumulative frequency of the current class.
2. For 240 to 270: Frequency = 4 - 1 = 3
3. For 270 to 300: Frequency = 8 - 4 = 4
4. For 300 to 330: Frequency = 24 - 8 = 16
5. For 330 to 360: Frequency = 33 - 24 = 9
6. For 360 to 390: Frequency = 38 - 33 = 5
7. For 390 to 420: Frequency = 40 - 38 = 2
Now, we can compile the class intervals and their corresponding frequencies into a frequency distribution table.
|----------------------|------------------|
| 0 - 240 | 1 |
| 240 - 270 | 3 |
| 270 - 300 | 4 |
| 300 - 330 | 16 |
| 330 - 360 | 9 |
| 360 - 390 | 5 |
| 390 - 420 | 2 |
To ensure our frequency distribution table is correct, we will sum the frequencies:
| Consumption (in kW) | No. of Factories |
|----------------------|------------------|
| 0 - 240 | 1 |
| 240 - 270 | 3 |
| 270 - 300 | 4 |
| 300 - 330 | 16 |
| 330 - 360 | 9 |
| 360 - 390 | 5 |
| 390 - 420 | 2 |
Topper's Solved these Questions
Similar Questions
Given below is a cumulative frequency distribution showing the mars secured by 50 students of a class

From the frequency distribution table for the data.
View SolutionGiven below are the cumulative frequencies showing the weights of 685 students of a school. Prepare a frequency distribution table. Weight (in kg), No. of students Below 25 Below 30 Below 35 Below 40 Below 45 Below 50 Below 55 Below 60 Below 65 Below 70, 0 24 78 183 294 408 543 621 674 685
View SolutionGiven below is distribution of daily earnings of 60 workers in a factory

Find 45th percentile.
View SolutionFind the mean of the frequency distribution:
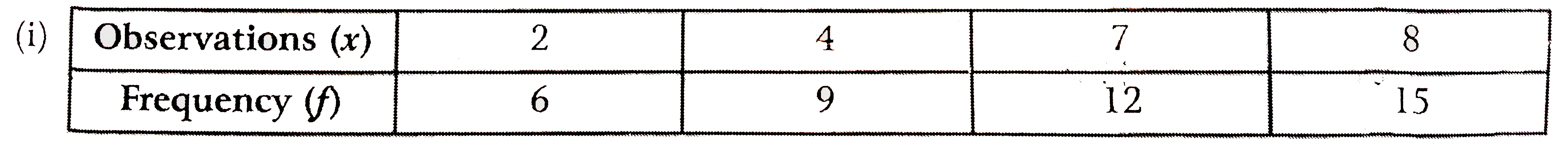
(ii) The weight of 40 students of class IX are given below:
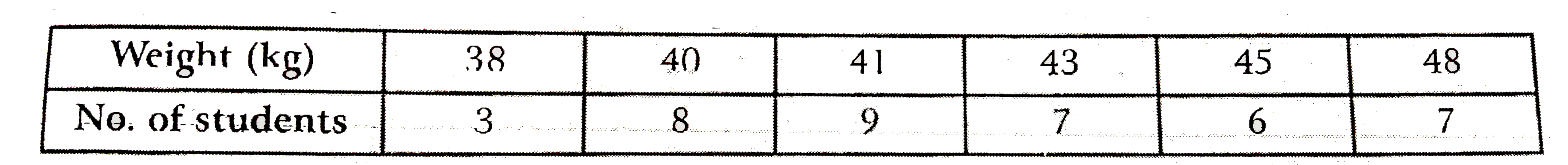
View SolutionConstruct the frequency distribution table from the following cumulative frequency table:
AgesNo of studentsBelow40Below785Below10140Below13243Below16300
State the age group which has the least number of students
View SolutionConstruct the frequency distribution table from the following cumulative frequency table:
AgesNo of StudentsBelow40Below785Below10140Below13243Below16300
State the number of students in the age group 10-13
View SolutionA cumulative frequency distribution is given below. Convert this into a frequency distribution table. Marks Below 45 Below 60 Below 75 Below 90 Below 105 Below 120 No. of Students 0 8 23 48 85 116
View SolutionA cumulative frequency distribution is given below. Convert this into a frequency distribution table. Marks Below 45 Below 60 Below 75 Below 90 Below 105 Below 120 No. of Students 0 8 23 48 85 116
View SolutionA life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are only given to persons having age 18 years onwards but less than 60 years. Age(inyears) Numberofpolicyholders Below20 2 Below25 6 Below 30 24 Below 35 45 Below 40 78 Below 45 89 Below 50 92 Below 55 98 Below 60 100
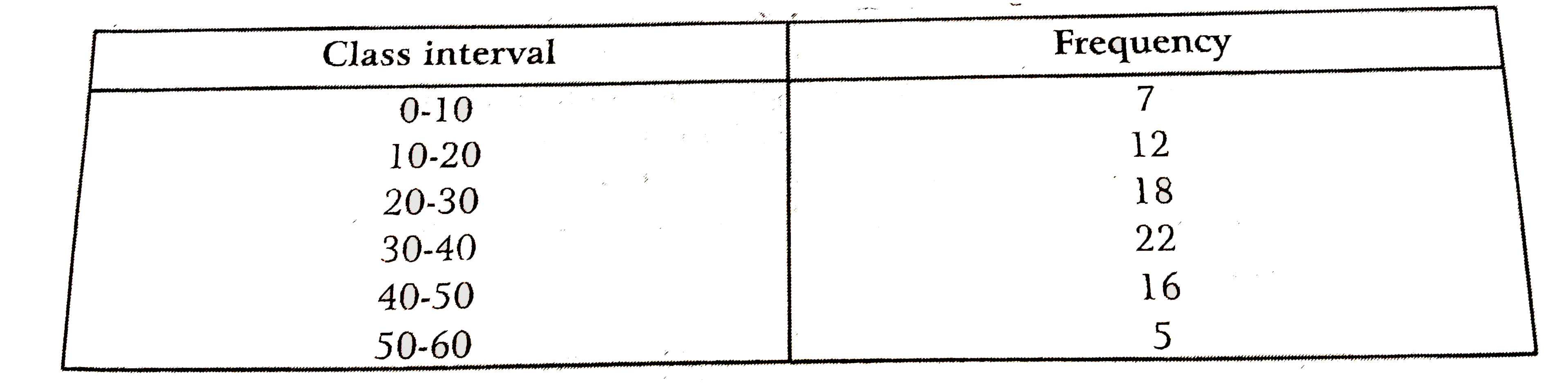
View SolutionThe table given below shows a frequency distribution table, find
(a) Upper limit of third class (b) Lower limit of fifth class
(c) Class size (d) Class mark of third class
View Solution
