The following is the p.d.g. (Probability Density Function) of a continuous random varible X:
f(x)=x32,0<x<8=0, otherwise
(a) Find following the expression for c.d.f. (Cumulative Distribution Function) of X.
(b) Also find its value at x=0.5and9.
To solve the problem, we will follow these steps:
Step 1: Define the Probability Density Function (PDF)
The given PDF of the continuous random variable X is:
f(x)=x32,0<x<8
and f(x)=0 otherwise.
Step 2: Find the Cumulative Distribution Function (CDF)
The CDF, denoted as F(x), is defined as:
F(x)=∫x−∞f(t)dt
Since f(t)=0 for t<0, we can change the limits of integration:
F(x)=∫x0f(t)dt
For 0<x<8:
F(x)=∫x0t32dt
Step 3: Calculate the Integral
Now, we will compute the integral:
F(x)=132∫x0tdt
The integral of t is:
∫tdt=t22
Thus,
F(x)=132(t22)x0=132(x22−0)=x264
Step 4: Define the CDF for all x
The complete CDF can be expressed as:
F(x)=⎧⎪⎨⎪⎩0if x≤0x264if 0<x<81if x≥8
Step 5: Evaluate the CDF at Specific Points
Now we will find F(0.5) and F(9).
1. For x=0.5:
F(0.5)=(0.5)264=0.2564=1256
2. For x=9:
Since 9≥8, we use the definition of the CDF for x≥8:
F(9)=1
Final Answers
(a) The expression for the CDF F(x) is:
F(x)=⎧⎪⎨⎪⎩0if x≤0x264if 0<x<81if x≥8
(b) The values are:
- F(0.5)=1256
- F(9)=1
The given PDF of the continuous random variable X is:
f(x)=x32,0<x<8
and f(x)=0 otherwise.
The CDF, denoted as F(x), is defined as:
F(x)=∫x−∞f(t)dt
Since f(t)=0 for t<0, we can change the limits of integration:
F(x)=∫x0f(t)dt
For 0<x<8:
F(x)=∫x0t32dt
Now, we will compute the integral:
F(x)=132∫x0tdt
The integral of t is:
∫tdt=t22
Thus,
F(x)=132(t22)x0=132(x22−0)=x264
The complete CDF can be expressed as:
F(x)=⎧⎪⎨⎪⎩0if x≤0x264if 0<x<81if x≥8
Now we will find F(0.5) and F(9).
F(0.5)=(0.5)264=0.2564=1256
Since 9≥8, we use the definition of the CDF for x≥8:
F(9)=1
(a) The expression for the CDF F(x) is:
F(x)=⎧⎪⎨⎪⎩0if x≤0x264if 0<x<81if x≥8
- F(0.5)=1256
- F(9)=1
Topper's Solved these Questions
- MARCH 2016BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2016EXERCISE - SECTION II
20 Videos
- MARCH 2015BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2015EXERCISE - SECTION-II
20 Videos
- MARCH 2017BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2017EXERCISE - SECTION-II
20 Videos
Similar Questions
The following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Find the expression for c.d.f (Cumulative Distribution Function ) of X
View SolutionThe following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Also, find its value at x = 0.5 and 9.
View Solution
Knowledge Check
If the p.d.f of a continuous random variable X is
f(x)=kx2(1−x),0<x<1
= 0 otherwise
Then the value of k isA8B10C12D16The p.d.f of a continuous random variable X is f(x)=x23,−1<x<2 0 = otherwise Then the c.d.f of X is
Ax39+19Bx39−19Cx24+14D19x3+19The p.d.f of a continous random variable X is
f(x)=x8,0<x<4
=0, otherwise
Then the value of P(X>3) isA316B516C716D916
The following is the p.d.f of continuous random variable X. f(x)=x8,0<x<4
=0 , otherwise.
Find the expression for c.d.f of X
View SolutionThe following is the p.d.f of continuous random variable X. f(x)=x8,0<x<4
=0 , otherwise.
Also , find its value at x = 0.5 , 1.7 and 5
View SolutionGiven the probability density functio (p.d.f) of a continuos random variable X as. f(x)=x23,−1<x<2
Determine the cumulative distribution function (c.d.f) X and hence find P(X<1),P(X>0),P(1<X<2).
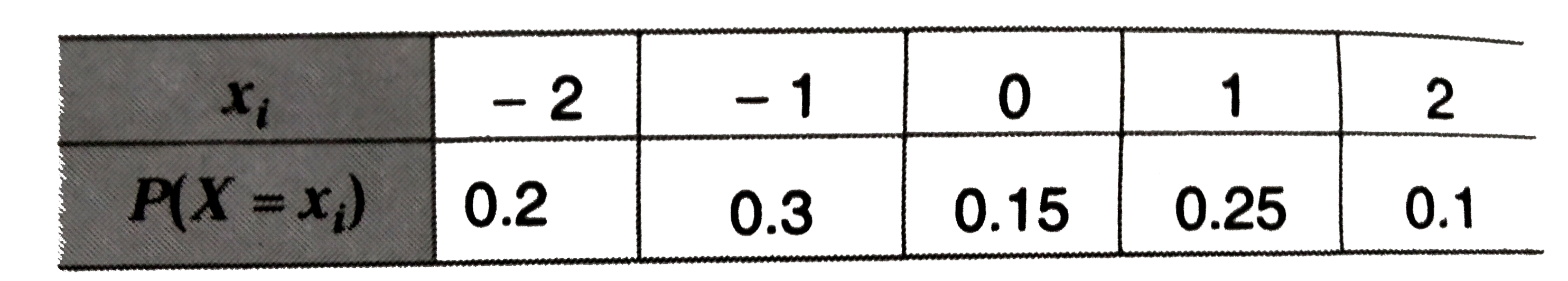
View SolutionIf the probability density function of a random variable X is given as
then F(0) is equal to
View SolutionThe p.d.f. of a continuous random variable X is f(x)=K√x,0<x<4
= 0 , otherwise
Then P(X≥1) is equal to
View Solution
