The following values are calculated in respect of heights and weights of the students of a section of class XI: Height Weight Mean 162.6 cm 52.36 kg Variance 127.69cm2 23.1361 kg2 Can we say that the weights show greater variation than the heights?
The correct Answer is:Yes. we can say that weights show more variability than heights.
To compare the variability, we have to calculate their coefficients of variation.
Given, variance of height
Therefore, standard deviation of height
Also, variance of weight
Therefore, standard deviation of weight
$$
=\sqrt{23.1361}=4.81 k g
$$
Now, the coefficient of variations are given by
$$
\begin{aligned}
&(C . V .) \text { in heights }=\frac{\text { Standerd Deviation }}{\text { Mean }} \times 100 \\
&=\frac{11.3}{162.6} \times 100=6.95
\end{aligned}
$$
and in weights
Clearly . in weights is greater than the . in heights.
Therefore, we can say that weights show more variability than heights.
Topper's Solved these Questions
Similar Questions
The following values are calculated in respect of heights and weights of the students of a section of Class XI
:
Can we say that the weights show greater variation than the
View SolutionThe following values are calculated in respect of heights and weights of the students of a section of class XI: Height Weight Mean 162.6 cm 52.36 kg Variance 127.69cm2 23.1361 kg2 Can we say that the weights show greater variation than the heights?
View SolutionThe means and standard deviations of heights ans weights of 50 students of a class are as follows: Weights Heights Mean 63.2 kg 63.2 inch Standard deviation 5.6 kg 11.5 inch Which shows more variability, heights or weights?
View SolutionFollowing table shows the weight of 12 students: Weight (in kgs) 67 70 72 73 75 Number of Students: 4 3 2 2 1 Find the mean weight of the students.
View SolutionA 6kg weight is fastened to the end of a steel wire of unstretched length 60cm. It is whirled in a vertical circle and has an angular velocity of 2rev/s at the bottom of the circle. The area of cross - section of the wire is 0.05cm2 . Calculate the elongation of the wire when the weight is at the lowest point of the path . Young's modulus of steel =2×1011N/m2 .
View SolutionA uniform one metre long rod AB of 17 kg weight I suspended horizontally from fixed supports by two vertical strings attached to points C and D on the rod at distances of 12 cm and 16 cm from A and B respectively .The strings at C and D can support weights of 10 kg and 9 kg respectively without breaking .Find where a weight of 2 kg can be attached to the rod without breaking either of the string .
View SolutionFollowing frequency distribution given the weights of 38 students of a class:
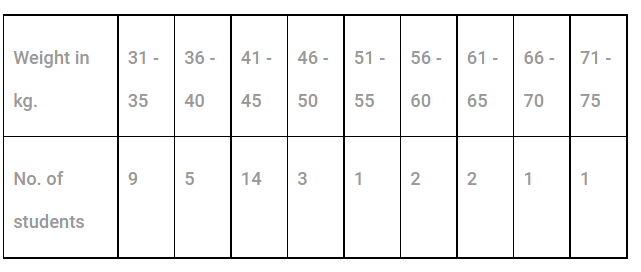
Find the probability that weight of a student in the class is:
View SolutionA man stands in the centre of a Zhukovskii turntable (a rotating platform with frictionless bearings) and rotates with it at 30 r.p.m. The moment of inertia of the man's body with respect to the axis of rotation is about 1.2kg.m2 . The man holds in his outstretched hands two weights of mass 3 kg each. The distance between the weights is 160 cm. What will be the change in the speed of rotation of the system, if the man lets his hands fall so that the distance between the weights becomes 40 cm? The moment of inertia of the turntable is 0.6 kg. m2 the change in the moment of inertia of the man's hands and the friction are to be neglected,
View SolutionHanumappa and his wife Gangavva are busy making Jaggery out of sugar-cane. They have processed the sugarcane juice to make the molasses which is poured into moulds of the shape shown in Fig 16.72. It will be cooled to solidify in this shape to be sent to the market. Each mould is in the sh ape of a frustum of a cone having the diameters of its two circular ends as 30 cm and 35 cm and the height of the mould is 14 cm. If each cm3 of molasses weighs about 1.2 gm, find the weight of molasses that can be poured into each mould (Take π=22/7 )
View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View Solution
