The graph of f(x)=x2 and g(x)=cx3 intersect at two points, If the area of the region over the interval [0,1c] is equal to 23, then the value of (1c+1c2) is
20
2
6
12
The correct Answer is:c
The two curves intersect at A(1c,1c2) and O (0,0) as shown in 33.
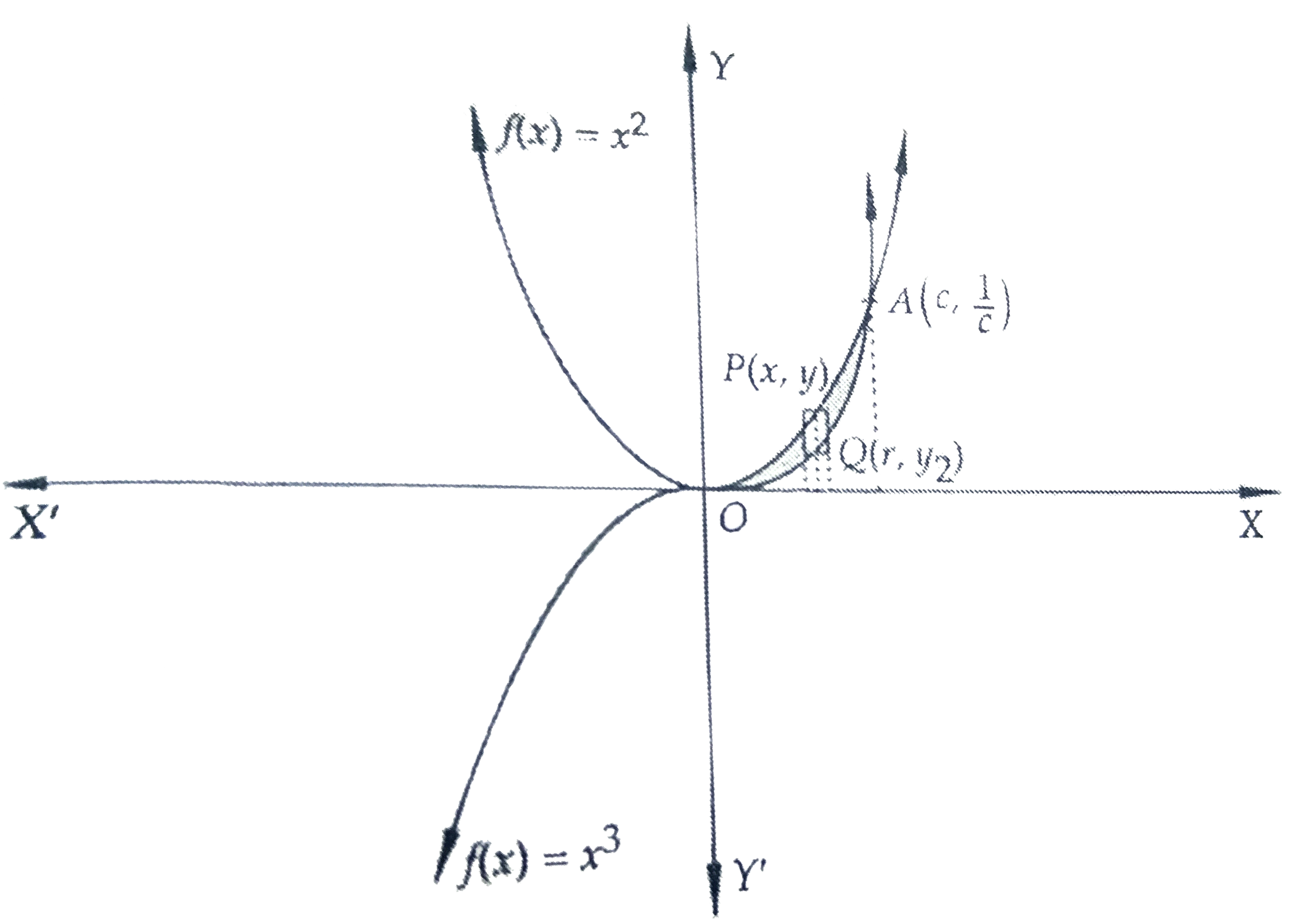
∴1/c∫0(y1−y2)dx=23
⇒1/c∫0(x2−cx3)dx=23
⇒[x33−cx44]1/c0=23
=112c3−23⇒c=12
Hence, 1c+1c2=2+4=6.
Topper's Solved these Questions
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
62 Videos
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- APPLICATION OF DERIVATIVESBOOK - OBJECTIVE RD SHARMACHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Illustration
6 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos
Similar Questions
If x lies in the interval [0, 1] , then the least value of x2+x+1 is (a) 3 (b) 3/4 (c) 1 (d) none of these
View SolutionA function y−f(x) has a second-order derivative fx=6(x−1). It its graph passes through the point (2,1) and at that point tangent to the graph is y=3x−5, then the value of f(0) is 1 (b) −1 (c) 2 (d) 0
View Solution
Knowledge Check
Let mean value of f(x)=1x+c over interval (0,2) is 12ln3 then positive value of c is
A1B12C2D32If f(x)=x2−6x+8 and there exists a point c in the interval [2,4] such that f'(c)=0, then what is the value of c?
A2.5B2.8C3D3.5If f(x)=x2−6x+8 and there exists a point c in the interval [2, 4] such that f'( c ) =0, then what is the value of c ?
A2.5B2.8C3D3.5
Let the functions : (−1,1)→Randg:(−1,1)→(−1,1) be defined by f(x)=|2x−1|+|2x+1|andg(x)=x−[x] where [ x ] denotes the greatest integar less than or equal to x, Let f∘g(−1,1)→R be the composite function defined by (f∘g)(x)=f(g(x)). Suppose c is the number of points in the interval (−1,1) at which f∘g is NOT continuous , and suppose d is the number of points in the interval (−1,1) at which f∘g is NOT differentiable . Then the value of c + is ______
View SolutionIf f(x)=x2+2bx+2c2andg(x)=−x2−2cx+b2 such that minf(x)>maxg(x), then the relation between aandc is (1) Non real value of bandc (2) 0<cbsqr2 (3) |c|<|b|√2 (4) |c|>|b|√2
View SolutionIf Rolle's theorem holds for the function f(x)=2x3+bx2+cx On the interval [−1,1] at the point K=12 then the value of 9b−c47=............
View SolutionIf A > 0, c,d,u.v are non-zero constants and the graph of f(x)=|Ax+c|+d and g(x)=−|Ax+u|+v intersect exactly at two points (1,4) and (3,1), then the value of u+cA equals
View SolutionSuppose that the quadratic function f(x)=ax2+bx+c is non-negative on the interval [-1,1] . Then, the area under tha graph of f over the interval [-1,1] and the X - axis is given by the formula
View Solution
