The length of side of a square is 'a' metre. A second square is formed by joining the middle points of this square. Then a third square is formed by joining the middle points of the sides of the second square and so on. Then, the sum of the areas of squares which carried upto infinity, is
a2
2a2
3a2
4a2
The correct Answer is:C
Step by step video, text & image solution for The length of side of a square is 'a' metre. A second square is formed by joining the middle points of this square. Then a third square is formed by joining the middle points of the sides of the second square and so on. Then, the sum of the areas of squares which carried upto infinity, is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMACHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise
129 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test
50 Videos
- SETSBOOK - OBJECTIVE RD SHARMACHAPTER - SETSEXERCISE - Chapter Test
30 Videos
Similar Questions
Knowledge Check
A side of a square is 16 cm. A new square is formed by joining the midpoints of sides, again a new square is formed by joining the mid-points of the new square and this process goes on till infiity. Find the total area of such square formed ?
A256cm2B128cm2C512cm2D1024cm2The area of a square ABCD is 36cm2. Find the area of the square obtained by joining the midpoints of the sides of the square ABCD.
A17 cm2B18 cm2C28 cm2D10 cm2If the side of a square is doubled, then the area of the square
Aremains sameBbecomes doubleCbecomes tripleDbecomes 4 times
The diagonal of a square is 4√2cm. The diagonal of another square whose area is double that of the first square is :
View SolutionThe figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only, if
View SolutionThe figure formed by joining the mid-points of the sides of a quadrilateral ABCD taken in order is a square only, if
View SolutionIn the figure shown square II is formed by joining the mid-points of square II

and so on . In this way total five squares are drawn . The sides of the square I is 'a' cm.
What is the total area of all the five squares ?
View SolutionIn the figure shown square II is formed by joining the mid-points of square I
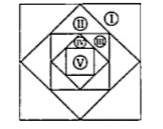
and so on . In this way total five squares are drawn . The sides of the square I is 'a' cm.
What is the perimeter of all the five squres ?
View Solution
