The line x+2y=a intersects the circle x2+y2=4 at two distinct points AandB Another line 12x−6y−41=0 intersects the circle x2+y2−4x−2y+1=0 at two CandD. The value of a for which the points A,B,CandD are concyclic -
A
1
B
3
C
4
D
2
Video Solution
Text Solution
The correct Answer is:4
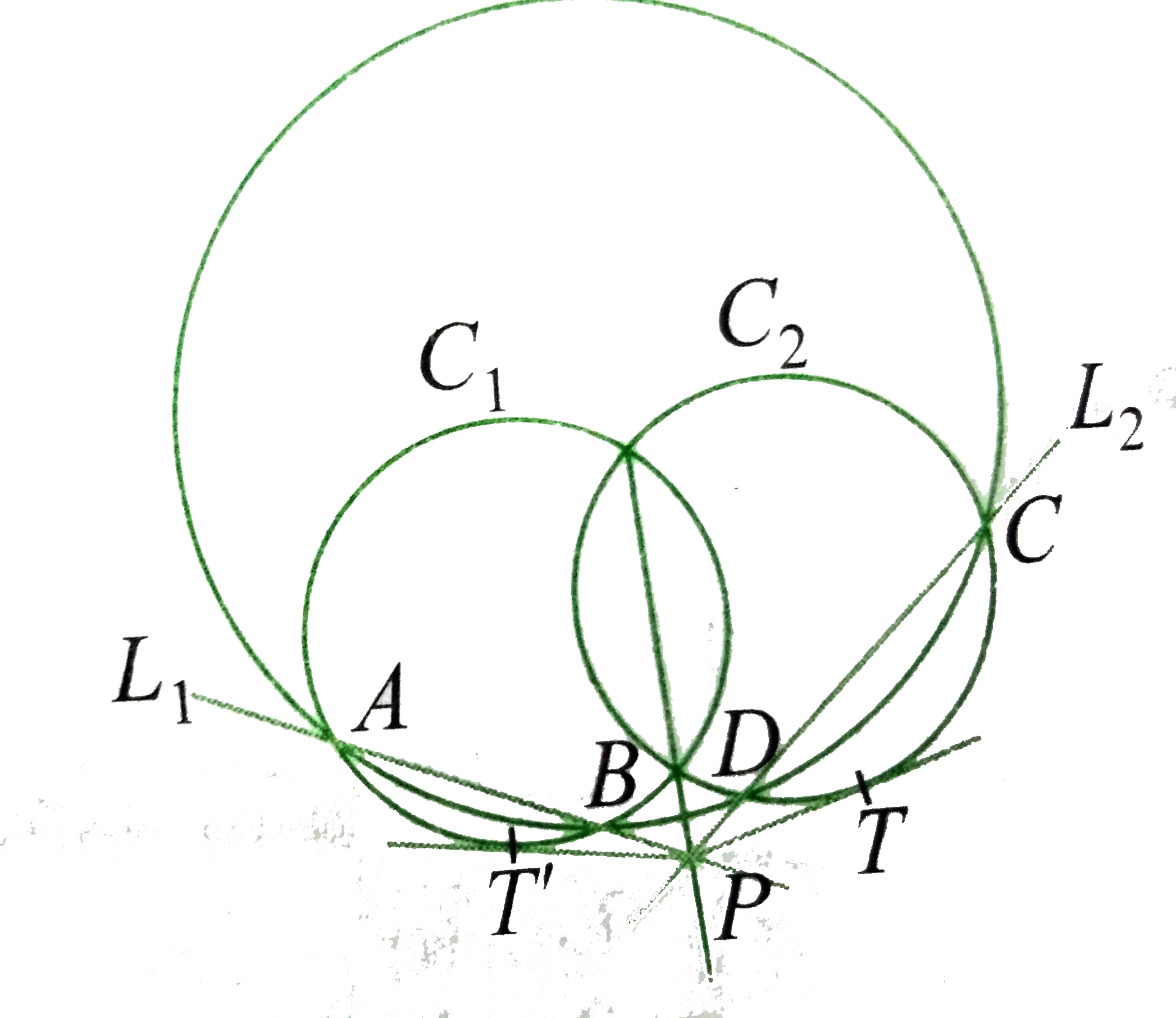
The given circles are
C1:x2+y2−4=0
and C2:x2+y2−4x−2y+1=0
The given lines are
L1:x+2y+a=0
and L2:12x−6y−41=0
If A,B,C, and D are concyclic , then
PA.PB=PC.PD
or PT2=PT'2
Therefore, point P will lie on the radical axis of circles C1 and C2 i.e., L3:4x+2y−5=0
Now, lines L1,L2, and L3 are concurrent. Therefore,
∣∣
∣∣42−512a12−6−41∣∣
∣∣0 or a=2
Also , for this value of a, the line meets circle (1) at two distinct points.
|
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE
6 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Matrix)
1 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
Knowledge Check
The line y=mx+c intersects the circle x2+y2=a2 in two distinct real points if
A−a√a+m2<cBc<a√1+m2C−c√1+m2<aDa<c√1+m2The line x+2y+a=0 intersects the circle x2+y2−4=0 at two distinct points A and B. Another line 12x-6y-41=0 intersects the circle x2+y2−4x−2y+1=0 at two distincts points C and D.
The value of 'a' so that the line x+2y+a=0 intersects the circle x2+y2−4=0 at two distinct points A and B isA−2√5<a<2√5B0<a<2√5C−√5<a<√5D0<a<√5The circle x2+y2=4x+8y+5 intersects the line 3x−4y=m at two distinct points if
A−35<m<15B15<m<65C35<m<85D−85<m<−35
A line parallel to the line x−3y=2 touches the circle x2+y2−4x+2y−5=0 at the point
View SolutionIf the line y=mx−(m−1) cuts the circle x2+y2=4 at two real and distinct points then
View SolutionThe line L:12x−16y−5=0 intersects the circle S:4x2+4y2−8x−16y−5=0 at A and B. If tangentsdrawn at the points A and B meet at C, then the correct option(s) is/are
View SolutionThe circle x2+y2=4x+8y+5 intersects the line 3x−4y=m at two distinct points if
View SolutionThe line 2x+y=3 intersects the ellipse 4x2+y2=5 at two points. The point of intersection of the tangents to the ellipse at these point is
View Solution
