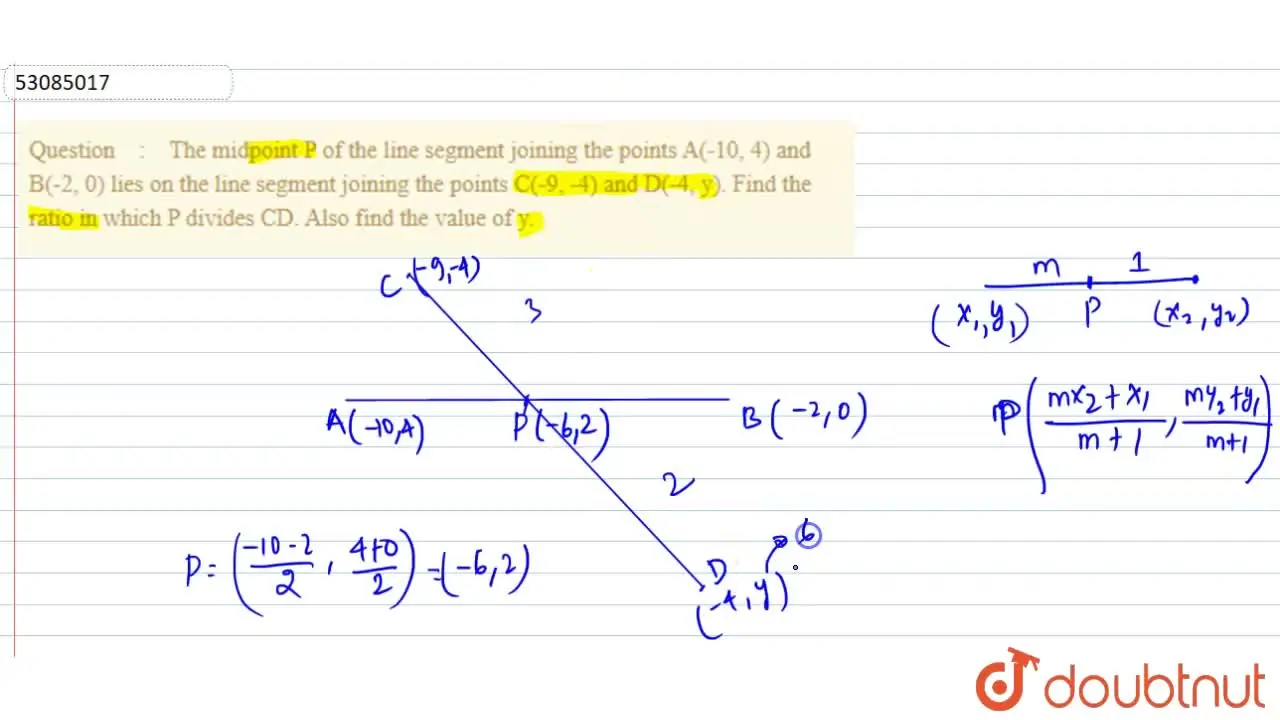
The midpoint P of the line segment joining the points A(-10, 4) and B(-2, 0) lies on the line segment joining the points C(-9, -4) and D(-4, y). Find the ratio in which P divides CD. Also find the value of y.
The correct Answer is:3:2,y=6
The point P is P(−10−22,4+02),i.e.,(−6,2).
Let P(-6, 2) divide CD in the ratio k:1.
Then, −4k−9k+1=−6 and ky−4k+1=2
⇒−4k−9=−6k and ky−4=2k+2
⇒2k=3 and k(y−2)=6
⇒k=32 and (y−2)=6k=(6×23)=4
⇒k=32 and y=4+2=6.
Hence, the ratio is 3:2 and y = 6.
Related Playlists
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 6C24videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 6D17videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 6A34videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself10videos
CONSTRUCTIONS
- BOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)25videos
HEIGHTS AND DISTANCES
Similar Questions
The mid-point P of the line segment joining the points A(−10,4)andB(−22,0) lies on the line segment joining the points C(−9,−4)andD(−4,y). Find the ratio in which P divides CD. Also, find the value of y.
View SolutionIf the point P(12,y) lies on the line segment joining the points A(3, -5) and B(-7, -9) the find the ratio in which P divides AB. Also, find the value of y.
View SolutionThe point P(-4,2) lies on the line segment joining the points A(-4,6) and B (-4,-6).
View SolutionPoint (x,4) lies on the line segment joining the points A (-5, 8) and B (4,-10) Find the ratio in which point P divides the line segment AB. Also find the value of x?
View SolutionFind the midpoint of the line segment joining the points (2,−5)and(−2,9).
View SolutionIf point P(4,2) lies on the line segment joining the points A(2,1) and B(8,4) then
View SolutionFind the point P divides the line segment joining the points (-1,2) and (4,-5) in the ratio 3:2
View SolutionFind the midpoint of the line segment joining the points (-3,4) and (1, - 2).
View SolutionIf point P(4,2) lies on the line segment joining the points A(2,1) and B(8,4) then :
View SolutionShow that the point P(−4,2) lies on the line segment joining the points A(−4,6) and B(−4,−6).
View Solution