The number of students who take both the subjects mathematics and chemistry is 30. This represents 10% of the enrolment in mathematics and 12% of the enrolment in chemistry. How many students take at least one of these two subjects?
520
490
560
480
The correct Answer is:A
To solve the problem step by step, we will use the information provided about the number of students enrolled in mathematics and chemistry, as well as those taking both subjects.
Step 1: Identify the known values
We know:
- The number of students taking both subjects (Mathematics and Chemistry) is N(M∩C)=30.
- This represents 10% of the total enrollment in Mathematics.
- This represents 12% of the total enrollment in Chemistry.
Step 2: Calculate the total enrollment in Mathematics
Let N(M) be the total enrollment in Mathematics. According to the information given:
N(M∩C)=10% of N(M)
This can be expressed mathematically as:
30=0.10×N(M)
To find N(M), we rearrange the equation:
N(M)=300.10=300
Step 3: Calculate the total enrollment in Chemistry
Let N(C) be the total enrollment in Chemistry. According to the information given:
N(M∩C)=12% of N(C)
This can be expressed mathematically as:
30=0.12×N(C)
To find N(C), we rearrange the equation:
N(C)=300.12=250
Step 4: Calculate the total number of students taking at least one subject
We need to find the number of students taking at least one of the two subjects, which is given by the formula:
N(M∪C)=N(M)+N(C)−N(M∩C)
Substituting the values we found:
N(M∪C)=300+250−30
Calculating this gives:
N(M∪C)=520
Final Answer
The number of students who take at least one of these two subjects is 520.
---
We know:
- The number of students taking both subjects (Mathematics and Chemistry) is N(M∩C)=30.
- This represents 10% of the total enrollment in Mathematics.
- This represents 12% of the total enrollment in Chemistry.
Let N(M) be the total enrollment in Mathematics. According to the information given:
N(M∩C)=10% of N(M)
This can be expressed mathematically as:
30=0.10×N(M)
To find N(M), we rearrange the equation:
N(M)=300.10=300
Let N(C) be the total enrollment in Chemistry. According to the information given:
N(M∩C)=12% of N(C)
This can be expressed mathematically as:
30=0.12×N(C)
To find N(C), we rearrange the equation:
N(C)=300.12=250
We need to find the number of students taking at least one of the two subjects, which is given by the formula:
N(M∪C)=N(M)+N(C)−N(M∩C)
Substituting the values we found:
N(M∪C)=300+250−30
Calculating this gives:
N(M∪C)=520
The number of students who take at least one of these two subjects is 520.
Topper's Solved these Questions
- BOOK - DISHA PUBLICATIONCHAPTER - SETSEXERCISE - EXERCISE-1 : CONCEPT BUILDER57videos
SETS
- BOOK - DISHA PUBLICATIONCHAPTER - SEQUENCES AND SERIES EXERCISE - Exercise -2 : Concept Applicator30videos
SEQUENCES AND SERIES
- BOOK - DISHA PUBLICATIONCHAPTER - STRAIGHT LINES AND PAIR OF STRAIGHT LINESEXERCISE - EXERCISE 2: CONCEPT APPLICATOR30videos
STRAIGHT LINES AND PAIR OF STRAIGHT LINES
Similar Questions
In a class of 80 students, 55 students passed in mathematics and 60 passed in chemistry. 10 stu-dents failed in both mathematics and chemistry.
(i) How many students passed in both the subjects ?
(ii) How many students passed in exactly one of the two subjects ?View SolutionIn a class of 80 students, 55 students passed in mathematics and 60 passed in chemistry. 10 stu-dents failed in both mathematics and chemistry.
(i) How many students passed in both the subjects ?
(ii) How many students passed in exactly one of the two subjects ?View Solution
Knowledge Check
- Question 1 - Select One
In a class of 175 students the following data shows the number of students opting one or more subjects Mathematics 100, physics 70, chemistry 28, physics and Chemistry 23, mathematics physics and chemistry 18. how many students have offered mathematics alone ?
A35B48C60D22 - Question 2 - Select One
In a class of 55 students, the number of students studying different subjects are 23 in Mathematics, 24 in Physics,19 in Chemistry, 12 in Mathematics and Physics, 9 in Mathematics and Chemistry, 7 in Physics and Chemistry and 4 in all the three subjects. The number of students who have taken exactly one subject is
A6B9C7D22 - Question 3 - Select One
In a class of 75 students, the number of students studying different Subjects are 43 in Mathematics 44 in Physics, 39 in Chemistry, 32 in Mathematics and Physics, 29 in Mathematics and Chemistry 27 in Physics and Chemistry and 24 in all three subjects. The number of students who have taken exactly one subject is
A26B29C27D22
A class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
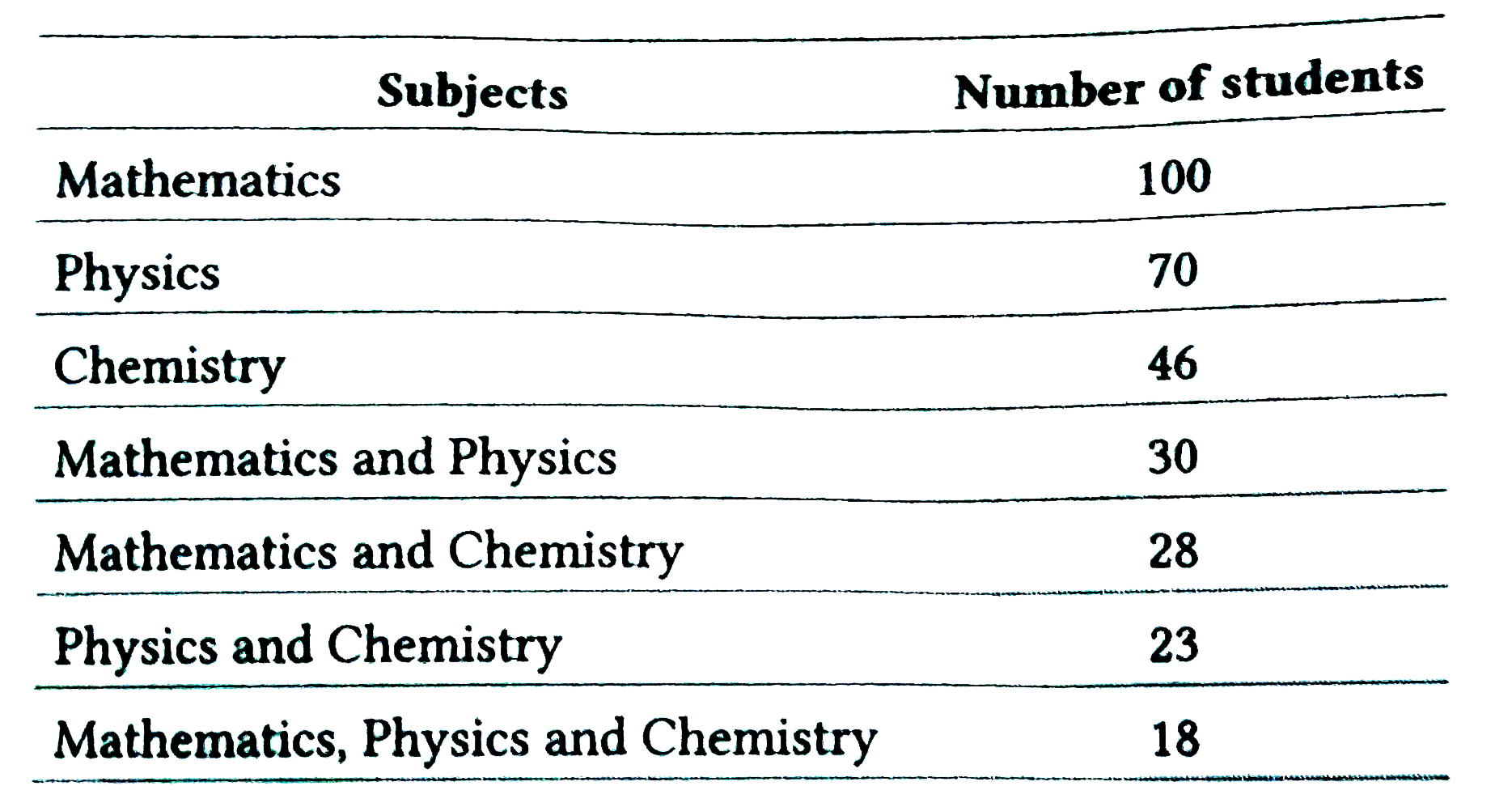
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?View SolutionIn a class of 80 students, 55 students passed in mathematics and 60 passed in chemistry. 10 stu-dents failed in both mathematics and chemistry.
(i) How many students passed in both the subjects ?
(ii) How many students passed in exactly one of the two subjects ?View SolutionA class has 175 students, The following description gives the number of students studying one or more of the subjects in this class, Mathematics 100, physics 70, chemistry 46, Mathematics and physics 30, mathematics and chemistry 28, physics and chemistry 23, mathematics, physics and chemistry alone 18,
(i) how many students are enrolled in mathematics alone, physics alone and children alone,
(ii) the number of students who have not offered any of these subjects.View SolutionIn a class of 55 students, the number of students studying different subjects are 23 in Mathematics, 24 in Physics, 19 in Chemistry, 12 in Mathematics and Physics, 9 in Mathematics and Chemistry, 7 in Physics and Chemistry and 4 in all the three subjects. Find the number of students who have taken exactly one subject.
View SolutionIn a class, the number of students studying different subjects are 23 in Mathematics, 24 in Physics, 19 in Chemistry, 12 in Mathematics and Physics, 9 in Mathematics and Chemistry, 7 in Physics and Chemistry and 4 in all the three subjects. The number of students who have taken exactly one subject is
View Solution

