The order and degree of the differential equation d2ydx2=(1+(dydx)2)32 areSolution in Tamil
2,2
2,1
1,2
2,3
The correct Answer is:A
Step by step video, text & image solution for The order and degree of the differential equation (d^(2)y)/(dx^(2))=(1+((dy)/(dx))^(2))^(3/2) are by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - CONTINUOUS EVALUATION (ASSIGNMENT)1videos
DIFFERENTIAL EQUATIONS
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - EXERCISE - HYPERBOLA9videos
CONIC SECTIONS
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - INTEGRALS EXERCISE - OBJECTIVE TYPE QUESTIONS34videos
INTEGRALS
Similar Questions
Complete the following tables
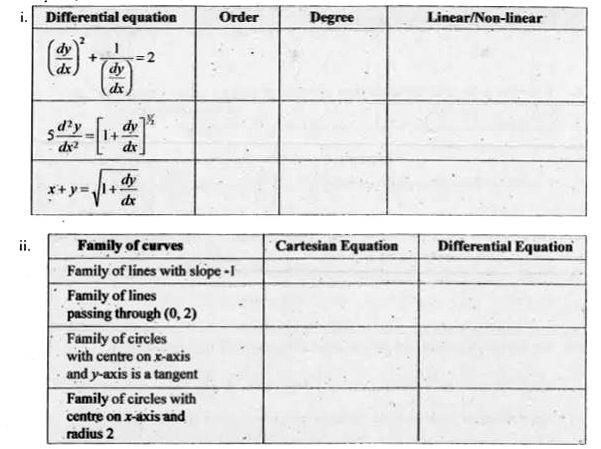 01:36View Solution
01:36View SolutionThe vertices of the hyperbola 9x2−16y2=144
01:08View Solution∫ealogxdx is equal to
01:55View SolutionOrder and degree of the differential equation d2ydx2={y+(dydx)2}1/4 are
02:02View SolutionThe order and degree of the differential equation d2ydx2=√1+(dydx)3, is
01:35View SolutionThe order and degree of the differential equation d2ydx2=3√1−(dydx)4 are respectively
01:58View Solutiond2ydx2+1(dydx)2=y . Find order and degree of differential equation.
02:41View Solutiond2ydx2=[1+dydx]3/2 . Find order and degree of differential equation.
02:08View SolutionOrder and degree of the differential equation d2ydx2=3√1+(dydx)3 respectively are
03:22View SolutionThe order and degree of the differential equation [1+(dydx)2]32=d2ydx2are respectively -
03:29View SolutionThe order and degree of the differential equation [(d2ydx2)+(dydx)2]12=d3ydx3 are
01:31View SolutionThe order and degree of the differential equation d2ydx2=(1+(dydx)2)32 are
03:05View SolutionOrder and degree of the differential equation
d2ydx2+(dydx)13+x=0 are respectively-01:21View SolutionThe degree and order of the differential equation :
2d2ydx2−3dydx+y=0 is :01:12View SolutionThe order and degree of the differential equation [1+(dydx)5]13=d2ydx2 are respectively
02:27View Solution

