The parabola $y^{2} = 4 x + 1$ divides the disc $x^{2} + y^{2} \leq 1$ into two regions with areas $A_{1}$ and $A_{2}$ . Then $| A_{1} - A_{2} |$ equal

A

$\frac{1}{3}$

B

$\frac{2}{3}$

C

$\frac{π}{4}$

D

$\frac{π}{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

$A_{1} = 2 \int_{- 1 / 4}^{0} \sqrt{4 x + 1} d x + 2 \int_{0}^{1} \sqrt{1 - x^{2}} d x$

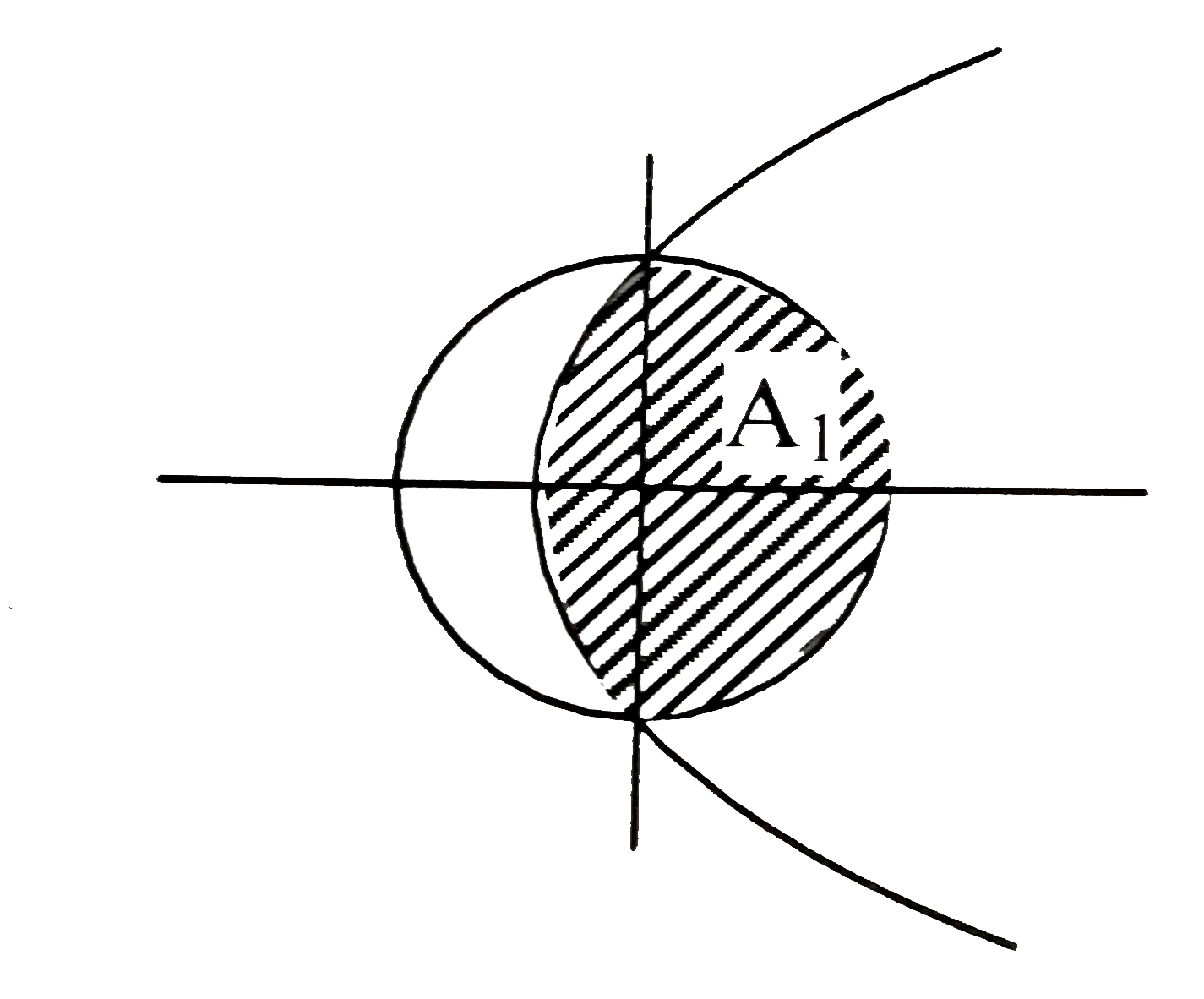
Solve $A_{1} = \frac{1}{3} + \frac{π}{2}$
$A_{2} = π {(1)}^{2} - A_{1} = \frac{π}{2} - \frac{1}{3}$
$| A_{1} - A_{2} | = \frac{2}{3}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The line $x = \frac{π}{4}$ divides the area of the region bounded by y = sin x, y = cos x and X-axis $(0 \leq x \leq \frac{π}{2})$ into two regions of areas $A_{1}$ and $A_{2}$ . Then, $A_{1} : A_{2}$ equals
A $4 : 1$
B $3 : 1$
C $2 : 1$
D $1 : 1$
Question 2 - Select One
If $A_{1}, A_{2}$ are between two numbers, then $\frac{A_{1} + A_{2}}{H_{1} + H_{2}}$ is equal to
A $\frac{H_{1} H_{2}}{G_{1} G_{2}}$
B $\frac{G_{1} G_{2}}{H_{1} H_{2}}$
C $\frac{H_{1} H_{2}}{A_{1} A_{2}}$
D $\frac{G_{1} G_{2}}{A_{1} A_{2}}$
Question 3 - Select One
Let points $A_{1}, A_{2} and A_{3}$ lie on the parabola $y^{2} = 8 x .$ If $△ A_{1} A_{2} A_{3}$ is an equilateral triangle and normals at points $A_{1}, A_{2} and A_{3}$ on this parabola meet at the point (h, 0). Then the value of h I s
A24
B26
C38
D28

KVPY PREVIOUS YEAR-KVPY-Part A - Mathematics

Home
Ask Doubt
Profile