The perimeter of a is and one side is . Then remaining sides of must be greater than : (a) (b) (c) (d)
8 cm
9 cm
12 cm
4 cm
The correct Answer is:D
To solve the problem, we need to find the minimum value of the remaining sides of triangle ABC given that the perimeter is 48 cm and one side is 20 cm.
1. Identify the given information:
- Perimeter of triangle ABC = 48 cm
- One side (let's say side A) = 20 cm
2. Set up the equation for the perimeter:
- Let the other two sides be B and C.
- According to the perimeter, we have:
A+B+C=48
- Substituting A = 20 cm:
20+B+C=48
3. Solve for B + C:
- Rearranging the equation gives:
B+C=48−20=28
4. Apply the triangle inequality:
- The triangle inequality states that the sum of the lengths of any two sides must be greater than the length of the third side. Therefore, we have:
- A+B>C
- A+C>B
- B+C>A
5. Substituting A = 20 cm into the inequalities:
- From A+B>C:
20+B>C(1)
- From A+C>B:
20+C>B(2)
- From B+C>A:
B+C>20(3)
- We already know from step 3 that B+C=28, so:
28>20(This is always true)
6. Rearranging inequalities (1) and (2):
- From inequality (1):
C<20+B
- From inequality (2):
B<20+C
7. Express C in terms of B:
- From B+C=28, we can express C as:
C=28−B
- Substitute this into inequality (1):
28−B<20+B
- Rearranging gives:
28−20<2B⟹8<2B⟹B>4
8. Express B in terms of C:
- Similarly, substitute into inequality (2):
B<20+(28−B)
- Rearranging gives:
B<48−B⟹2B<48⟹B<24
9. Conclusion:
- From the inequalities derived:
- B>4
- B<24
- Since B and C are interchangeable, we can conclude that both remaining sides must be greater than 4 cm.
Final Answer:
The remaining sides of triangle ABC must be greater than 4 cm.
- Perimeter of triangle ABC = 48 cm
- One side (let's say side A) = 20 cm
- Let the other two sides be B and C.
- According to the perimeter, we have:
A+B+C=48
- Substituting A = 20 cm:
20+B+C=48
- Rearranging the equation gives:
B+C=48−20=28
- The triangle inequality states that the sum of the lengths of any two sides must be greater than the length of the third side. Therefore, we have:
- A+B>C
- A+C>B
- B+C>A
- From A+B>C:
20+B>C(1)
- From A+C>B:
20+C>B(2)
- From B+C>A:
B+C>20(3)
- We already know from step 3 that B+C=28, so:
28>20(This is always true)
- From inequality (1):
C<20+B
- From inequality (2):
B<20+C
- From B+C=28, we can express C as:
C=28−B
- Substitute this into inequality (1):
28−B<20+B
- Rearranging gives:
28−20<2B⟹8<2B⟹B>4
- Similarly, substitute into inequality (2):
B<20+(28−B)
- Rearranging gives:
B<48−B⟹2B<48⟹B<24
- From the inequalities derived:
- B>4
- B<24
- Since B and C are interchangeable, we can conclude that both remaining sides must be greater than 4 cm.
The remaining sides of triangle ABC must be greater than 4 cm.
Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - VK JAISWAL ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-2 : One or More than One Answer is/are Correct
14 Videos
- SOLUTION OF TRIANGLESBOOK - VK JAISWAL ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-3 : Comprehension Type Problems
12 Videos
- SEQUENCE AND SERIES BOOK - VK JAISWAL ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
21 Videos
- STRAIGHT LINES BOOK - VK JAISWAL ENGLISHCHAPTER - STRAIGHT LINES EXERCISE - Exercise-5 : Subjective Type Problems
10 Videos
Similar Questions
The perimeter of a parallelogram is 70cm. If one side measures 20 cm, find the measure of its adjacent side.
View SolutionThe perimeter of a parallelogram is 64 cm. If one side measures 18 cm, find the measure of its adjacent side
View SolutionFind the perimeter of ΔABC, if AP=12 cm
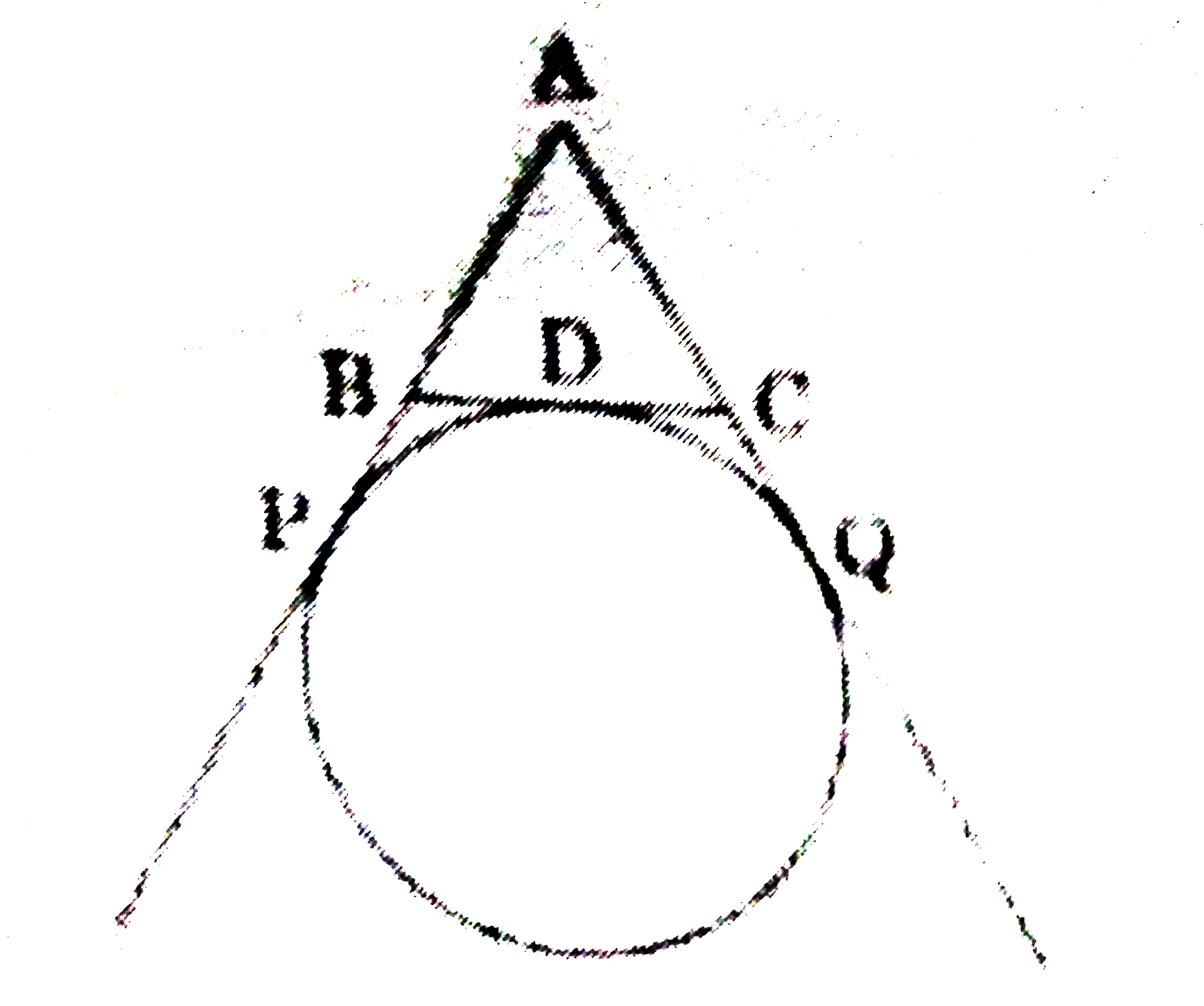
View SolutionThe hypotenuse of a right triangle is 26 cm long. If one of the remaining two sides is 10 cm long, the length of the other side is (a) 25 cm (b) 23 cm (c) 24 cm (d) 22 cm
View SolutionFind the perimeter of an equilateral triangle with side 9cm.
View SolutionThe perimeter of a triangle is 16 cm. One ofthe sides is of length 6 cm. If the area of thetriangle is 12 sq. cm, then the triangle is
View SolutionThe length of a rectangle is 8 cm and its area is 48 cm2. The perimeter of the rectangle is
(a)14 cm
(b) 24 cm
(c)12 cm
(d) 28 cm
View SolutionThe perimeter of a triangle is 30cm. Two sides are 12cm and 10cm. What is the length of the third side of the triangle?
View SolutionIn Figure, the perimeter of ABC is (a) 30cm (b) 60cm (c) 45cm (d) 15cm
View SolutionFind the perimeter of a triangle whose sides are 7 cm, 5.4 cm and 10.2 cm long,
View Solution
