Question

The point (2,1) , translated parallel to the line $x-y=3$ by the distance of 4 units. If this new position $A\text{'}$ is in the third quadrant, then the coordinates of $A\text{'}$ are-

Options

A. `(2+2sqrt(2),1+2sqrt(2))`
B. `(-2+sqrt(2),-1-2sqrt(2))`
C. `(2-2sqrt(2),1-2sqrt(2))`
D. none of these

Answer

C

Explanation

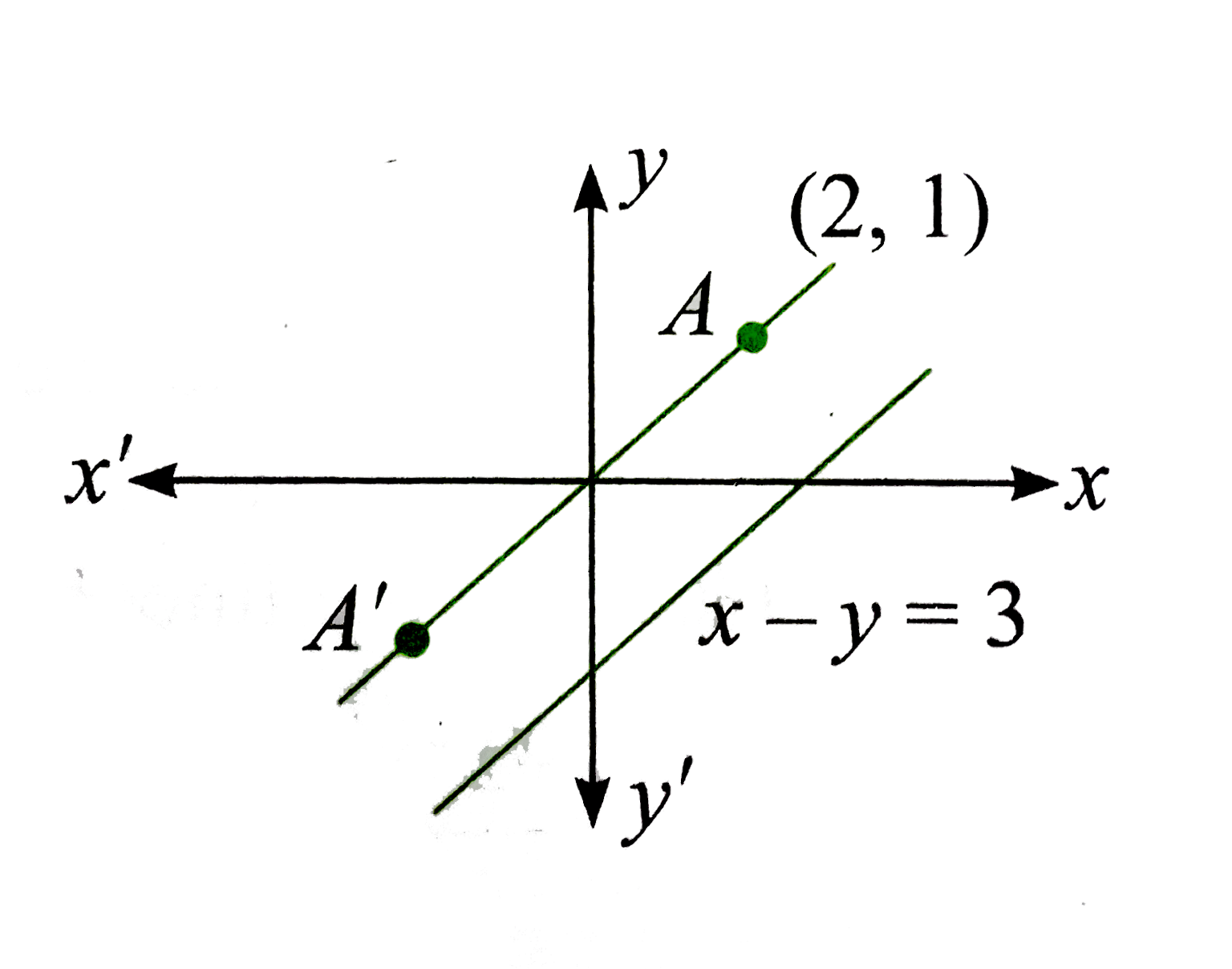
Since the poinnt A(2,1) is translated parallel to x-y=3, A'A has the same slope as that of x-y=3. Therefore, A'A passes through (2,1) and has slope 1. Here, `"tan" theta=1 "or cos" theta = 1sqrt(2), "sin" theta = 1sqrt(2).`
Thus, the equation of AA' is
`(x-2)/("cos"(pi//4)) = (y-1)/("sin"(pi//4))`
Since AA' =4, the coordinates of A' are given by
`(x-2)/("cos"(pi//4)) = (y-1)/("sin"(pi//4))=-4`
`"or " x=2-4"cos"(pi)/(4),y=1-4"sin"(pi)/(4)`
`"or " x=2-2sqrt(2),y=1-2sqrt(2)`
Hence, the coordinates of A' are `(2-2sqrt(2), 1-2sqrt(2)).`