The set of all values of x satisfying the inequations (x−1)3(x+1)<0 is
(−1,1)
(−∞,−1)
[−1,1]
(−1,∞)
The correct Answer is:A
We have,
(x−1)3(x+1)<0
⇒(x−1)2(x−1)(x+1)>0
⇒(x−1)(x+1)<0 [∵(x−1)2≥0for all x∈R]
⇒−1<x<1⇒x∈(−1,1)
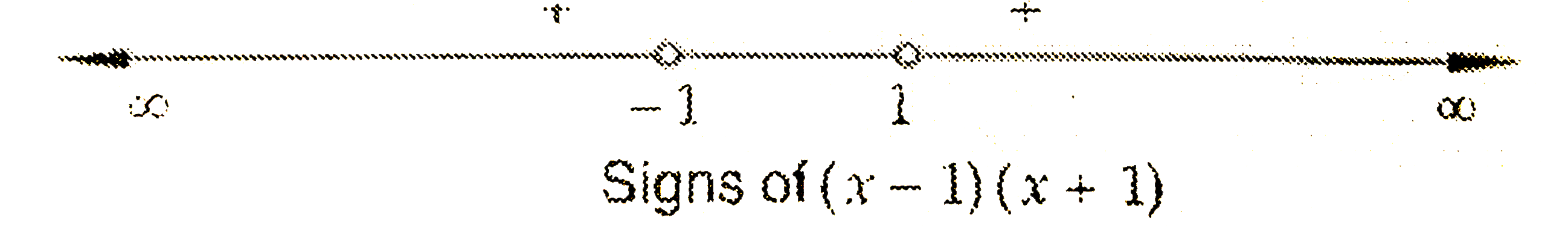
Topper's Solved these Questions
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Section I - Solved Mcqs20videos
ALGEBRAIC INEQUATIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise39videos
ALGEBRAIC INEQUATIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRA OF VECTORSEXERCISE - Chapter Test30videos
ALGEBRA OF VECTORS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test60videos
AREAS OF BOUNDED REGIONS
Similar Questions
The set of all values of x satisfying the inequations |x−1|≤5 and |x|≥2, is
View SolutionThe set of real values of x satisfying the inequality ∣∣x2+x−6∣∣<6, is
View SolutionThe set of values of x∈R satisfying the inequality x2−4x−21≤0 is
View SolutionThe set of all real numbers satisfying the inequation
2x+2|x|>2√2, isView SolutionThe set of values of x in (−π,π) satisfying the inequation |4sinx−1|<√5, is
View SolutionFind the set of all real values of x satisfying the inequality sec−1x>tan−1x.
View SolutionThe set of real values of x satisfying the inequality logx+1x(log2(x−1x+2))>0, is equal to
View SolutionSolve the inequation: x−1x−3<1
View SolutionThe set of real values of x satisfying ||x−1|−1|≤1, is
View SolutionNumber of intergral value of x satisfying the inequality x2+6x−7|x+4|<0 is :
View Solution

