The shape of a garden is rectangular in the middle and semi circular at the ends as shown in the diagram. Find the area and the perimeter of this garden [Length of rectangle is 20−(35+35) metres].
129.5,47
129.5,48
127.7,49
189.5,50
The correct Answer is:B
To solve the problem of finding the area and perimeter of the garden, which is rectangular in the middle and semi-circular at the ends, follow these steps:
Step 1: Determine the dimensions of the rectangle
Given that the total length of the garden is 20−(35+35) metres, we can simplify this:
20−(35+35)=20−70=−50 metres (which doesn't make sense)
However, based on the context, we can assume the length of the rectangle is 20 metres, and the diameter of the semicircles is 7 metres. Therefore, the radius r of the semicircles is:
r=72=3.5 metres
The length of the rectangle can be calculated as follows:
Length of rectangle=20−Diameter of semicircles
Length of rectangle=20−7=13 metres
Step 2: Calculate the area of the garden
The area of the garden consists of the area of the rectangle and the area of the two semicircles.
1. Area of the rectangle:
Area of rectangle=Length×Breadth=13×7=91 square metres
2. Area of the semicircles:
The area of one semicircle is given by the formula:
Area of semicircle=12πr2
Therefore, the area of two semicircles is:
Area of two semicircles=2×12πr2=πr2
Substituting r=3.5:
Area of two semicircles=π(72)2=π×494=49π4
Using π≈227:
Area of two semicircles=49×224×7=107828=38.5 square metres
3. Total area of the garden:
Total Area=Area of rectangle+Area of two semicircles
Total Area=91+38.5=129.5 square metres
Step 3: Calculate the perimeter of the garden
The perimeter consists of the lengths of the two semicircles and the two lengths of the rectangle.
1. Perimeter of the semicircles:
The perimeter of one semicircle is:
Perimeter of semicircle=πr
Therefore, the perimeter of two semicircles is:
Perimeter of two semicircles=2×12πd=πd
where d=7:
Perimeter of two semicircles=π×7
Substituting π≈227:
Perimeter of two semicircles=7×227=22 metres
2. Perimeter of the rectangle:
The perimeter of the rectangle is:
Perimeter of rectangle=2×Length=2×13=26 metres
3. Total perimeter of the garden:
Total Perimeter=Perimeter of two semicircles+Perimeter of rectangle
Total Perimeter=22+26=48 metres
Final Answers:
- Area of the garden: 129.5 square metres
- Perimeter of the garden: 48 metres
Given that the total length of the garden is 20−(35+35) metres, we can simplify this:
Length of rectangle=20−7=13 metres
The area of the garden consists of the area of the rectangle and the area of the two semicircles.
Area of rectangle=Length×Breadth=13×7=91 square metres
The area of one semicircle is given by the formula:
Area of semicircle=12πr2
Therefore, the area of two semicircles is:
Area of two semicircles=2×12πr2=πr2
Substituting r=3.5:
Area of two semicircles=π(72)2=π×494=49π4
Using π≈227:
Area of two semicircles=49×224×7=107828=38.5 square metres
Total Area=Area of rectangle+Area of two semicircles
Total Area=91+38.5=129.5 square metres
The perimeter consists of the lengths of the two semicircles and the two lengths of the rectangle.
The perimeter of one semicircle is:
Perimeter of semicircle=πr
Therefore, the perimeter of two semicircles is:
Perimeter of two semicircles=2×12πd=πd
where d=7:
Perimeter of two semicircles=π×7
Substituting π≈227:
Perimeter of two semicircles=7×227=22 metres
The perimeter of the rectangle is:
Perimeter of rectangle=2×Length=2×13=26 metres
Total Perimeter=Perimeter of two semicircles+Perimeter of rectangle
Total Perimeter=22+26=48 metres
- Area of the garden: 129.5 square metres
- Perimeter of the garden: 48 metres
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - MENSURATIONEXERCISE - SOLVED EXAMPLES11videos
MENSURATION
- BOOK - NCERT ENGLISHCHAPTER - MENSURATIONEXERCISE - EXERCISE 11.48videos
MENSURATION
- BOOK - NCERT ENGLISHCHAPTER - MENSURATIONEXERCISE - EXERCISE 11.211videos
MENSURATION
- BOOK - NCERT ENGLISHCHAPTER - LINEAR EQUATIONS IN ONE VARIABLEEXERCISE - EXERCISE 2.39videos
LINEAR EQUATIONS IN ONE VARIABLE
- BOOK - NCERT ENGLISHCHAPTER - PLAYING WITH NUMBERSEXERCISE - EXERCISE 16.110videos
PLAYING WITH NUMBERS
Similar Questions
The shape of a garden is rectangular in the middle and semi circular at the ends as shown in the diagram. Find the area and the perimeter of this garden [Length of rectangle is
View SolutionFind the area of the flower bed (with semi-circular ends) shown in figure.
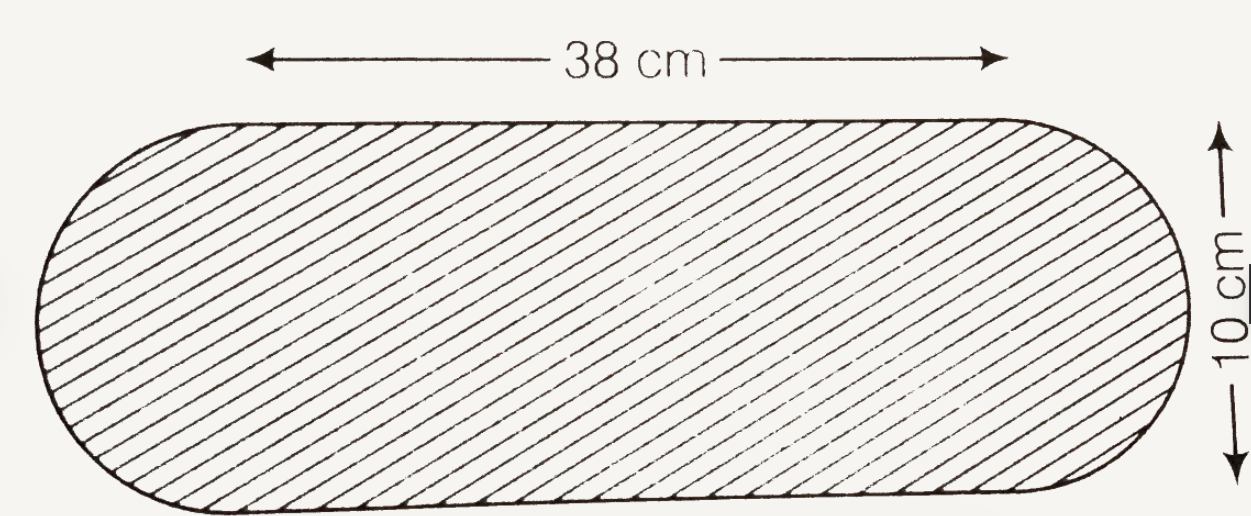 View Solution
View SolutionThe length of a rectangle is 16 cm and its perimeter is equal to the perimeter of a square with side 12.5 cm. Find the area of the rectangle.
View SolutionThe length of a rectangle is reduced to its one-third and its breadth is increased three times. Find the new perimeter and the new area.
View SolutionFind the perimeter of the following shape.
A square of side 35 mView SolutionIf a rectangle has breadth 11 m and area 264 sq. m, what is the length of the rectangle? Also, find the perimeter of the rectangle.
View SolutionThere is a path of uniform width 7 m round and outside a circular garden of diameter 210 m. Find the area of the path.
View SolutionThe area of a square and a rectangle are equal.If the side of the square is 40 cm and the breadth of the rectangle is 25 cm,find the length of the rectangle. Also,find the perimeter of the rectangle.
View SolutionA rectangular field is of length 60 m and breadth 35 m. Find the area of the field.
View SolutionA square and a rectangle have equal area. If side of a square is 24 cm and the length of the rectangle is 32 cm , find
the perimeter of the rectangle.View Solution

