The shape of the top surface of a table is a trapezium. Find its area if its parallel sides are 1m and 1.2m and perpendicular distance between them is 0.8m.
To find the area of the trapezium-shaped top surface of the table, we can follow these steps:
Step 1: Identify the lengths of the parallel sides and the height
The lengths of the parallel sides (bases) of the trapezium are given as:
- Base 1 (b1) = 1 m
- Base 2 (b2) = 1.2 m
The perpendicular distance (height) between the parallel sides is:
- Height (h) = 0.8 m
Step 2: Use the formula for the area of a trapezium
The formula for the area (A) of a trapezium is:
A=12×(b1+b2)×h
Step 3: Substitute the values into the formula
Now, we will substitute the values of b1, b2, and h into the formula:
A=12×(1+1.2)×0.8
Step 4: Calculate the sum of the parallel sides
First, calculate the sum of the parallel sides:
b1+b2=1+1.2=2.2
Step 5: Multiply by the height and then by 1/2
Now, substitute this sum back into the area formula:
A=12×2.2×0.8
Calculating this step-by-step:
1. Multiply 2.2 by 0.8:
2.2×0.8=1.76
2. Now, multiply by 12:
A=1.762=0.88
Step 6: State the final area
Thus, the area of the trapezium is:
A=0.88m2
The lengths of the parallel sides (bases) of the trapezium are given as:
- Base 1 (b1) = 1 m
- Base 2 (b2) = 1.2 m
- Height (h) = 0.8 m
The formula for the area (A) of a trapezium is:
A=12×(b1+b2)×h
Now, we will substitute the values of b1, b2, and h into the formula:
A=12×(1+1.2)×0.8
First, calculate the sum of the parallel sides:
b1+b2=1+1.2=2.2
Now, substitute this sum back into the area formula:
A=12×2.2×0.8
1. Multiply 2.2 by 0.8:
2.2×0.8=1.76
2. Now, multiply by 12:
A=1.762=0.88
Thus, the area of the trapezium is:
A=0.88m2
Topper's Solved these Questions
- MENSURATIONBOOK - NCERTCHAPTER - MENSURATIONEXERCISE - SOLVED EXAMPLES
11 Videos
- MENSURATIONBOOK - NCERTCHAPTER - MENSURATIONEXERCISE - EXERCISE 11.3
10 Videos
- LINEAR EQUATIONS IN ONE VARIABLEBOOK - NCERTCHAPTER - LINEAR EQUATIONS IN ONE VARIABLEEXERCISE - EXERCISE 2.3
9 Videos
- PLAYING WITH NUMBERSBOOK - NCERTCHAPTER - PLAYING WITH NUMBERSEXERCISE - SOLVED EXAMPLES
8 Videos
Similar Questions
The shape of the top surface of a table is a trapezium. Find its area if its parallel sides are 1m and 1.2m and perpendicular distance between them is 0.8m.
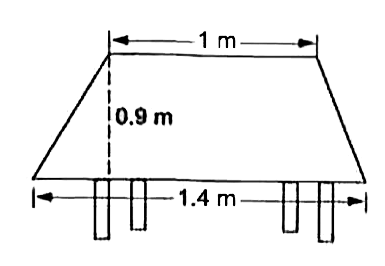
View SolutionThe shape of the surface of a table is trapezium. Its parallel sides are 1 m and 1.4 m and the perpendicular distance between them is 0.9 m. Find its area.
View Solution
Knowledge Check
Directions : In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct answer as
Assertion : The area of trapezium if its parallel sides are lm and 1.2 m and the perpendicular distance between them is 0.8 m is 0.98 m2.
Reason : Area of trapezium =12 sum of its parallel sides × heightAIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is true.
Find the area of a trapezium whose parallel sides are 24 cm and 20 cm and the distance between them is 15 cm.
View SolutionFind the area of a trapezium whose parallel sides are 57 cm and 33 cm and the distance between them is 13 cm.
View SolutionFind area of a trapezium whose parallel sides are 28cm and 14cm and the distance between them is 5cm..
View SolutionFind the area of a trapezium whose parallel sides are of lengths 10cm and 12cm and the distance between them is 4cm.
View SolutionThe area of a trapezium is 960 cm2. If the parallel sides are 34cm and 46cm, find the distance between them.
View SolutionFind the area of a trapezium whose parallel sides are 9 cm and 6cm respectively and the distance between these sides is 8 cm.
View SolutionFind the area of trapezium having length of parallel sides 25 m and 15 m .The distance between the parallel sides is 8 m.
View Solution
