The sides of two similar triangles are in the ratio 2 : 3 , then their areas are in the ratio ………… Solution in Tamil
The correct Answer is:B
Step by step video, text & image solution for The sides of two similar triangles are in the ratio 2 : 3 , then their areas are in the ratio ………… by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - ADDITIONAL QUESTIONS SOLVED (ANSWER THE FOLLOWING QUESTIONS)24videos
GEOMETRY
- BOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - THINKING CORNER2videos
GEOMETRY
- BOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - UNIT EXERCISE 410videos
GEOMETRY
- BOOK - FULL MARKSCHAPTER - DEPARTMENTAL MODEL PAPER EXERCISE - PART-IV3videos
DEPARTMENTAL MODEL PAPER
- BOOK - FULL MARKSCHAPTER - MENSURATION EXERCISE - ADDITIONAL QUESTIONS SOLVED (iii)8videos
MENSURATION
Similar Questions
The image of man height 1.8m , is of length 1.5 cm on the film of a camera . If the film is 3 cm from the camera, how far is the man from the camera ?
00:58View SolutionCan we draw two tangents parallel to each other on a circle ?
01:16View SolutionIn the figure, if BD⊥ACandCE⊥AB , prove that
(i)ΔAEC~ΔADB (ii)CAAB=CEDB
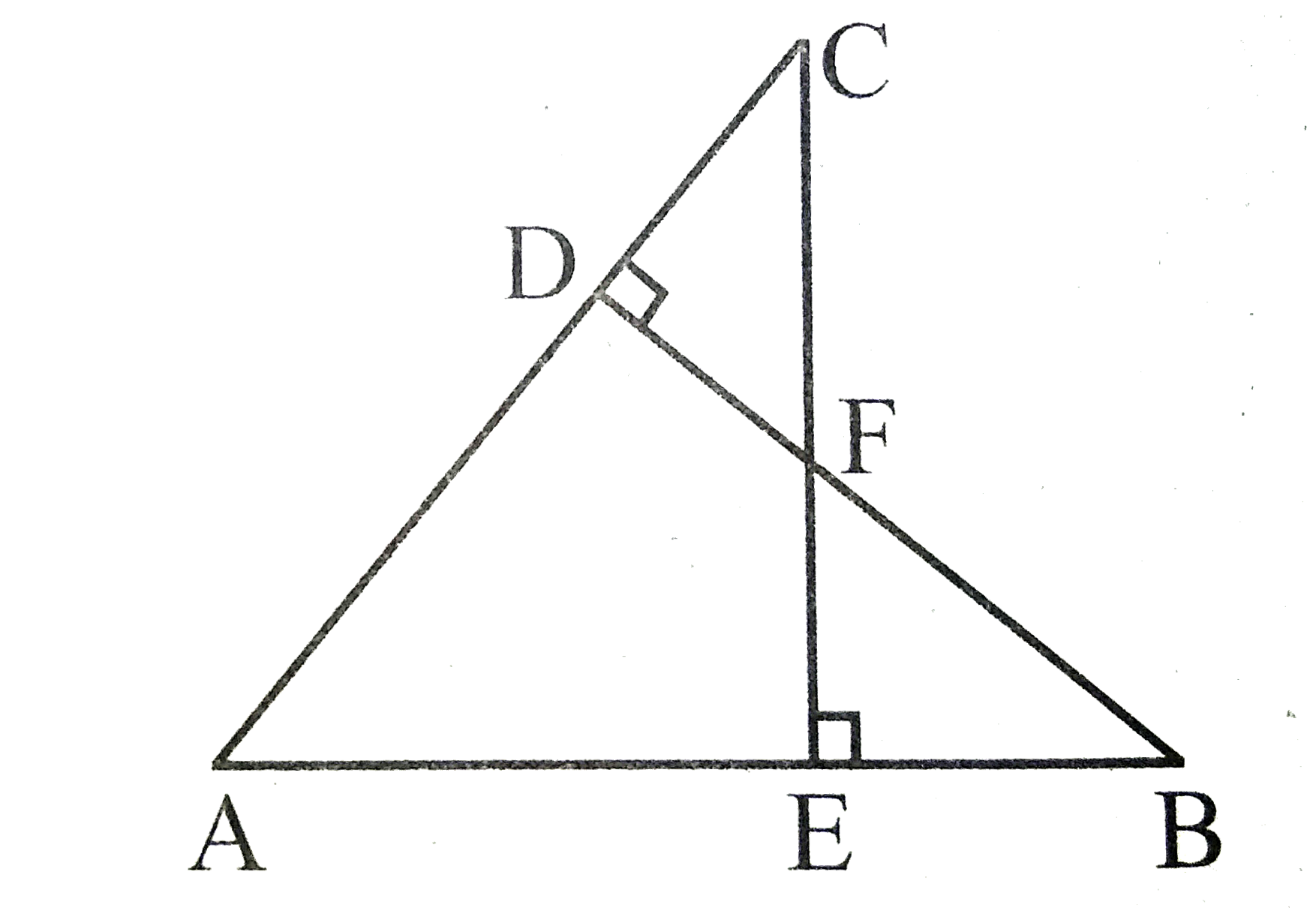 01:50View Solution
01:50View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
03:40View SolutionA vessel is in the form of a hemispherical bowl mounted by a hollow cylinder. The diameter is 14 cm and the height of the vessel is 13 cm. Find the capacity of the vessel.
01:17View SolutionThe sides of two similar triangles are in the ratio 2:3, then the areas of these triangles are in the ratio ________.
02:26View SolutionSides of two similar triangles are in the ratio 3:5, Areas of these triangles are in the ratio..
01:38View SolutionThe ratio of the corresponding sides of two similar triangles is 5:3. Then the ratio of their areas………..
01:43View SolutionIF the sides of two similar triangles are in the ratio 7:2 then the ratio of their areas is………..
02:01View SolutionSides of two similar triangles are in the ratio 4 : 9 Areas of these triangles are in the ratio
01:41View SolutionArea of two similar triangles are in the ratio of 5:3 then the ratio of their corresponding sides is :
View SolutionIf the ratio of the sides of two similar triangles is 3 : 5, then the ratio of their areas is :
01:27View SolutionIf the ratio of sides of two similar triangles is 2:5, then the ratio of their areas will be :
01:12View SolutionAreas of two similar triangles are in the ratio 64:121, then the sides of these triangles are in the ratio:
View SolutionThe sides of two similar triangles are in the ratio 3:7. The ratio of areas of these triangles will be :
03:30View Solution

