The triangle formed by the tangent to the curve $f (x) = x^{2} + b x - b$ at the point $(1, 1)$ and the coordinate axes, lies in the first quadrant. If its area is 2, then the value of $b$ is (a) $- 1$ (b) $3$ (c) $- 3$ (d) $1$

A

$- 1$

B

$- \frac{5}{2}$

C

$- 3$

D

1

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Since, tangent at (1,1) to the parabola forms a triangle with axis, which lie in the first quadrant, vertex lies to the ringt of x=1.
$∴ - \frac{b}{2} > 1 \Rightarrow b < - 2$

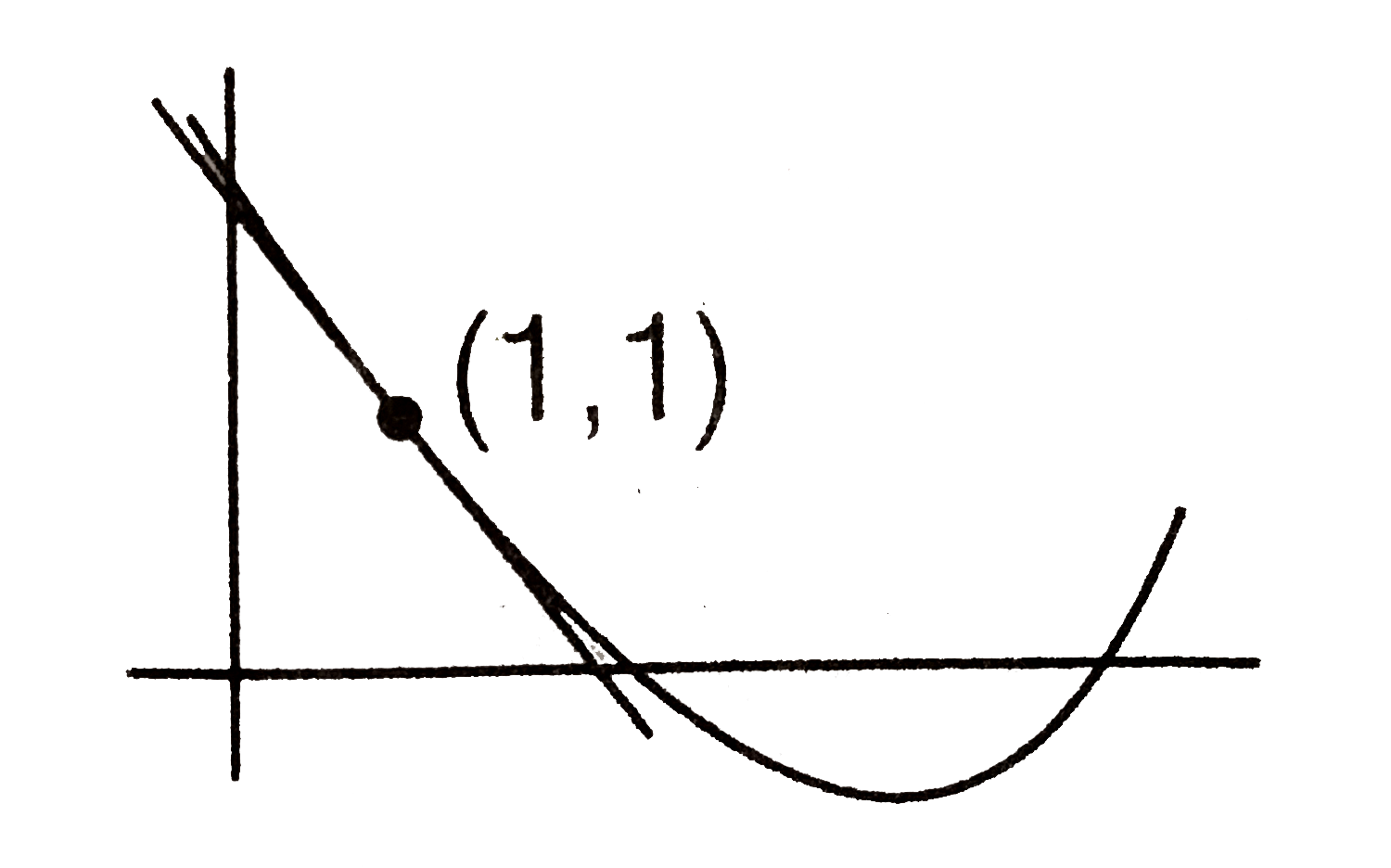
Equation of tangent at (1,1) is
$y - 1 = (b + 2) (x - 1)$
$∴$ x-intercept $= \frac{(b + 1)}{b + 2}$
y-intercept=-(b+1)
$∴$ Area of $Δ = - \frac{{(b + 1)}^{2}}{2 (b + 2)} = 2$
$\Rightarrow b^{2} + 6 b + 9 = 0$
$\Rightarrow b = - 3$ .

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
The triangle formed by the normal to the curve $f (x) = x^{2} - a x + 2 a$ at the point (2,4) and the coordinate axes lies in second quadrant, if its area is 2 sq units, then a can be
A2
B $17 / 4$
C5
DNone of these
Question 2 - Select One
The area of the triangle formed by the tangent at the point (a, b) to the circle $x^{2} + y^{2} = r^{2}$ and the coordinate axes, is
A $\frac{r^{4}}{2 a b}$
B $\frac{r^{4}}{2 | a b |}$
C $\frac{r^{4}}{a b}$
D $\frac{r^{4}}{| a b |}$
Question 3 - Select One
The area of the traingle formed by the coordinate axes and a tangent to the curve $x y = a^{2}$ at the point $(x_{1}, y_{1})$ is
A $\frac{a^{2} x_{1}}{y_{1}}$
B $\frac{a^{2} y_{1}}{x_{1}}$
C $2 a^{2}$
D $4 a^{2}$

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-PRACTICE SET 06-PAPER 2 (MATHEMATICS)

Home
Ask Doubt
Profile