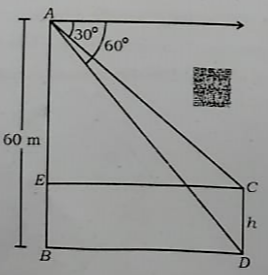
The two towers of heights x and y, standing on level ground, subtend angles of 30∘and60∘ respectively at the centre of the line joining their feet. Then, find x: ySolution in Gujrati
The correct Answer is:1:3
Step by step video, text & image solution for The two towers of heights x and y, standing on level ground, subtend angles of 30^(@) and 60^(@) respectively at the centre of the line joining their feet. Then, find x: y by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Practice Thoroughly7videos
SOME AAPLICATIONS OF TRIGONOMETRY
- BOOK - KUMAR PRAKASHANCHAPTER - REAL NUMBERS EXERCISE - TEST YOUR SKILLS26videos
REAL NUMBERS
- BOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - Objective Questions (True or false)5videos
STATISTICS
Similar Questions
A jet plane is at a vertical height of h. The angles of depression of two tanks on the ground in the same line with the plane are αandβ(α>β). Prove that the distance between the tanks is h(tanα−tanβ)tanαtanβ
02:12View SolutionShow that the square of any positive integer is of the form 4m or 4m + 1 for some integer m.
03:15View SolutionFor any frequency distribution, mean is always greater than mode.
04:54View SolutionThe tops of two towers of height x and y , standing on level ground, subtend angles of 30o and 60o respectively at the centre of the line joining their feet, then find x :y .
05:51View Solutionएक मीनार पर 10 मीटर ऊँचा झंडा खड़ा है। जमीन पर स्थित एक बिंदु से झंडे के पद और शीर्ष के उन्नयन कोण क्रमशः 30∘ तथा 60∘ है, मीनार के ऊँचाई ज्ञात करें ।
04:18View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60^@ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30^@ . The height of the tower is
02:48View SolutionIf two towers of height X and Y subtend angles of 30∘ and 60∘ respectively at the centre of the line joining their feet, then X:Y is equal to
04:21View SolutionA tower and a pole stand vertically on the same level ground. it is observed that the angles of depression of top and foot of the pole from the top of the tower of height 60m is 30∘ and 60∘ respectively. find the height of the pole.
 04:06View Solution
04:06View SolutionThese are two towers on a horizontal line. From the mid point of the line joining their feet, the tops of the towers appear at angles of elevation of 60∘ and 30∘ respectively. The first tower has a height of 100 m. The height of the second tower is
View SolutionA vertical tower subtends an angle of 60∘ at a point on the same level as the foot of the tower. On moving 100 m further in line with the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower is
03:10View SolutionThe angles of elevation of the top of two vertical towers as seen from the middle point of the line joining the feet of the towers are 60^(@) and 30^(@) respectively. The ratio of the height of the towers is
02:59View SolutionThe shadow of a tower standing on a level ground is x metres long when the sun's altitude is 30∘, while it is y metres long when the altitude is 60∘. If the height of the tower is 45.√33 meters, then x−y is :
02:31View SolutionA tower stands at the centre of a circular park .A and B are two points on the boundary of the park such that AB = (=a) subtends an angle of 60^@ at the foot of the tower ,and the angle of elevation of the top of the tower from A or B is 30^@ the height of hte tower is
02:35View SolutionThe tops of two towers of height x and y standing on a level ground subtend angles of 30∘ and 60∘ respectively at the centre of the line joining their feet. Find x:y
02:22View SolutionThe tops of two towers of height x and y , standing on level ground, subtend angles of 30∘ and 60∘ respectively at the centre of the line joining their feet, then find x :y .
03:58View Solution

