The value of 10∑n=1n is:Solution in Kannada
10
11
55
110
The correct Answer is:C
Step by step video, text & image solution for The value of sum_(n=1)^(10) n is: by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - OSWAAL PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - VERY SHORT ANSWER TYPE QUESTION8videos
ARITHMETIC PROGRESSIONS
- BOOK - OSWAAL PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - SHORT ANSWER TYPE QUESTION15videos
ARITHMETIC PROGRESSIONS
- BOOK - OSWAAL PUBLICATIONCHAPTER - AREAS RELATED TO CIRCLES EXERCISE - LONG ANSWER TYPE QUESTIONS - I20videos
AREAS RELATED TO CIRCLES
- BOOK - OSWAAL PUBLICATIONCHAPTER - CIRCLES EXERCISE - TEXTBOOK CORNER (EXERCISE 4.2)13videos
CIRCLES
Similar Questions
What is the common difference between the consecutive terms of an A.P.
07:41View SolutionHow many two digit numbers are divisible by 3?
03:00View SolutionIn the given figure, AOB is a sector of angle 60∘ of a circle with centre O and radius 17 cm . If AP ⊥ OB and AP = 15 cm find the area of the shaded region.
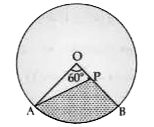 02:01View Solution
02:01View SolutionFrom a point Q ,the length of the tangent to a circle is 24 cm and the distacne to Q from the centre is 24.5 cm.The radius of the circle is
(a) 4.92 cm
(b)12 cm
©15 cm
(d)24.5 cm
02:31View SolutionIf the sum 10∑n=110∑m=1tan−1(mn)=kπ, find the value of k
01:46View Solutionsum_ (n = 1) ^ (oo) (5 ^ (n) -2 ^ (n)) / (10 ^ (n))
01:32View SolutionThe value of sum_(n=1)^(10)int xdx=
04:15View SolutionThe value of lim_(n rarr oo)(sum_(r=1)^(100)(n+r)^(10))/(n^(10)+10^(10)) is equal to
View SolutionThe value of 10∑n=0in equals
02:23View SolutionIf n∑r=1r√103n∑r=1r2,n∑r=1r3 are in G.P., then the value of n, is
04:37View SolutionThe value of 13∑i=1(nn+in−1) is
06:29View SolutionLet f(n)=n∑k=−n(cot−1(1k)−tan−1(k)) such that 10∑n=2(f(n)+f(n−1))=aπ then find the value of (a+1).
View SolutionFind the value of n∑i=1n∑i=1n∑k=11
08:38View SolutionFind the value of n∑i=1n∑i=1n∑k=1k
00:46View SolutionFind the values of n and ¯¯¯X in each of the following cases: (i) n∑i=1(x1−12)=−10 and n∑i=1(x1−3)=62 (ii) n∑i=1(x1−10)=30 and n∑i=1(x1−6)=150
05:55View Solution
