The values of x and y satisfying (x+3)(y−5)=xy+39 and (x−2)(y+3)=xy−40 respectively are:
6 and 8
6 and -8
−6 and 8
−6 and -8
The correct Answer is:C
To solve the equations (x+3)(y−5)=xy+39 and (x−2)(y+3)=xy−40, we will follow these steps:
Step 1: Expand the first equation
We start with the first equation:
(x+3)(y−5)=xy+39
Expanding the left side:
xy−5x+3y−15=xy+39
Step 2: Simplify the first equation
Now, we can cancel xy from both sides:
−5x+3y−15=39
Adding 15 to both sides gives:
−5x+3y=54(Equation 1)
Step 3: Expand the second equation
Now, let's look at the second equation:
(x−2)(y+3)=xy−40
Expanding the left side:
xy+3x−2y−6=xy−40
Step 4: Simplify the second equation
Cancelling xy from both sides:
3x−2y−6=−40
Adding 6 to both sides gives:
3x−2y=−34(Equation 2)
Step 5: Solve the system of equations
Now we have the two equations:
1. −5x+3y=54
2. 3x−2y=−34
We can solve these equations using the method of substitution or elimination. Here, we will use the elimination method.
Step 6: Multiply equations to align coefficients
To eliminate y, we can multiply Equation 1 by 2 and Equation 2 by 3:
2(−5x+3y)=2(54)⟹−10x+6y=108(Equation 3)
3(3x−2y)=3(−34)⟹9x−6y=−102(Equation 4)
Step 7: Add the equations
Now, we add Equation 3 and Equation 4:
(−10x+6y)+(9x−6y)=108−102
This simplifies to:
−x=6
Thus,
x=−6
Step 8: Substitute x back to find y
Now, substitute x=−6 back into Equation 1:
−5(−6)+3y=54
This simplifies to:
30+3y=54
Subtracting 30 from both sides gives:
3y=24
Thus,
y=8
Final Answer
The values of x and y are:
x=−6,y=8
We start with the first equation:
(x+3)(y−5)=xy+39
Expanding the left side:
xy−5x+3y−15=xy+39
Now, we can cancel xy from both sides:
−5x+3y−15=39
Adding 15 to both sides gives:
−5x+3y=54(Equation 1)
Now, let's look at the second equation:
(x−2)(y+3)=xy−40
Expanding the left side:
xy+3x−2y−6=xy−40
Cancelling xy from both sides:
3x−2y−6=−40
Adding 6 to both sides gives:
3x−2y=−34(Equation 2)
Now we have the two equations:
1. −5x+3y=54
2. 3x−2y=−34
To eliminate y, we can multiply Equation 1 by 2 and Equation 2 by 3:
2(−5x+3y)=2(54)⟹−10x+6y=108(Equation 3)
3(3x−2y)=3(−34)⟹9x−6y=−102(Equation 4)
Now, we add Equation 3 and Equation 4:
(−10x+6y)+(9x−6y)=108−102
This simplifies to:
−x=6
Thus,
x=−6
Now, substitute x=−6 back into Equation 1:
−5(−6)+3y=54
This simplifies to:
30+3y=54
Subtracting 30 from both sides gives:
3y=24
Thus,
y=8
The values of x and y are:
x=−6,y=8
Topper's Solved these Questions
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SIMULTANEOUS LINEAR EQUATIONSEXERCISE - SELF ASSESSMENT SHEET10videos
SIMULTANEOUS LINEAR EQUATIONS
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SIMULTANEOUS LINEAR EQUATIONSEXERCISE - SELF ASSESSMENT SHEET10videos
SIMULTANEOUS LINEAR EQUATIONS
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SIMPLE INTERESTEXERCISE - SELF ASSESSMENT SHEET-185videos
SIMPLE INTEREST
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Unit Test - 620videos
SOME APPLICATIONS OF TRIGONOMETRY
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the value of x:y, if 8x−10y3x+4y=45.
A55:29B36:17C33:14D16:11 - Question 2 - Select One or More
If x and y satisfy te equation xy−2x2−9x+3y−16=0 then
Anumber of ordered pair (x,y) is 4 where x,y∈ZBnumber of ordered paire (x,y) is 1 where x,y∈NCif x≥y,x,y∈N then number of ordered pair is zeroDif x≤y,x,y∈N then number of ordered pairs are two - Question 3 - Select One
Find the value of x:y, if 8x−10y3x+4y=45.
A55:29B36:17C33:14D16:11
Find the value of x and y which satisfy the following equations (x.y∈R) (i) (x+2y)+(2x−3y)i+4i=5
View SolutionWhat is the point on the xy-plane satisfying 5x+2y=7xy and 10x+3y=8xy ?
View SolutionThe number of distinct pairs (x,y) of the real number satisfying x=x3+y4andy=2xy is :
View SolutionIf x+y=60 and xy3 is maximum, then x and y respectively will be
View SolutionIn the figure above (no to scale), l∣∣m and also x:y=5:3. Find the measure of angles (x−40∘) and (y+40∘) respectively.
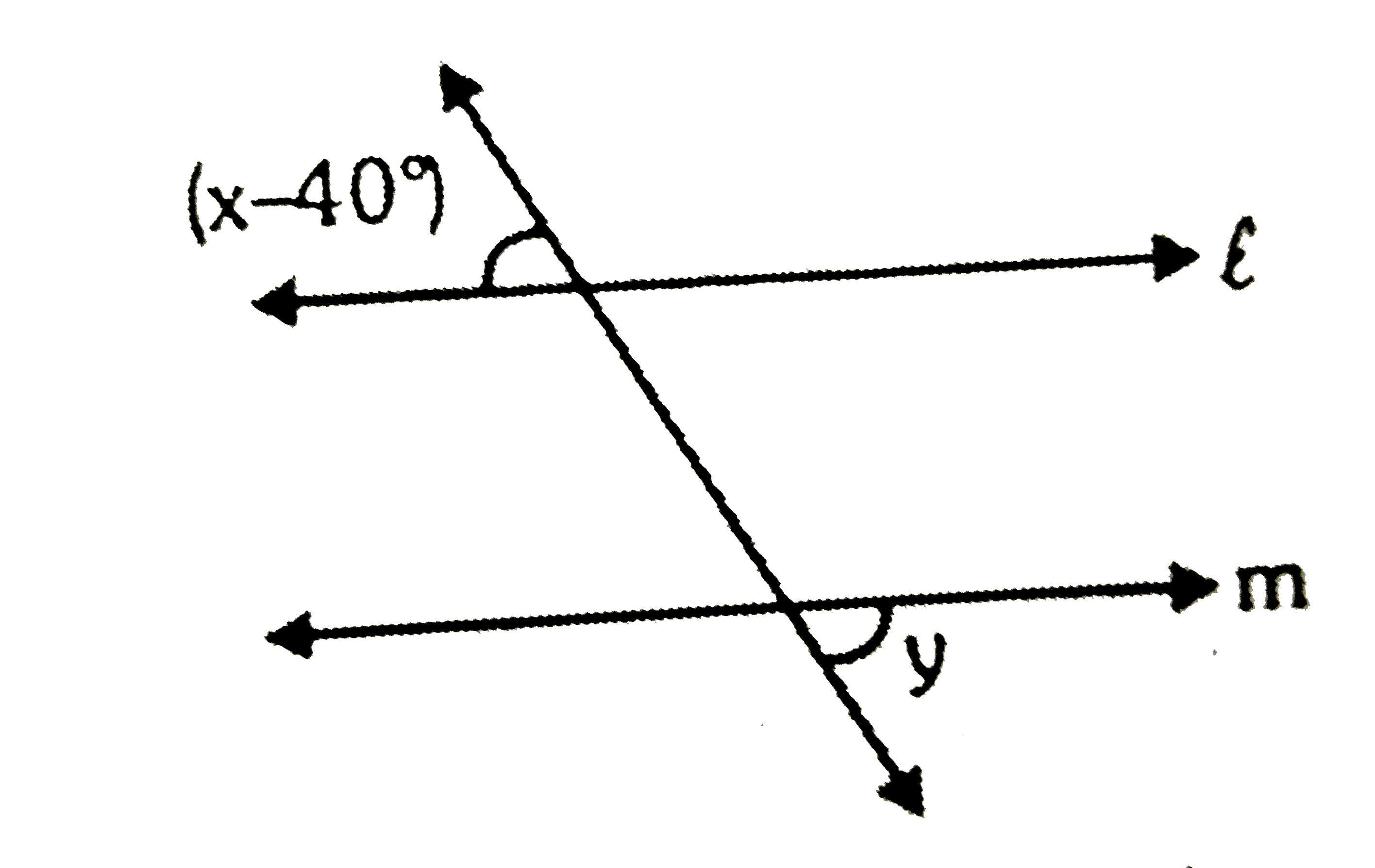 View Solution
View Solution

