Three points A,B,C are collinear they lie on the shown in the figure.can you draw △ABC?if not why .

Step by step video & image solution for Three points A,B,C are collinear they lie on the shown in the figure.can you draw triangleABC?if not why . by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES OF TRIANGLESBOOK - RS AGGARWALCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - EXERCISE 15A
15 Videos
- PROPERTIES OF TRIANGLESBOOK - RS AGGARWALCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - EXERCISE 15-B
8 Videos
- PROPERTIES OF PARALLEL LINE BOOK - RS AGGARWALCHAPTER - PROPERTIES OF PARALLEL LINE EXERCISE - EXERCISE 14
15 Videos
- RATION AND PROPORTION BOOK - RS AGGARWALCHAPTER - RATION AND PROPORTION EXERCISE - Test papers
18 Videos
Similar Questions
Knowledge Check
Given four points A, B, C, D such that three points ABC are collinear. By joining these points in order to get a closed figure, we get :-
AA Straight lineBA TriangleCA QuadrilateralDNone of theseA point C is said to lie between the points A and B if
AAC=ABBAC+CB=ABCpoints A,C and B are collinearDnone of these
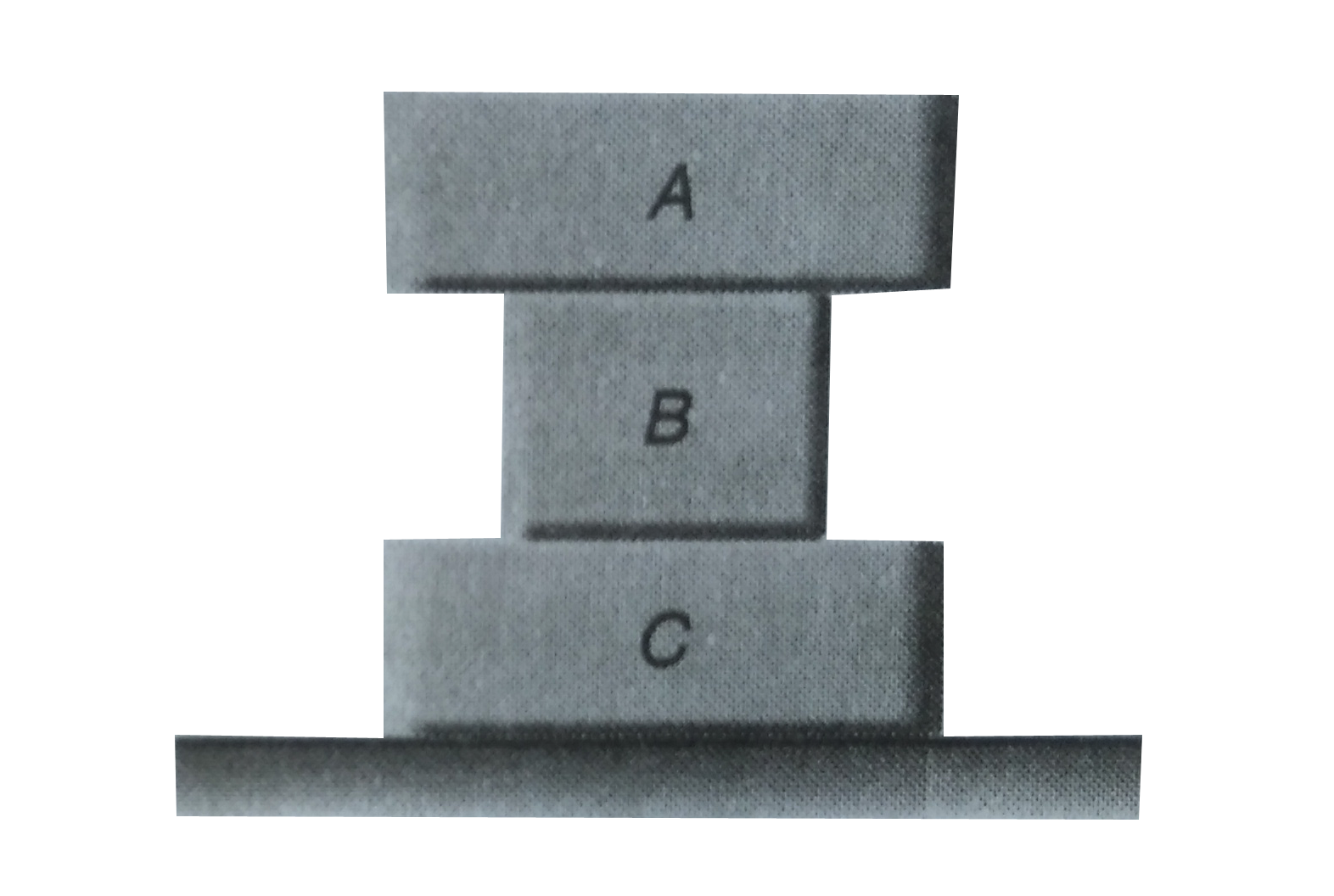
Three blocked A,B and C are placed one over the other as shown in figure. Draw free body diagram of all the three blocks.
View SolutionShow that the three points (3,0),(-2,-2) and (8,2) are collinear. Also, find the equation of the straight line on which these points lie.
View SolutionIf O be the origin the vector −−→OP is called the position vector of point P. Also −−→AB=−−→OB−−−→OA. Three points are said to be collinear if they lie on the same stasighat line.Points A,B,C are collinear if one of them divides the line segment joining the others two in some ratio. Also points A,B,C are collinear if and only if −−→AB×−−→AC=→0 Let the points A,B, and C having position vectors →a,→band→c be collinear Now answer the following queston: (A) veca.vecb=veca.vecc(B)vecaxxvecb=vecc(C)vecaxxvecb+vecbxxvecc+veccxxveca=vec0 (D) none of these
View SolutionIf the points A (x,y), B (1,4) and C (-2,5) are collinear, then shown that x + 3y = 13.
View SolutionThrough three collinear points a circle can be draw.
View SolutionIf A,B and C are three points on a line and B lies between A and C (as shown in the given figure), then prove that AB+ BC= AC
View Solution
