Question

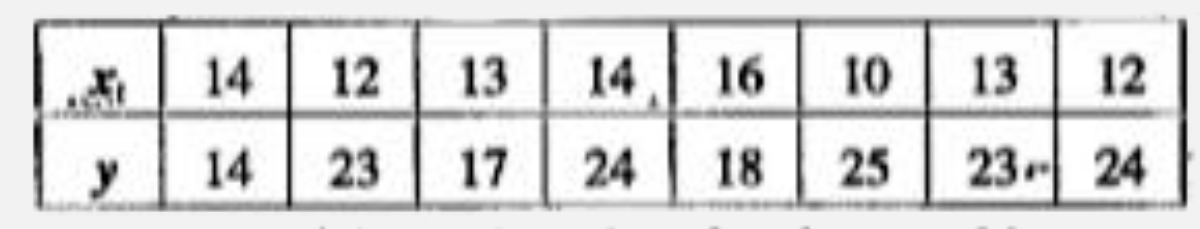
Treating x as dependent variable, find the line of best fit for the following data :
$\begin{array}{cccccc}x& 15& 12& 11& 14& 13\\ y& 26& 28& 24& 22& 30\end{array}$
Hence, predict the value of y when x = 10

Answer

`24 . 6 `

Explanation

To find the line of best fit treating $$x$$ as the dependent variable, we will follow these steps:

Step 1: Organize the Data
We have the following data points:
- \( x = (15, 12, 11, 14, 13) \)
- \( y = (26, 28, 24, 22, 30) \)

Step 2: Calculate the Summations
Calculate the summation of \( x \) and \( y \):
\( \sum x = 15 + 12 + 11 + 14 + 13 = 65 \)
\( \sum y = 26 + 28 + 24 + 22 + 30 = 130 \)

Step 3: Calculate the Means
Calculate the means \( \bar{x} \) and \( \bar{y} \):
\( \bar{x} = \frac{\sum x}{n} = \frac{65}{5} = 13 \)
\( \bar{y} = \frac{\sum y}{n} = \frac{130}{5} = 26 \)

Step 4: Calculate Deviations and Their Squares
Now, we calculate \( x - \bar{x} \), \( y - \bar{y} \), \( (x - \bar{x})^2 \), and \( (y - \bar{y})^2 \):
- For \( x - \bar{x} \):
- \( 15 - 13 = 2 \)
- \( 12 - 13 = -1 \)
- \( 11 - 13 = -2 \)
- \( 14 - 13 = 1 \)
- \( 13 - 13 = 0 \)

- For \( y - \bar{y} \):
- \( 26 - 26 = 0 \)
- \( 28 - 26 = 2 \)
- \( 24 - 26 = -2 \)
- \( 22 - 26 = -4 \)
- \( 30 - 26 = 4 \)

- Now calculate \( (x - \bar{x})^2 \) and \( (y - \bar{y})^2 \):
- \( (2)^2 = 4 \)
- \( (-1)^2 = 1 \)
- \( (-2)^2 = 4 \)
- \( (1)^2 = 1 \)
- \( (0)^2 = 0 \)

Step 5: Calculate Summations of Squares and Products
Now we calculate:
\( \sum (x - \bar{x})^2 = 4 + 1 + 4 + 1 + 0 = 10 \)
\( \sum (y - \bar{y})^2 = 0 + 4 + 4 + 16 + 16 = 40 \)
\( \sum (x - \bar{x})(y - \bar{y}) = (2)(0) + (-1)(2) + (-2)(-2) + (1)(-4) + (0)(4) = 0 - 2 + 4 - 4 + 0 = -2 \)

Step 6: Calculate the Slope \( b_{xy} \)
Now we can calculate the slope \( b_{xy} \):
\( b_{xy} = \frac{\sum (x - \bar{x})(y - \bar{y})}{\sum (y - \bar{y})^2} = \frac{-2}{40} = -0.05 \)

Step 7: Write the Regression Equation
The regression equation of \( x \) on \( y \) is given by:
\( x - \bar{x} = b_{xy}(y - \bar{y}) \)
Substituting the values:
\( x - 13 = -0.05(y - 26) \)
Rearranging gives:
\( x = -0.05y + 13 + 1.3 \)
\( x = -0.05y + 14.3 \)

Step 8: Predict \( y \) when \( x = 10 \)
Now, we need to predict the value of \( y \) when \( x = 10 \):
\( 10 = -0.05y + 14.3 \)
Rearranging gives:
\( -0.05y = 10 - 14.3 \)
\( -0.05y = -4.3 \)
Dividing by -0.05:
\( y = \frac{-4.3}{-0.05} = 86 \)

Final Answer
The predicted value of \( y \) when \( x = 10 \) is \( y = 86 \).

---