Tricia manages a health bar and wants to add a new fruit and protein smoothie to the menu. To decide on the new flavor she plans to offer. Tricia sold trial-sized banana smoothies and kiwi smoothies. She charged $2 for a banana smoothies and $2.50 for a kiwi smoothies and she told 40 in all, totalling $87. How much more maney did Tricia make on the banana smoothies than the kiwi smoothies?
12
17
26
52
The correct Answer is:B
To solve the problem step by step, we will set up equations based on the information given in the question.
Step 1: Define the Variables
Let:
- B = number of banana smoothies sold
- K = number of kiwi smoothies sold
Step 2: Set Up the Equations
From the problem, we know:
1. The total number of smoothies sold (banana + kiwi) is 40:
B+K=40
2. The total revenue from selling the smoothies is $87. The revenue from banana smoothies is $2 per smoothie and from kiwi smoothies is $2.50 per smoothie:
2B+2.5K=87
Step 3: Solve the First Equation for One Variable
From the first equation, we can express B in terms of K:
B=40−K
Step 4: Substitute into the Second Equation
Now, substitute B in the second equation:
2(40−K)+2.5K=87
Expanding this gives:
80−2K+2.5K=87
Combining like terms:
80+0.5K=87
Step 5: Isolate K
Subtract 80 from both sides:
0.5K=7
Now, divide by 0.5:
K=14
Step 6: Find B
Now that we have K, substitute back to find B:
B=40−K=40−14=26
Step 7: Calculate the Revenue from Each Type of Smoothie
Now, calculate the revenue from banana smoothies and kiwi smoothies:
- Revenue from banana smoothies:
Revenue from bananas=2B=2×26=52
- Revenue from kiwi smoothies:
Revenue from kiwis=2.5K=2.5×14=35
Step 8: Find the Difference in Revenue
Now, find how much more money Tricia made on banana smoothies than on kiwi smoothies:
Difference=Revenue from bananas−Revenue from kiwis=52−35=17
Final Answer
Tricia made $17 more on banana smoothies than on kiwi smoothies.
---
Let:
- B = number of banana smoothies sold
- K = number of kiwi smoothies sold
From the problem, we know:
1. The total number of smoothies sold (banana + kiwi) is 40:
B+K=40
2. The total revenue from selling the smoothies is $87. The revenue from banana smoothies is $2 per smoothie and from kiwi smoothies is $2.50 per smoothie:
2B+2.5K=87
From the first equation, we can express B in terms of K:
B=40−K
Now, substitute B in the second equation:
2(40−K)+2.5K=87
Expanding this gives:
80−2K+2.5K=87
Combining like terms:
80+0.5K=87
Subtract 80 from both sides:
0.5K=7
Now, divide by 0.5:
K=14
Now that we have K, substitute back to find B:
B=40−K=40−14=26
Now, calculate the revenue from banana smoothies and kiwi smoothies:
- Revenue from banana smoothies:
Revenue from bananas=2B=2×26=52
- Revenue from kiwi smoothies:
Revenue from kiwis=2.5K=2.5×14=35
Now, find how much more money Tricia made on banana smoothies than on kiwi smoothies:
Difference=Revenue from bananas−Revenue from kiwis=52−35=17
Tricia made $17 more on banana smoothies than on kiwi smoothies.
Topper's Solved these Questions
Similar Questions
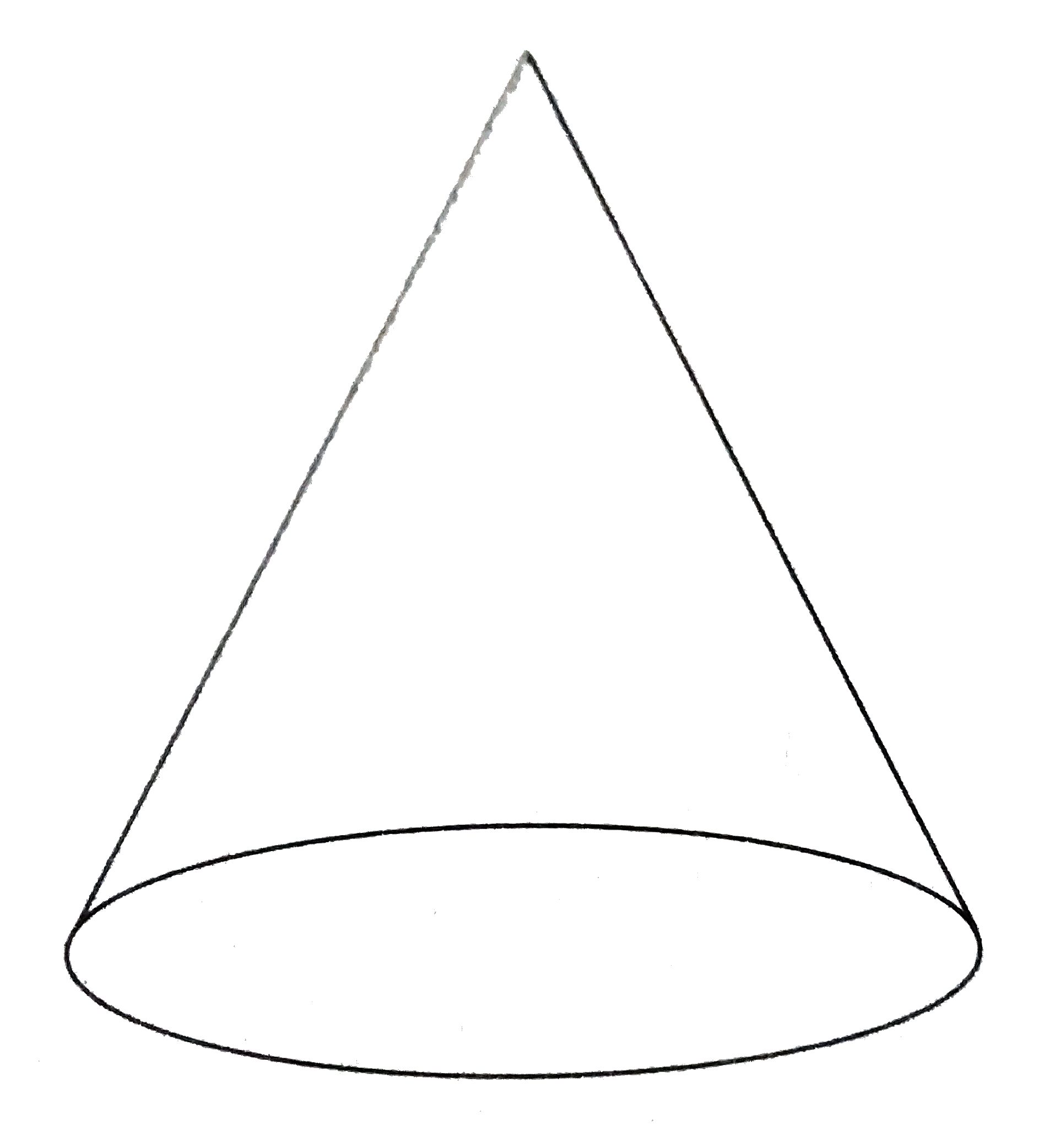
The lateral area of the right circular cone is 60π. If the radius of the base is 6, what is the volume of the cone
View SolutionIf x≠±1, then x+1x−1−x−1x+1=
View SolutionNew Banana plants develop from
View SolutionIniya bought 50 kg of fruits consisting of apples and bananas. She paid twice as much per kg for the apple as she did for the banana. If Iniya bought ₹1800 worth of apples and ₹600 worth bananas, then how many kg of each fruits did she buy?
View Solutionनया केले का पादप विकसित होता है?
View SolutionShalvi wants to organize her birthday party. She was happy on her birthday. She is very health conscious, thus she decided to serve fruits only.

She has 36 apples and 60 bananas at home and decided to serve them. She want to distribute fruits among guests. She does not want to discriminate among guests so she decided to distribute equally among all.
How many maximum guests Shalvi can invite?
View SolutionShalvi wants to organize her birthday party. She was happy on her birthday. She is very health conscious, thus she decided to serve fruits only.

She has 36 apples and 60 bananas at home and decided to serve them. She want to distribute fruits among guests. She does not want to discriminate among guests so she decided to distribute equally among all.
How many apples and bananas will each guest get?
View SolutionShalvi wants to organize her birthday party. She was happy on her birthday. She is very health conscious, thus she decided to serve fruits only.

She has 36 apples and 60 bananas at home and decided to serve them. She want to distribute fruits among guests. She does not want to discriminate among guests so she decided to distribute equally among all.
Shalvi decide to add 42 mangoes also. In this case how many maximum guests Shalvi can invite ?
View SolutionShalvi wants to organize her birthday party. She was happy on her birthday. She is very health conscious, thus she decided to serve fruits only.

She has 36 apples and 60 bananas at home and decided to serve them. She want to distribute fruits among guests. She does not want to discriminate among guests so she decided to distribute equally among all.
she also adds 42 mangoes,How many total fruits will each guest get?
View SolutionShalvi wants to organize her birthday party. She was happy on her birthday. She is very health conscious, thus she decided to serve fruits only.

She has 36 apples and 60 bananas at home and decided to serve them. She want to distribute fruits among guests. She does not want to discriminate among guests so she decided to distribute equally among all.
If Shalvi decide to add 45 mangoes instead OF 6 apple, in this case how many maximum guests Shalvi can invite ?
View SolutionNew Banana plants develop from
View SolutionSunandabai bought milk for ₹ 475. She converted it into yoghurt and sold It for ₹ 700. How much profit did she make?
View SolutionA fruit- seller sells 8 banana for a rupee gaining 25%. How banana did he buy for a rupee .
View SolutionSunandabai bought milk for ₹ 475. She converted it into yoghurt and sold It for ₹ 700. How much profit did she make?
View SolutionNew Banana plants develop from
View Solution
