Twelve typists, all working with same speed, type a certain number of pages in 18 days working 8 hours a day. Find, how many hours per day must sixteen typists work in order to type the same number of pages in 9 days ?
The correct Answer is:12 hours/days
To solve the problem step by step, we will use the concept of work done, which is given by the formula:
Work = Number of Typists × Hours per Day × Number of Days
Step 1: Calculate the total work done by 12 typists.
- Given:
- Number of typists = 12
- Hours per day = 8
- Days = 18
Using the formula:
Total Work=12 typists×8 hours/day×18 days
Calculating:
Total Work=12×8×18
=12×144
=1728 typist-hours
Step 2: Set up the equation for the work done by 16 typists.
- We need to find how many hours per day (let's denote it as x) 16 typists must work to complete the same amount of work in 9 days.
Using the same formula:
Total Work=16 typists×x hours/day×9 days
Step 3: Set the two expressions for work equal to each other.
Since both expressions represent the same total work:
12×8×18=16×x×9
Substituting the total work calculated in Step 1:
1728=16×x×9
Step 4: Simplify the equation.
First, simplify the right side:
1728=144x
Step 5: Solve for x.
To find x, divide both sides by 144:
x=1728144
Calculating:
x=12
Conclusion:
The number of hours per day that 16 typists must work is 12 hours.
---
- Given:
- Number of typists = 12
- Hours per day = 8
- Days = 18
Total Work=12 typists×8 hours/day×18 days
Total Work=12×8×18
=12×144
=1728 typist-hours
- We need to find how many hours per day (let's denote it as x) 16 typists must work to complete the same amount of work in 9 days.
Total Work=16 typists×x hours/day×9 days
Since both expressions represent the same total work:
12×8×18=16×x×9
1728=16×x×9
First, simplify the right side:
1728=144x
To find x, divide both sides by 144:
x=1728144
x=12
The number of hours per day that 16 typists must work is 12 hours.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - DIRECT AND INVERSE VARIATIONSEXERCISE - EXERCISE 10 (E)19videos
DIRECT AND INVERSE VARIATIONS
- BOOK - ICSECHAPTER - DIRECT AND INVERSE VARIATIONSEXERCISE - EXERCISE 10 C18videos
DIRECT AND INVERSE VARIATIONS
- BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 22 (B)10videos
DATA HANDLING
- BOOK - ICSECHAPTER - EXPONENTS (POWERS) EXERCISE - EXERCISE 2(B)50videos
EXPONENTS (POWERS)
Similar Questions
Eight men can dig a field 14 days,working 6 hours a day. In how many days can 7 men dig the same field, working 8 hours a day ?
View SolutionA man working 8 hours a day takes 5 days to complete a project. How many hours a day must he work to complete it in 4 days ?
View SolutionWorking 8 hours a day, Ashu can a book in 18 days. How many hours a day should he work so as to finish the work in 12 days?
View SolutionFour workers can build a house in 9 days. How many days would it takes 3 workers to build the same house?
View Solution90 men can complete a work in 24 days working 8 hours a day. How many men are working 8 hours a day. How many men are required to complete the same work in 18 days working 712 hours a day ?
View Solution36 men can do a piece of work in 7 days. How many men will do the same work in 42 days ?
View SolutionThe following table gives the number of pages written by Saria for completing her own book for 30 days.
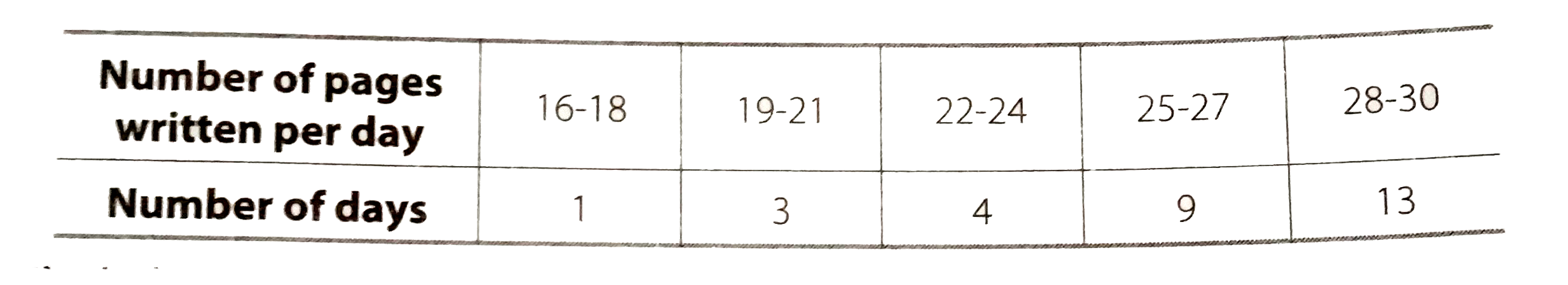
Find the mean number of pages written per day.View Solution2 men or 3 women can do a piece of work in 45 days. Find, in how many days will 6 men and 1 woman be able to complete the same work ?
View SolutionA man can work 8 hours daily and finishes a work in 12 days. If he works 6 hrs daily, in how many days will the same work be finished?
View SolutionIf 78 men can do a work in 140 days, in how many days will 42 men do the same work ?
View Solution
