Two circular path of radii a and b intersect at a point O and AB is a line through O meeting the circles at A and B respectively. Chords OA and OB subtend equal angles of 60∘ at their respective centres. A vertical pole at O subtends angles αandβ respectively at A and B then height of the pole is
acotα
bcotβ
a+bcotα+cotβ
None of these
The correct Answer is:C
Topper's Solved these Questions
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - EXERCISE (LEVEL-2 ( SINGLE CORRECT ANSWER TYPE QUESTIONS ))
15 Videos
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - EXERCISE (NUMERICAL ANSWER TYPE QUESTIONS )
5 Videos
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - EXERCISE (CONCEPT - BASED ( SINGLE CORRECT ANSWER TYPE QUESTIONS ))
5 Videos
- ELLIPSEBOOK - MCGROW HILL PUBLICATIONCHAPTER - ELLIPSEEXERCISE - Previous Years B-Architecture Entrance Examination Papers
6 Videos
- HYPERBOLABOOK - MCGROW HILL PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - QUESTION FROM PREVIOUS YEARS. B-ARCHITECTURE ENTRANCE EXAMINATION PAPERS
8 Videos
Similar Questions
If the arcs of the same length in two circles subtend angles of 60∘ and 75∘ at their respective centres, find the ratio of their radii?
View SolutionA vertical pole stands at a point O on a horizontal ground. A and B are points on the ground d metres apart. The pole subtends angles αandβ at A and B respectively. AB subtends an angle at O. the height of the pole is ______.
View Solution
Knowledge Check
If a chord AB subtends and angle of 60∘ at the centre of a circle, then the angle between the tangents to the circle drawn from A and B is
A30∘B60∘C90∘D120∘A pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point B on the boundary. If the chord AB subtends an angle α at the centre of the path, the height of the pole is
A2acos(α/2)tanαB2asin(α/2)cotαC2asin(α/2)tanαD2acos(α/2)cotαA vertical pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point β on the boundary. If the chord AB subtends an angle α at the centre of the park, the height of the pole is
A2a sinα2tanαB2a cosα2tanαC2a sinα2cotαD2a cosα2cotα
If a chord AB subtends and angle of 60∘ at the centre of a circle, then the angle between the tangents to the circle drawn from A and B is
View SolutionIn a circle with centre O. an arc ABC subtends an angle of 136∘ at the centre of the circle. The chord AB is produced to a point P. Then ∠CBP is equal to :
View SolutionA vertical pole stands at a point A on the boundary of a circular park of radius 2 km and subtends an angle 60∘ at another point B on the boundary. If the chord AB subtends the same angle 60∘ at the centre of the park, the height of the pole is
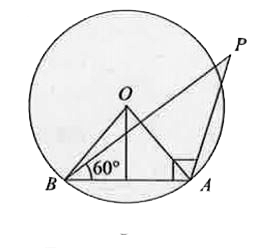
View SolutionIn a circle with centre O an are ABC subtends an angle of 140∘ at the centre of the circle . The chord AB is produced to point P. Then ∠CBP is equal to :
View SolutionAB is a vertical pole resting at the end A on the level ground. P is a point on the level ground such that AP = 3AB and C Is the midpoint of AB. If AC and CB subtend angles α and β, respectively, at P, then the value of tanβ is
View Solution
