Two godowns A and B have grain capacity of 100 quintal and 50 quintal respectively. They supply to 3 ration shops, D, E and F, whose requirements are 60, 50 and 40 quintals respectively. The cost of transportation per quintal from the godowns to the shops are given in the following table:

How should the supplies be transpored in order that the transportation cost is minimum cost?
The correct Answer is:From A to D,E,F,10,50,40 quintals respectively and from B to D,E,F 50,0,0 quintals respectively, Minimum cost =rs.510
NA
Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12.1
10 Videos
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12.2
11 Videos
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12 A
8 Videos
- INVERES TRIGONOMETRIC FUNCTIONSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - INVERES TRIGONOMETRIC FUNCTIONSEXERCISE - Miscellaneous Exercise (prove That )
9 Videos
- MATRICESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - MATRICESEXERCISE - Miscellaneous Exerice
15 Videos
Similar Questions
Two godowns A and B have grain capacity of 100 quintals and 50 qunitals respectively. They supply to 3 ration shops, D,E and F whose requirements are 60,50 and 40 quintals repectively.The cost of transportation per quintal from the godowns to the shops are given in the following table:
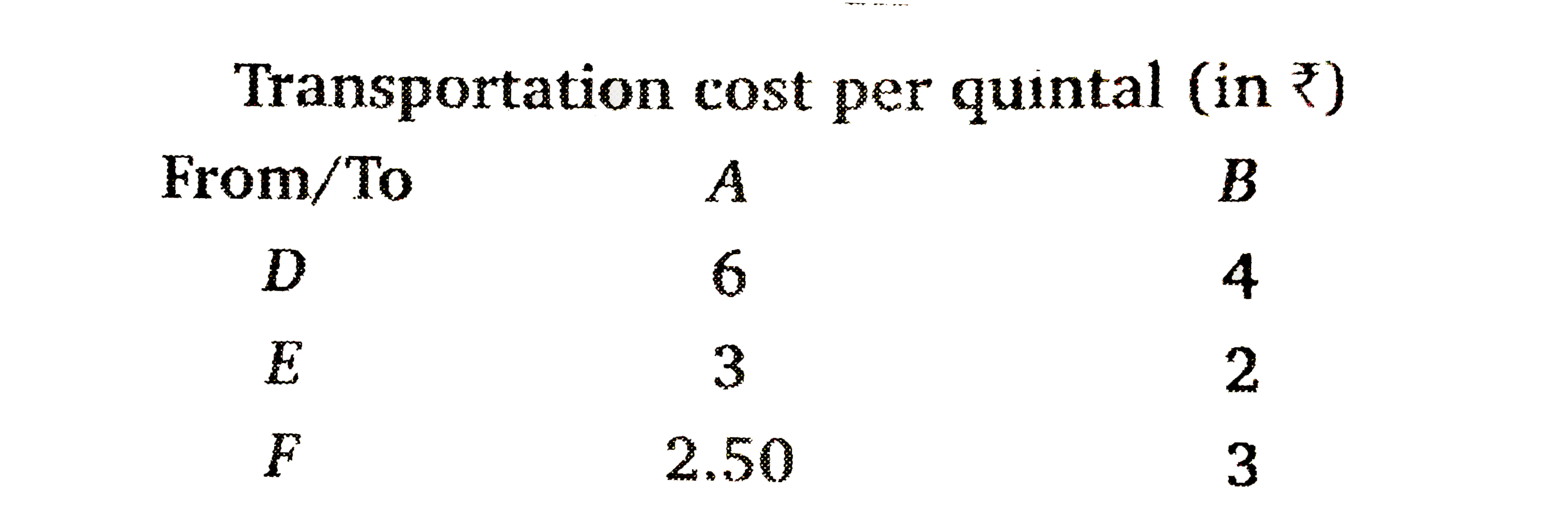
How should the supplies be transported in order that the transportation cost is minimum? What is the minimum cost?
View SolutionAn oil company has two depots, A and B, with capacities of 7000 L and 4000 L respectively. The company is to supply oil to three pumps D,E,F whose requirements are 4500 L, 3000 L and 3500 L respectively. The distance (in Km) between the depots and the petrol pumps are given in the following table :
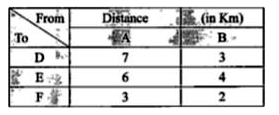
Assuming that the transportation cost of10 litres of oil is ₹ 1 per km , how should the delivery be scheduled in order that the transportation cost is minimum ?
View SolutionA brick manufacturer has two depots A and B with stocks of 30000 and 20000 bricks respectively. He receives orders from three builders P, Q and R for 15000, 20000 and 15000 bricks respectively. The cost of transporting 1000 bricks to the builders from the depots (in rupees) are given below:
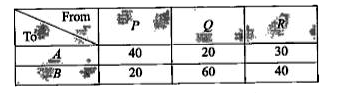
How should the manufacturer fulfil the order so as to keep the cost of transportation minimum? What is the minimum cost?
View SolutionA farm is engaged in breeding hens. The hens are fed products A and B grown in the farm which contains nutrients P, Q and R. One kilogram of product A contains 36 units, 3 units and 20 units of nutrients P Q and R respectively, whereas one kilogram of product B contains 6 units, 12 units and 10 units of nutrients P, Q and R respectively. The minimum requirement of nutrients P Q and R for a hen is 108, 36 and 100 units respectively. Product A costs 20 per kilogram and product B costs 40 per kilogram. Using linear programming, find the number of kilograms of products A and B to be produced to minimize the total cost. Identify the feasible region from the rough sketch.
View SolutionVitamins A and B are found in two different foods F1 and F2 . One unit of food F1 contains 2 units of vitamin A and 3 units of vitamin B. One unit of food F2 contains 4 units of vitamin A and 2 units of vitamin B. One unit of food F1 and F2 cost Rs. 50 and 25 respectively. The minimum daily requirements for a person of vitamin A and B is 40 and 50 units respectively. Assuming that anything in excess of daily minimum requirement of vitamin A and B is not harmful, find out the optimum mixture of food F1 and F2 at the minimum cost which meets the daily minimum requirement of vitamin A and B. Formulate this as a LPP.
View SolutionA medical company has factories at two places A and B. From these places supply is made to each of its three agencies situated at P, Q and R. The monthly requirements of the agen cies are respectively 40,40 and 50 packets of the medicines, while the production capacity of the factories A and B are 60 and 70 packets respectively. The transportation costs per packet from the factories to the agencies are given below:

View SolutionA diet for a sick person must contain at least 4000 units of vitamins, 50 units of minerals and 1400 calories. Two foods X and Y are available at the cost of रु 4 and रु 3 per unit respectively. One unit of the food X contains 200 units of vitamins, 1 unit of minerals and 40 calories, whereas one unit of the food Y contains 100 units of vitamins, 2 units of minerals and 40 calories. Find what combination of X and Y should be used to have least cost, satisfying the requirements.
View SolutionA farmer mixes two brands P and Q of cattle feed. Brand P costing Rs. 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs. 200 per bag contains 1.5 units of nutritonal element A, 11.25 units of element B and 3 units of element C. The minimum requirements of nutrients A,B and C are 18 units, 45 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost per bag? What is the minimum cost of the mixture per bag?
View SolutionIn order to supplement daily diet, a person wishes to take X and Y tablets. The contents (in milligrams per tablet) of iron, calcium and vitamins in X and Y are given as below : Tablets Iron Calcium Vitamin X 6 3 2 Y 2 3 4 The person needs to supplement at least 18 milligrams of iron, 21 milligrams of calcium and 16 milligrams of vitamins. The price of each tablet of X and Y is Rs. 2 and Rs. 1 respectively. How many tablets of each type should the person take in order to satisfy the above requirement at the minimum cost ? Make an LPP and solve graphically.
View SolutionA diet for a sick person must contain at least 4000 units of vitamins, 50 units of minerals and 1400 calories. Two foods A and B, are available at a cost of Rs. 4 and Rs. 3 per unit respectively. If one unit of A contains 200 units of vitamin, 1 unit of mineral and 40 calories and one unit of food B contains 100 units of vitamin, 2 units of minerals and 40 calories, find what combination of foods should be used to have the least cost?
View Solution
