Two hunters A and B set out to hunt ducks. Each of them hits as often as he misses when shooting at ducks. Hunter A shoots at 50 ducks and hunter B shoots at 51 ducks. The probability that B bags more ducks than A can be expressed as pq in its lowest form. Find the value of (p+q).
The correct Answer is:3
Topper's Solved these Questions
- PROBABILITY BOOK - VK JAISWALCHAPTER - PROBABILITY EXERCISE - Exercise-4 : Matching Type Problems
1 Videos
- PERMUTATION AND COMBINATIONS BOOK - VK JAISWALCHAPTER - PERMUTATION AND COMBINATIONS EXERCISE - Exercise-5 : Subjective Type Problems
13 Videos
- QUADRATIC EQUATIONS BOOK - VK JAISWALCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
45 Videos
Similar Questions
Let S=1,2,3,4,5,6,7,8,9,10. Two subscts A and B of S are chosen randomly with replacement with B chosen after A. The probability that A is a subset of B can be expressed as paq8 for some s p and q. Find the value of (ba+p+q).
View SolutionGiven a triangle ABC with sides a=7,b=8 and c=5. If the value of the expression (∑sinA)(∑cotA2) can be expressed in the form pq where p,q∈N and pq is in its lowest form find the value of (p+q).
View SolutionA bag 'A' contains 2 white and 3 red balls and bag 'B' contains 4 while and 5 red balls. One ball is drawn at random from a randomly chosen bag and is found to be red. If the proability that it was drawn from bag 'B' can be expressed as rational pq (where p and q are in their lowest form), then find (p+q).
View SolutionSuppose AandB shoot independently until each hits his target. They have probabilities 3/5 and 5/7 of hitting the targets at each shot. Find probability that B will require more shots than A.
View SolutionMr. A writes an article. The article originally is error free. Each day Mr. B introduces one new error into the article. At the end of the day, Mr. A checks the article and has 23 chance of catching each individual error still in the article. After 3 days, the probability that the article is error free can be expressed as pq where p and q are relatively prime positive integers. Let λ=q−p, then find the sum of the digits of λ.
View SolutionMr. A writes an article. The article originally is error free. Each day Mr. B introduces one new error into the article. At the end of the day, Mr. A checks the article and has 23 chance of catching each individual error still in the article. After 3 days, the probability that the article is error free can be expressed as pq where p and q are relatively prime positive integers. Let λ=q−p, then find the sum of the digits of λ.
View SolutionLet a,b,c,d are distinct numbers to be chosen from the set {1,2,3,4,5}. If the least possible positive solution for x to the system of equations ax+by=1cx+dy=2} can be expressed in the form pq where p and q are relatively prime, then find the value of (p+q).
View Solution2 hunters A & B shot at a bear simultaneously. The bear was shot dead with only one hole in its hide.Probability of A shooting the bear 0.8 & that of B shooting the bear is 0.4. The hide was sold for Rs. 280/-. If this sum of money is divided between A & B in a fair way, then find their respective shares.
View SolutionColumn I, Column II If the probability of getting at least one head is at least 0.8 in n trials, then value of n can be, p. 2 One mapping is selected at random from all mappings of the set s={1,2,3,...,n} into itself. If the probability that the mapping being one-one is 3/32, then the value of n is, q. 3 If m is selected at random from set {1,2,...,10} and the probability that the quadratic equation 2x2+2mx+m+1=0 has real roots is k, then value of 5k is more than, r. 4 A man firing at a distant target as 20% chance of hitting the target in one shoot. If P be the probabililty of hitting the target in n attempts , where 20P2−13P+2≤0, then the ratio of maximum and minimum value of n is less than, s. 5
View SolutionTwo parallel beams of light P and Q (separation d) containing radiation of wavelengths 4000A and 5000A (which are mutually coherent in each wavelength separately) are incident normally on a prism as shown in fig. The refractive index of the prism as a function of wavelength is given by the relation. μ(λ)=1.20+bλ2 Where λ is in A and b is positive constant. The value of b is such that the condition wave length and is not satisfied for the other.
(a) Find the value of b.
(b) find the deviation of the beams transmitted through the face AC. (c) A convergent lens is used to bring these transmitted beams into focus. If the intensities of transmission form the face AC, are 41 and I respectively, find the resultant intensity at the focus.
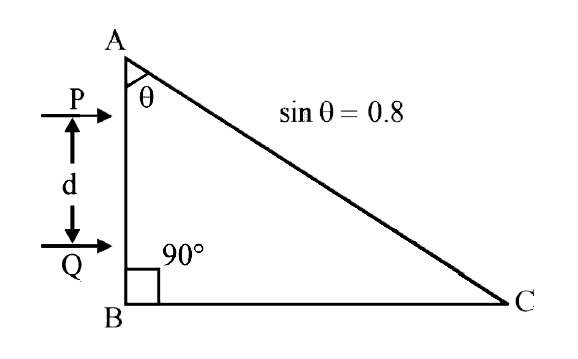
View Solution
