Two packets of sweets weight 278kgand314kg respectively. How much is the total weight of the sweets ?
518kg
618kg
398kg
578kg
The correct Answer is:B
To find the total weight of the two packets of sweets, we need to add their weights together. The weights given are 278kg and 314kg.
Step 1: Convert Mixed Numbers to Improper Fractions
First, we convert the mixed numbers into improper fractions.
For 278:
278=(2×8)+78=16+78=238
For 314:
314=(3×4)+14=12+14=134
Step 2: Find a Common Denominator
Next, we need to find a common denominator to add the two fractions. The denominators are 8 and 4. The least common multiple (LCM) of 8 and 4 is 8.
Step 3: Convert the Second Fraction
Since the first fraction is already with a denominator of 8, we need to convert the second fraction 134 to have a denominator of 8:
134=13×24×2=268
Step 4: Add the Two Fractions
Now we can add the two fractions:
238+268=23+268=498
Step 5: Convert the Improper Fraction to a Mixed Number
Finally, we convert 498 back to a mixed number:
49÷8=6(remainder 1)
So, 498=618.
Final Answer
The total weight of the sweets is 618kg.
---
First, we convert the mixed numbers into improper fractions.
278=(2×8)+78=16+78=238
314=(3×4)+14=12+14=134
Next, we need to find a common denominator to add the two fractions. The denominators are 8 and 4. The least common multiple (LCM) of 8 and 4 is 8.
Since the first fraction is already with a denominator of 8, we need to convert the second fraction 134 to have a denominator of 8:
134=13×24×2=268
Now we can add the two fractions:
238+268=23+268=498
Finally, we convert 498 back to a mixed number:
49÷8=6(remainder 1)
So, 498=618.
The total weight of the sweets is 618kg.
Topper's Solved these Questions
- RATIONAL NUMBERS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS) (LEVEL-2)
15 Videos
- RATIONAL NUMBERS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - EXERCISE (MATCHING)
2 Videos
- RATIONAL NUMBERS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - NCERT SECTION (EXERCISE -9.2)
25 Videos
- PREP TEST 2 BOOK - MTG IIT JEE FOUNDATIONCHAPTER - PREP TEST 2 EXERCISE - SECTION-B
30 Videos
- SIMPLE EQUATIONSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SIMPLE EQUATIONSEXERCISE - Olympiad /Hots Corner
18 Videos
Similar Questions
Atul bought 212kg mangoes and 434kg oranges.What is the total weight of fruits "
View SolutionAverage of the weight of 150 students of a school is 60 kg. If the average weight of the boys is 52 kg and the average weight of the girls is 30 kg, then what will be the respective ratio of the total weight of boys and the total weight of girls?
View Solution
Knowledge Check
Sameera purchased 312 kg apples and 434 kg oranges. What is the total weight of fruits purchased by her?
A814B14C72D418Aneeta bought 334 kg apples and 412 kg guava. What is the total weight of fruits purchased by her?
A714kgB814kgC914kgD614kgAverage of the weight of 138 students of a school is 45 kg. If the average weight of the boys is 49 kg and the average weight of the girls is 25 kg, then what will be the respective ratio of the total weight of boys and the total weight of girls?
(a)5:43
(b)49:5
(c)3:49
(d)7:1A5:43B49:5C3:49D7:1
Sameera purchased 312 kg apples and 434 kg oranges. What is the total weight of fruits purchased by her?
View SolutionThe average weight of 6 men is 65 kgs. Two of them weight 70 and 43 kgs respectively. What is the average weight of remaining four?
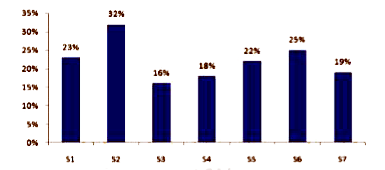
View SolutionThe bar chart given below shows the amount of sugar used in making 7 different varieties of sweets S1, S2, S3, S4, S5, S6 and S7 has been show as a percentage of total weight of the sweet.
The total weight of sweet S4 is 150 kg. What is the amount of sugar used in sweet S4
?
View SolutionThree boxes weigh 1834kg, 712kg and 1015 kg respectively. A porter carries all the three boxes. What is the total weight carried by the porter?
View SolutionA bucket when filled 57 with water weights 12 kg and filled 34 of it with water weight 12.5 kg. What is the weight of the empty bucket?
View Solution
