Two persons A and B took a wire of equal length 'A' bent in the form of a reactangle with perimeter 12 cm. B bet it in the form of a square, then find the area of the square , then find the area of the square formed.
The correct Answer is:9cm2
Let the side of the square be a.
Since A and B took an equal length of the wire.
∴ Perimeter of the square= Perimeter of the rectangle
4a=12
a=3cm
Area of the square =a2=32=9cm2.
Topper's Solved these Questions
- MENUSRATION BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MENUSRATION EXERCISE - Concept application
5 Videos
- MENUSRATION BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MENUSRATION EXERCISE - Assessment Test
15 Videos
- MENUSRATION BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MENUSRATION EXERCISE - very short type questions
22 Videos
- GEOMETRYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - CROSSWORD
1 Videos
- NUMBERSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - NUMBERSEXERCISE - CROSSWORD
1 Videos
Similar Questions
Knowledge Check
If area of a square is 64 sq cm, then find the area ofthe circle formed by the same perimeter.
A215πsqcmB216πsqcmC256πsqcmD318πsqcm
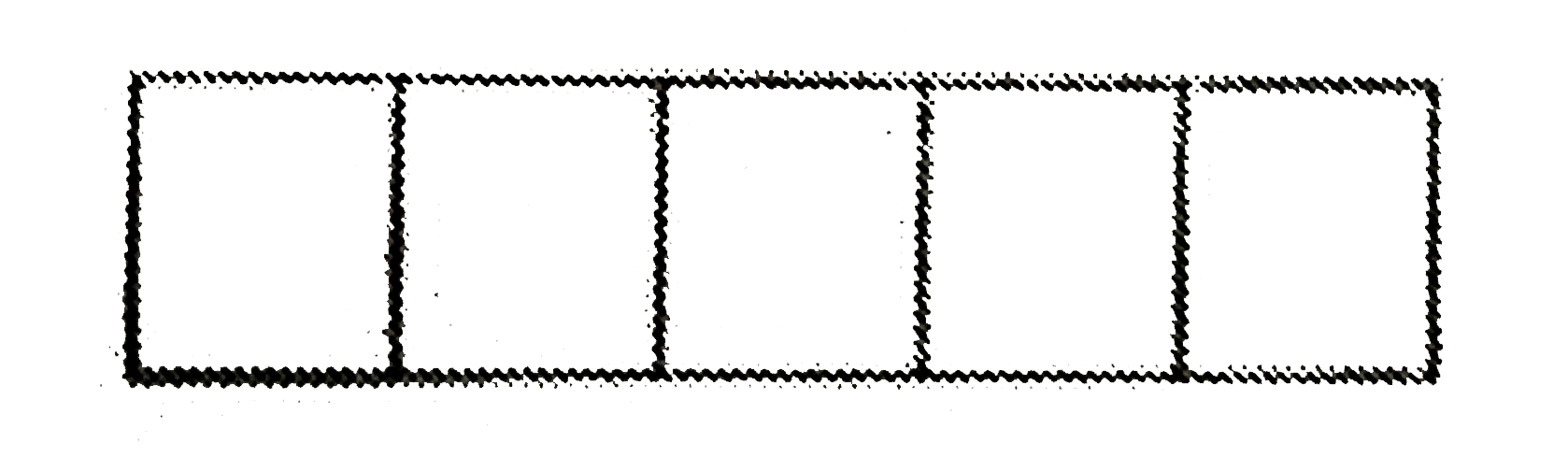
A rectangle of perimeter 276 cm is cut into five equal squares as shown below. Find the area of each squares.
View SolutionA wire is in a circular shape of radius 28 cm. If it is bent in the form of a square, what will bethe area of the square formed?
View SolutionFind the area of a square whose perimeter is 84 cm.
View SolutionA wire is looped in the form of a circle of radius 35 cm. If it is rebent in the form of a square,what will be the length of each side of the square?
View SolutionA steel wire when bent in the form of a square encloses an area of 121 cm2 . If the same wire is bent in the form of a circle, find the area of the circle.
View SolutionA steel wire when bent in the form of a square encloses an area of 121 cm2 . If the same wire is bent in the form of a circle, find the area of the circle.
View SolutionA wire is in the form of a circle of radius 21cm. If is bent into a square, then side of the square is
View Solution
