Two ships leave port at the same time. One goes 24 km per hour in the direction N 450E and other travels 32 km per hour in the direction S750E. Find the distance between the ships at the end of 3 hours.
Let A and B be the positions of the two ships at the end of 3 houts brgt Then, OA=(24×3)km=72km.
And, OB=(32×3)km=96km.
Let, AB=xkm.
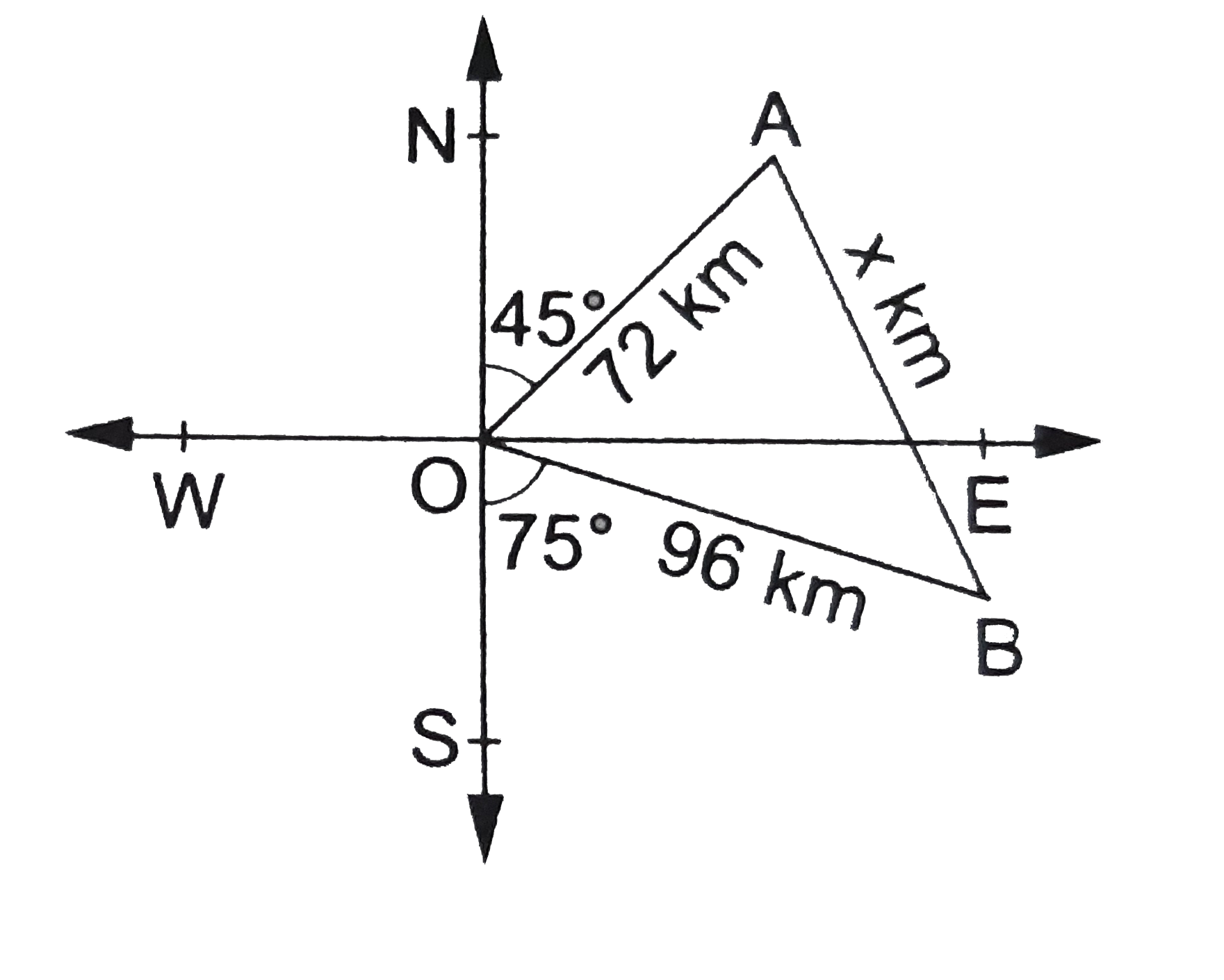
We have: ∠NOA=45∘and∠SOB=75∘.
∴ ∠AOB=180∘−(45∘+75∘)=60∘.
Applying cosine formula on ΔABC, we get
cos60∘=(72)2+(96)2−x22×72×96
⇒ (72)2+(96)2−x2=12×2×72×96
⇒5184+9216−x2=6912
⇒ x2=14400−6912=7488
⇒ x=√7488=86.53km.
Hence, the distance between the ships at the end of 3 hours is 86.53 km.
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - SOLUTION OF TRIANGLESEXERCISE - EXERCISE 18 A22videos
SOLUTION OF TRIANGLES
- BOOK - RS AGGARWALCHAPTER - SOLUTION OF TRIANGLESEXERCISE - EXERCISE 18 B3videos
SOLUTION OF TRIANGLES
- BOOK - RS AGGARWALCHAPTER - SOLUTION OF TRIANGLESEXERCISE - EXERCISE 18 B3videos
SOLUTION OF TRIANGLES
- BOOK - RS AGGARWALCHAPTER - SETSEXERCISE - EXERCISE-1H15videos
SETS
- BOOK - RS AGGARWALCHAPTER - SOME SPECIAL SERIESEXERCISE - EXERCISE 13B (Very short Answer Questions)9videos
SOME SPECIAL SERIES
Similar Questions
Two ships leave port at the same time. One goes 24 km per hour in the direction N 450E and other travels 32 km per hour in the direction S750E. Find the distance between the ships at the end of 3 hours.
View SolutionTwo ships leave a port at the same time. One goes 24 km/hr in the direction N380E and other travels 32 km/hr in the directionS520 E. Find the distance between the ships at the end of 3 hrs.
View Solution
Knowledge Check
- Question 1 - Select One
The following questions are based on the table given below which represents the distance (in km) travelled by two persons A and B is the same direction :
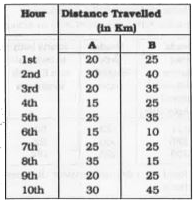
What is the distance (in km) between A a nd B at the end of 8th hour?A30B25C15D12 - Question 2 - Select One
The speed of a boat in a river is 20 km/h and the speed of another boat is 23 km/h. They travel in the same direction from the same place at the same time. The distance between the boats after three and half hours is
A11.5 kmB10 kmC10.5 kmD11 km - Question 3 - Select One
Two cars leave the same place at the same time. One runs at 20 km/ hr towards north and the other at 15 km/hr towards east. What will be the distance between them (in km) after 2 hours of travelling?
A25B50C70D10
Two ships leave a port at the same time. One goes 24 km per hour in the direction N45oEand other travels 32 km per hour in the direction S75oE. Find the distance between the ships at the end of 3 hours.
View SolutionTwo boats leave a port at the same time. One travels 60 km in the direction N50∘E while the other travale 50 km in the direction S70∘E. What is the distance between the boats ?
View SolutionTwo boats leave a place at the same time. One travels 56 km in the direction N 40∘ E, while the other travels 48 km in the direction S 80∘ E. What is the distance between the boats?
View SolutionA motor car does a journey in 10 hours, the first half at 21 km per hour, and the rest at 24 km per hour. Find the distance.
View SolutionTwo ships move from a point at the same time. First ship moves at the speed of 24 km/hr at 45∘ angle from north to east and second ship moves at the speed of 32 km/hr at 75∘ angle from south to east. Find the distance between them after 3 hours.
View Solution

