Two sides of a rhombus lying in the first quadrant are given by 3x−4y=0and12x−5y=0 . If the length of the longer diagonal is 12, then find the equations of the other two sides of the rhombus.
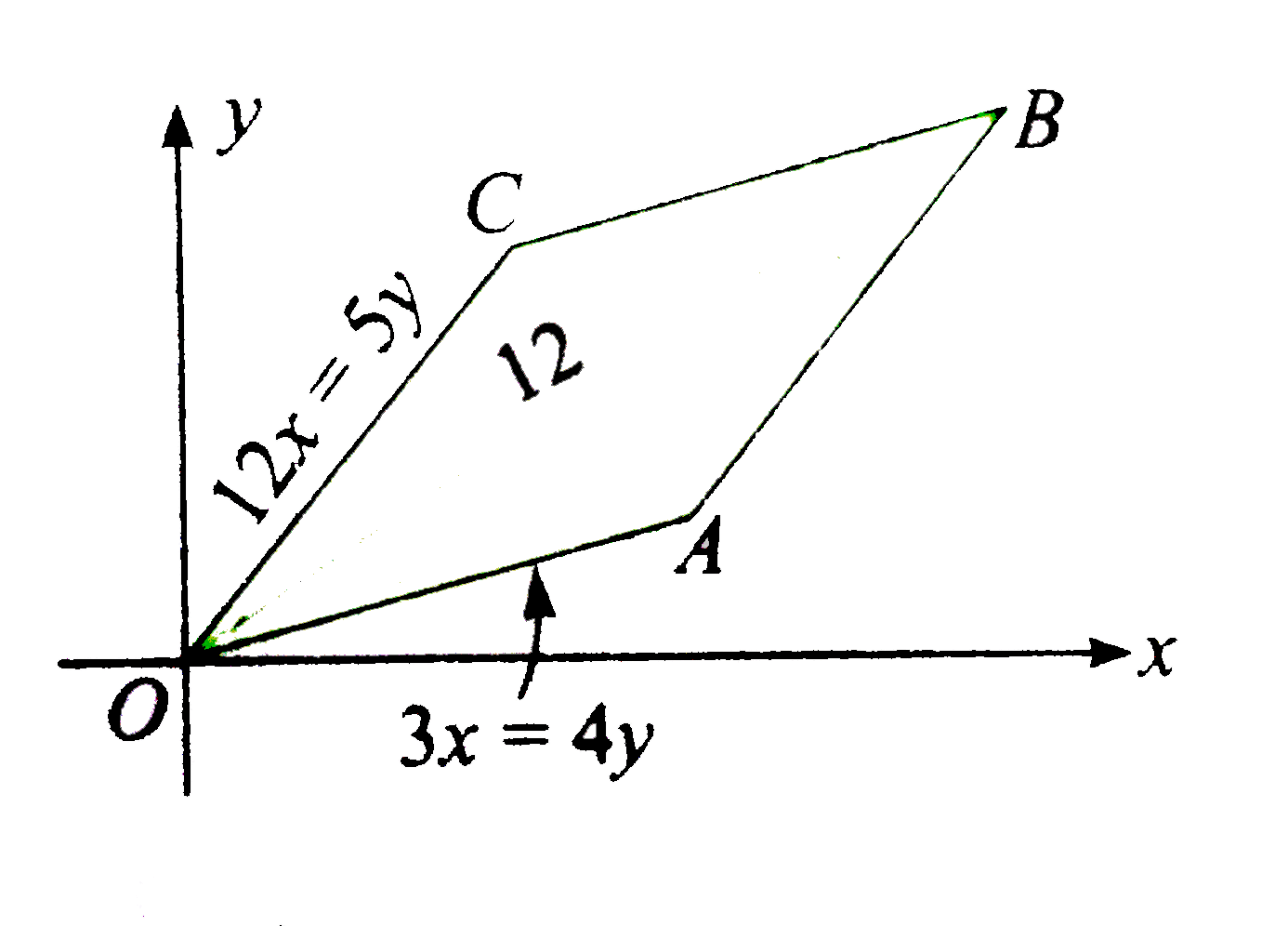
OB is along the acute angle bisector of the given lines: 3x-4y=0 and 12x-5y =0.
The equation of acute angle bisector is
3x−4y5=−12−5y13
or 9x-7y=0
∴Slope of OB=tanθ=97
Now, OB= 12.
Therefore, the coordinates of B are
(12cosθ,12sinθ)≡(127√130,129√130)≡(84√130,108√130)
AB is passing through B and is parallel to OC.
Therefore, the equation of AB is
y−108√130=125(x−84√130)
BC is passing through B and is parallel to OA.
Therefore, the equation of BC is y−108√130=34(x−84√130)
Topper's Solved these Questions
- STRAIGHT LINESBook:CENGAGEChapter:STRAIGHT LINESExercise:Exercise 2.1
Explore 23 Videos
- STRAIGHT LINESBook:CENGAGEChapter:STRAIGHT LINESExercise:Exercise 2.2
Explore 4 Videos
- STRAIGHT LINEBook:CENGAGEChapter:STRAIGHT LINEExercise:Multiple Correct Answers Type
Explore 8 Videos
- THEORY OF EQUATIONSBook:CENGAGEChapter:THEORY OF EQUATIONSExercise:JEE Advanced Previous Year
Explore 9 Videos
Similar Questions
Two sides of a rhombus lying in the first quadrant are given by 3x−4y=0 and 12x−5y=0. If the length of the longer diagonal is 12, then find the equations of the other two sides of the rhombus.
View SolutionTwo consecutive sides of a parallelogram are 4x+5y=0 and 7x+2y=0 . If the equation of one diagonal is 11x=7y=9, find the equation of the other diagonal.
View Solution
Knowledge Check
The lengths of the diagonals of a rhombus are 16 cm and 12 cm. Then, the length of the side of the rhombus is
A9 cmB10 cmC8 cmD20 cmThe lengths of the diagonals of a rhombus are 16 cm and 12 cm . The length of each side of the rhombus is
A8cmB9 cmC10 cmD12 cmThe lengths of two diagonals of a rhombus are 12 cm and 16 cm. What is the side (in cm) of the rhombus?
A10B20C10√2D20√2
Each side of a rhombus is 10 cm long and one of its diagonals measures 16 cm. Find the length of the other diagonal and hence find the area of the rhombus.
View SolutionThe diagonals of a rhombus are 16cm and 12cm. Find the length of each side of the rhombus.
View SolutionIf the side of a rhombus is 10 cm and the longer diagonal is 16 cm, find the length of the other diagonal and hence the area of the rhombus.
View SolutionThe combined equation of two adjacent sides of a rhombus formed in first quadrant is 7x2−8xy+y2=0 then slope of its longer diagonal is
View SolutionTwo adjacent sides of a rhombus are given by 2x2−47xy+2y2=0. If length of the shorter diagonal passing through their point of intersection is 10√2 Then find the total number of possible positions of the vertex lying on it other than origin.
View Solution