Two spheres of radii r1andr2, cut orthogonally. The radius of the common chord is 1r1r2√(r21+r22)
The correct Answer is:T
To solve the problem of finding the radius of the common chord between two orthogonally intersecting spheres of radii r1 and r2, we will follow a systematic approach:
1. Understanding the Geometry:
- We have two spheres with centers C1 and C2 and radii r1 and r2 respectively.
- The spheres intersect orthogonally, meaning the angle between their radii at the intersection points is 90∘.
Hint: Visualize the two spheres and their centers. The intersection forms a common chord.
2. Identifying the Common Chord:
- Let the points where the spheres intersect be A and B. The line segment AB is the common chord.
- Let L be the midpoint of the chord AB. The length AL is half the length of the chord.
Hint: Label the points clearly to keep track of the geometry.
3. Using Trigonometric Relationships:
- In triangle C1AL:
- AL=r1sin(θ) where θ is the angle at C1.
- In triangle C2AL:
- AL=r2cos(θ) since ∠C2AL=90∘−θ.
Hint: Remember the sine and cosine definitions in right triangles.
4. Setting Up the Equations:
- From the above relationships, we can write:
AL=r1sin(θ)(1)
AL=r2cos(θ)(2)
Hint: You can express sin(θ) and cos(θ) in terms of AL.
5. Using the Pythagorean Identity:
- From trigonometric identities, we know:
sin2(θ)+cos2(θ)=1
- Substituting equations (1) and (2) into this identity gives:
(ALr1)2+(ALr2)2=1
Hint: This is a crucial step that connects the lengths with the radii.
6. Simplifying the Equation:
- Rearranging gives:
AL2r21+AL2r22=1
- Factoring out AL2:
AL2(1r21+1r22)=1
Hint: Factor out common terms to simplify the equation.
7. Solving for AL2:
- Thus, we can express AL2 as:
AL2=11r21+1r22=r21r22r21+r22
Hint: This step involves taking the reciprocal of the sum of fractions.
8. Finding AL:
- Taking the square root gives:
AL=r1r2√r21+r22
Hint: Ensure to take the positive root since lengths are positive.
9. Finding the Radius of the Common Chord:
- The length of the common chord AB is:
AB=2⋅AL=2⋅r1r2√r21+r22
Hint: Remember that the common chord length is twice the distance from the center to the chord.
Final Result
The radius of the common chord is given by:
Radius of common chord=r1r2√r21+r22
- We have two spheres with centers C1 and C2 and radii r1 and r2 respectively.
- The spheres intersect orthogonally, meaning the angle between their radii at the intersection points is 90∘.
- Let the points where the spheres intersect be A and B. The line segment AB is the common chord.
- Let L be the midpoint of the chord AB. The length AL is half the length of the chord.
- In triangle C1AL:
- AL=r1sin(θ) where θ is the angle at C1.
- In triangle C2AL:
- AL=r2cos(θ) since ∠C2AL=90∘−θ.
- From the above relationships, we can write:
AL=r1sin(θ)(1)
AL=r2cos(θ)(2)
- From trigonometric identities, we know:
sin2(θ)+cos2(θ)=1
- Substituting equations (1) and (2) into this identity gives:
(ALr1)2+(ALr2)2=1
- Rearranging gives:
AL2r21+AL2r22=1
- Factoring out AL2:
AL2(1r21+1r22)=1
- Thus, we can express AL2 as:
AL2=11r21+1r22=r21r22r21+r22
- Taking the square root gives:
AL=r1r2√r21+r22
- The length of the common chord AB is:
AB=2⋅AL=2⋅r1r2√r21+r22
The radius of the common chord is given by:
Radius of common chord=r1r2√r21+r22
Topper's Solved these Questions
- CO-ORDINATE GEOMETRY OF THREE DIMENSIONBOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - MISCELLANEOUS EXERCISE( FILL IN THE BLANKS)
16 Videos
- CO-ORDINATE GEOMETRY OF THREE DIMENSIONBOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - MATCHING ENTRIES (MATCH THE ENTERIES OF COLUMN-II WITH THOSE OF COLUMN-II UNDER THE FOLLOWING CONDITIONS : )
4 Videos
- CO-ORDINATE GEOMETRY OF THREE DIMENSIONBOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - PROBLEM SET (4)
19 Videos
- BINOMIAL THEOREM AND MATHEMATICAL INDUCTION BOOK - ML KHANNACHAPTER - BINOMIAL THEOREM AND MATHEMATICAL INDUCTION EXERCISE - Self Assessment Test
35 Videos
- COMPLEX NUMBERS BOOK - ML KHANNACHAPTER - COMPLEX NUMBERS EXERCISE - Assertion / Reason
2 Videos
Similar Questions
Knowledge Check
The circles having radii r1andr2 intersect orthogonally. The length of their common chord is 2r1r2√r12+r12 (b) √r12+r122r1r2 r1r2√r12+r12 (d) √r12+r12r1r2
A2r1r2√r21+r22Br1r2√r21+r22C2r21r2√r21+r22D2r22r1√r21+r22Two conducting spheres of radii r1 and r2 are equally charged. The ratio of their potentral is-
Ar21/r22Br22/r21Cr1/r2Dr2/r1Two metal spheres of radii R1 and R2 are charged to the same potential. The ratio of charges on the spheres is
A√R1:√R2BR1:R2CR21:R22DR31:R32
The circles having radii r 1 and r2 intersect orthogonally Length of their common chord is
View SolutionProve that if two bubbles of radii r1 and r2 coalesce isothermally in vacuum then the radius of new bubble will be r=√r21+r22
View SolutionTwo soap bubbles of radii r1andr2 are attached as shown. Find the radius of curvature of the common film ACB.
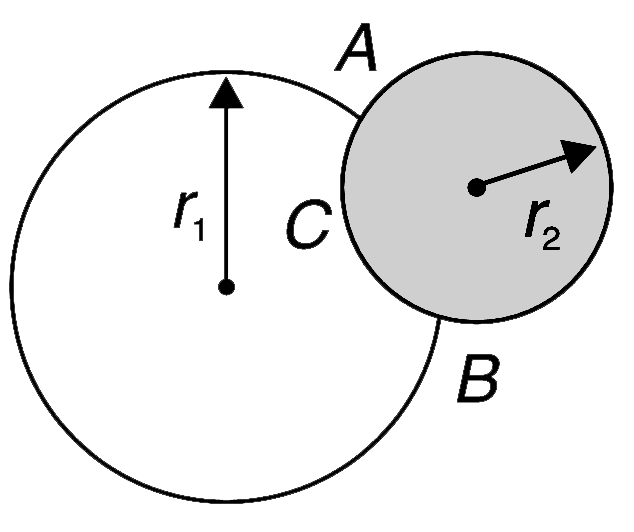
View SolutionTwo soap bubbles of radius r1 and r2 combine. Find radius of curvature of the common surface separating them.

View SolutionTwo charged spheres of radii R1andR2 having equal surface charge density. The ratio of their potential is
View Solution
