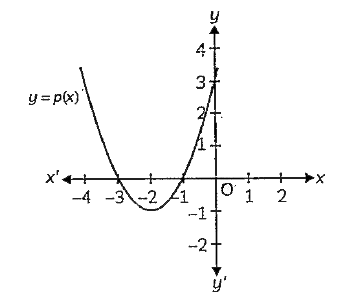
What are the zeroes of the polynomial (x2−9)?
(3, 0)
(3, -3)
(0, 3)
(3, 3)
The correct Answer is:B
To find the zeroes of the polynomial x2−9, we need to determine the values of x for which the polynomial equals zero.
1. Set the polynomial equal to zero:
x2−9=0
2. Rearrange the equation:
x2=9
3. Take the square root of both sides:
x=±√9
4. Calculate the square root:
x=±3
5. Write the zeroes:
The zeroes of the polynomial x2−9 are:
x=3andx=−3
Final Answer:
The zeroes of the polynomial x2−9 are 3 and −3.
x2−9=0
x2=9
x=±√9
x=±3
The zeroes of the polynomial x2−9 are:
x=3andx=−3
The zeroes of the polynomial x2−9 are 3 and −3.
Topper's Solved these Questions
- SAMPLE PAPER 7BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - Section - A
7 Videos
- SAMPLE PAPER 7BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - Section - B
4 Videos
- SAMPLE PAPER 6BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section -C
7 Videos
- SAMPLE QUESTION PAPERBOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE QUESTION PAPEREXERCISE - Section -C
5 Videos
Similar Questions
Knowledge Check
What are the zeroes of the polynomial
2x2+14x+20 ?A−5,−2B5, 2C−3,−2D3, 2The zeroes of the polynomial x2−7 are :
A√7,√7B7,−7C√7,−√7DNone of these.
Write the zeros of the polynomial x2−x−6 .
View SolutionFind the zeroes of the polynomial 6x2−3
View SolutionFind the zeroes of the polynomial 6x2−3.
View SolutionIf α and β are the zeroes of the polynomial x2+6x+9 then form a polynomial whose zeroes are −α and −β
View SolutionIf α and β are the zeros of the polynomial f(x)=x2+px+q, form a polynomial whose zeros are (α+β)2 and (α−β)2.
View SolutionFind zeroes of the polynomial x2+2x
View Solution